10.7 PROBLEMS
Question 10.12
For each of the following situations, decide whether Ali has increasing, constant, or diminishing marginal utility.
The more economics classes Ali takes, the more he enjoys the subject. And the more classes he takes, the easier each one gets, making him enjoy each additional class even more than the one before.
Ali likes loud music. In fact, according to him, “the louder, the better.” Each time he turns the volume up a notch, he adds 5 utils to his total utility.
Ali enjoys watching reruns of the old sitcom Corner Gas. He claims that these episodes are always funny, but he does admit that the more he sees an episode, the less funny it gets.
Ali loves toasted marshmallows. The more he eats, however, the fuller he gets and the less he enjoys each additional marshmallow. And there is a point at which he becomes satiated: beyond that point, more marshmallows actually make him feel worse rather than better.
Question 10.13
Use the concept of marginal utility to explain the following: Newspaper vending machines are designed so that once you have paid for one paper, you could take more than one paper at a time. But soft drink vending machines, once you have paid for one can of pop, dispense only one can of pop at a time.
Question 10.14
Brenda likes to have bagels and coffee for breakfast. The accompanying table shows Brenda’s total utility from various consumption bundles of bagels and coffee.
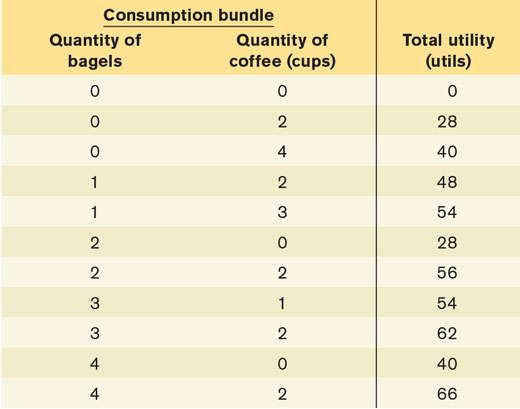
Suppose Brenda knows she will consume 2 cups of coffee for sure. However, she can choose to consume different quantities of bagels: she can choose either 0, 1, 2, 3, or 4 bagels.
Calculate Brenda’s marginal utility from bagels as she goes from consuming 0 bagel to 1 bagel, from 1 bagel to 2 bagels, from 2 bagels to 3 bagels, and from 3 bagels to 4 bagels.
Draw Brenda’s marginal utility curve of bagels. Does Brenda have increasing, diminishing, or constant marginal utility of bagels?
Question 10.15
Brenda, the consumer in Problem 3, now has to make a decision about how many bagels and how much coffee to have for breakfast. She has $8 of income to spend on bagels and coffee. Use the information given in the table in Problem 3 to answer the following questions.
Bagels cost $2 each, and coffee costs $2 per cup. Which bundles are on Brenda’s budget line? For each of these bundles, calculate the level of utility (in utils) that Brenda enjoys. Which bundle is her optimal bundle?
The price of bagels increases to $4, but the price of coffee remains at $2 per cup. Which bundles are now on Brenda’s budget line? For each bundle, calculate Brenda’s level of utility (in utils). Which bundle is her optimal bundle?
What do your answers to parts (a) and (b) imply about the slope of Brenda’s demand curve for bagels? Describe the substitution effect and the income effect of this increase in the price of bagels, assuming that bagels are a normal good.
Question 10.16
Bruno can spend his income on two different goods: Drake CDs and notebooks for his class notes. For each of the following three situations, decide if the given consumption bundle is within Bruno’s consumption possibilities. Then decide if it lies on the budget line or not.
CDs cost $10 each, and notebooks cost $2 each. Bruno has income of $60. He is considering a consumption bundle containing 3 CDs and 15 notebooks.
CDs cost $10 each, and notebooks cost $5 each. Bruno has income of $110. He is considering a consumption bundle containing 3 CDs and 10 notebooks.
CDs cost $20 each, and notebooks cost $10 each. Bruno has income of $50. He is considering a consumption bundle containing 2 CDs and 2 notebooks.
Question 10.17
Bruno, the consumer in Problem 5, is best friends with Bernie, who shares his love for notebooks and Drake CDs. The accompanying table shows Bernie’s utilities from notebooks and Drake CDs.
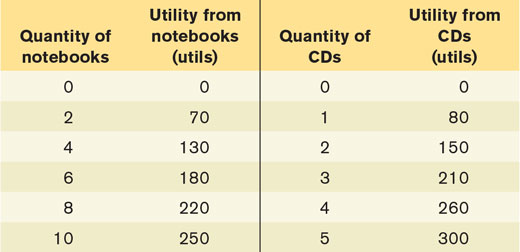
The price of a notebook is $5, the price of a CD is $10, and Bernie has $50 of income to spend.
Which consumption bundles of notebooks and CDs can Bernie consume if he spends all his income? Illustrate Bernie’s budget line with a diagram, putting notebooks on the horizontal axis and CDs on the vertical axis.
Calculate the marginal utility of each notebook and the marginal utility of each CD. Then calculate the marginal utility per dollar spent on notebooks and the marginal utility per dollar spent on CDs.
Draw a diagram like Figure 10-4 in which both the marginal utility per dollar spent on notebooks and the marginal utility per dollar spent on CDs are illustrated. Using this diagram and the utility-
maximizing principle of marginal analysis, predict which bundle— from all the bundles on his budget line— Bernie will choose.
Question 10.18
For each of the following situations, decide whether the bundle Lakshani is considering optimal or not. If it is not optimal, how could Lakshani improve her overall level of utility? That is, determine which good she should spend more on and which good should she spend less on.
Lakshani has $200 to spend on running shoes and sweaters. Running shoes cost $50 per pair, and sweaters cost $20 each. She is thinking about buying 2 pairs of running shoes and 5 sweaters. She tells her friend that the additional utility she would get from the second pair of running shoes is the same as the additional utility she would get from the fifth sweater.
Lakshani has $5 to spend on pens and pencils. Each pen costs $0.50 and each pencil costs $0.10. She is thinking about buying 6 pens and 20 pencils. The last pen would add five times as much to her total utility as the last pencil.
Lakshani has $50 per season to spend on tickets to football games and tickets to soccer games. Each football ticket costs $10 and each soccer ticket costs $5. She is thinking about buying 3 football tickets and 2 soccer tickets. Her marginal utility from the third football ticket is twice as much as her marginal utility from the second soccer ticket.
Question 10.19
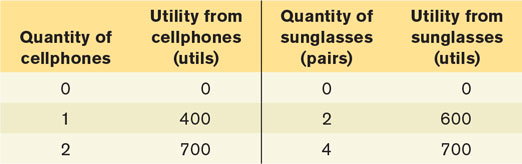
Cal “Cool” Cooper has $200 to spend on cellphones and sunglasses.
Each cellphone costs $100 and each pair of sunglasses costs $50. Which bundles lie on Cal’s budget line? Draw a diagram like Figure 10-4 in which both the marginal utility per dollar spent on cellphones and the marginal utility per dollar spent on sunglasses are illustrated. Use this diagram and the optimal consumption rule to decide how Cal should allocate his money. That is, from all the bundles on his budget line, which bundle will Cal choose? The accompanying table gives his utility of cellphones and sunglasses.
The price of cellphones falls to $50 each, but the price of sunglasses remains at $50 per pair. Which bundles lie on Cal’s budget line? Draw a diagram like Figure 10-4 in which both the marginal utility per dollar spent on cellphones and the marginal utility per dollar spent on sunglasses are illustrated. Use this diagram and the utility-
maximizing principle of marginal analysis to decide how Cal should allocate his money. That is, from all the bundles on his budget line, which bundle will Cal choose? The accompanying table gives his utility of cellphones and sunglasses. 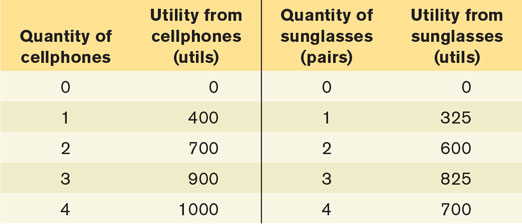
How does Cal’s consumption of cellphones change as the price of cellphones falls? In words, describe the income effect and the substitution effect of this fall in the price of cellphones, assuming that cellphones are a normal good.
Question 10.20
Damien Matthews is a busy actor. He allocates his free time to watching movies and working out at the gym. The accompanying table shows his utility from the number of times per week he watches a movie or goes to the gym.
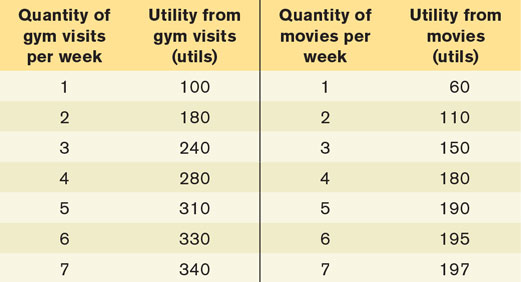
Damien has 14 hours per week to spend on watching movies and going to the gym. Each movie takes 2 hours and each gym visit takes 2 hours. (Hint: Damien’s free time is analogous to income he can spend. The hours needed for each activity are analogous to the price of that activity.)
Which bundles of gym visits and movies can Damien consume per week if he spends all his time either going to the gym or watching movies? Draw Damien’s budget line in a diagram with gym visits on the horizontal axis and movies on the vertical axis.
Calculate the marginal utility of each gym visit and the marginal utility of each movie. Then calculate the marginal utility per hour spent at the gym and the marginal utility per hour spent watching movies.
Draw a diagram like Figure 10-4 in which both the marginal utility per hour spent at the gym and the marginal utility per hour spent watching movies are illustrated. Use this diagram and the utility-
maximizing principle of marginal analysis to decide how Damien should allocate his time.
Question 10.21
Anna Jenniferson is an actress who currently spends several hours each week watching movies and going to the gym. On the set of a new movie she meets Damien, the consumer in Problem 9. She tells him that she likes watching movies much more than going to the gym. In fact, she says that if she had to give up seeing 1 movie, she would need to go to the gym twice to make up for the loss in utility from not seeing the movie. A movie takes 2 hours, and a gym visit also lasts 2 hours. Damien tells Anna that she is not watching enough movies. Is he right?
Question 10.22
Sven is a poor student who covers most of his dietary needs by eating cheap breakfast cereal, since it contains most of the important vitamins. As the price of cereal increases, he decides to buy even less of other foods and even more breakfast cereal to maintain his intake of important nutrients. This makes breakfast cereal a Giffen good for Sven. Describe in words the substitution effect and the income effect from this increase in the price of cereal. In which direction does each effect move, and why? What does this imply for the slope of Sven’s demand curve for cereal?
Question 10.23
In each of the following situations, describe the substitution effect and, if it is significant, the income effect. In which direction does each of these effects move? Why?
Ed spends a large portion of his income on his children’s education. Because tuition fees rise, one of his children has to withdraw from college.
Homer spends much of his monthly income on home mortgage payments. The interest on his adjustable-
rate mortgage falls, lowering his mortgage payments, and Homer decides to move to a larger house. Pam thinks that Spam is an inferior good. Yet as the price of Spam rises, she decides to buy less of it.
Question 10.24
Restaurant meals and housing (measured in the number of rooms) are the only two goods that Neha buys. She has income of $1000. Initially, she buys a consumption bundle such that she spends exactly half her income on restaurant meals and the other half of her income on housing. Then her income increases by 50%, but the price of restaurant meals increases by 100% (it doubles). The price of housing remains the same. After these changes, if she wanted to, could Neha still buy the same consumption bundle as before?
Question 10.25
Scott finds that the higher the price of orange juice, the more money he spends on orange juice. Does that mean that Scott has discovered a Giffen good?
Question 10.26
Margo’s marginal utility of one dance lesson is 100 utils per lesson. Her marginal utility of a new pair of dance shoes is 300 utils per pair. The price of a dance lesson is $50 per lesson. She currently spends all her income, and she buys her optimal consumption bundle. What is the price of a pair of dance shoes?
Question 10.27
According to Statistics Canada, the average retail price of a litre of regular gasoline in Regina, Saskatchewan rose from $0.547 in 1990 to $1.217 in 2011, a 122% increase.
Other things equal, describe the effect of this price increase on the quantity of gasoline demanded. In your explanation, make use of the utility-
maximizing principle of marginal analysis and describe income and substitution effects. In fact, however, other things were not equal. Over the same time period, the prices of other goods and services rose as well. According to Statistics Canada, the overall price of a bundle of goods and services consumed by an average consumer in Regina rose by 63% (from 1990 to 2011).
Taking into account the rise in the price of gasoline and in overall prices, other things equal, describe the effect on the quantity of gasoline demanded.
However, this is not the end of the story. Between 1990 and 2011, the typical consumer’s nominal income increased, too: Statistics Canada reports that in Saskatchewan, average household nominal after-
tax and transfer income rose from $30 300 in 1990 to $47 100 in 2011, an increase of 55%. Taking into account the rise in the price of gasoline, in overall prices, and in consumers’ incomes, describe the effect on the quantity of gasoline demanded.
Question 10.28
Sometimes, consumers might purchase goods not because of their particular functionality but rather for the social status associated with owning these goods. In economics, we call these goods positional goods as they serve as status symbols. Luxury homes, cars, jewellery, designer clothing, and other luxury goods and services can be considered positional goods.
Why would consumers purchase these goods? (Hint: Take luxury automobiles as an example. If you just want a means of transportation, then any car should do.)
Are the consumers of positional goods acting rationally or irrationally? Why is that so?