11.1 The Production Function
A production function is the relationship between the quantity of inputs a firm uses and the quantity of output it produces, for a given state of the production technology.
Afirm is an organization that produces goods or services for sale. To do this, it must transform inputs into output. The quantity of output a firm produces depends on the quantity of inputs and the state or generosity of the production technology; this relationship is known as the firm’s production function. As we’ll see, a firm’s production function underlies its cost curves. As a first step, let’s look at the characteristics of a hypothetical production function.
Inputs and Output
To understand the concept of a production function, let’s consider a farm that we assume, for the sake of simplicity, produces only one output, wheat, and uses only two inputs, land and labour. This particular farm is owned by a couple named Alec and Janet. They hire workers to do the actual physical labour on the farm. Moreover, we will assume that all potential workers are of the same quality—
A fixed input is an input whose quantity is fixed for a period of time and cannot be varied.
Alec and Janet’s farm sits on 1 hectare of land; no more land is available to them, and they are currently unable to either increase or decrease the size of their farm by selling, buying, or leasing land. Land here is what economists call a fixed input—an input whose quantity is fixed for a period of time and cannot be varied. Alec and Janet are, however, free to decide how many workers to hire. The labour provided by these workers is called a variable input—an input whose quantity the firm can vary at any time.
A variable input is an input whose quantity the firm can vary at any time.
The long run is the time period in which all inputs can be varied.
In reality, whether or not the quantity of an input is really fixed depends on the time horizon. In the long run—that is, given that a long enough period of time has elapsed—
The short run is the time period in which at least one input is fixed.
The total product curve shows how the quantity of output depends on the quantity of the variable input employed, for a given quantity of the fixed input and production technology.
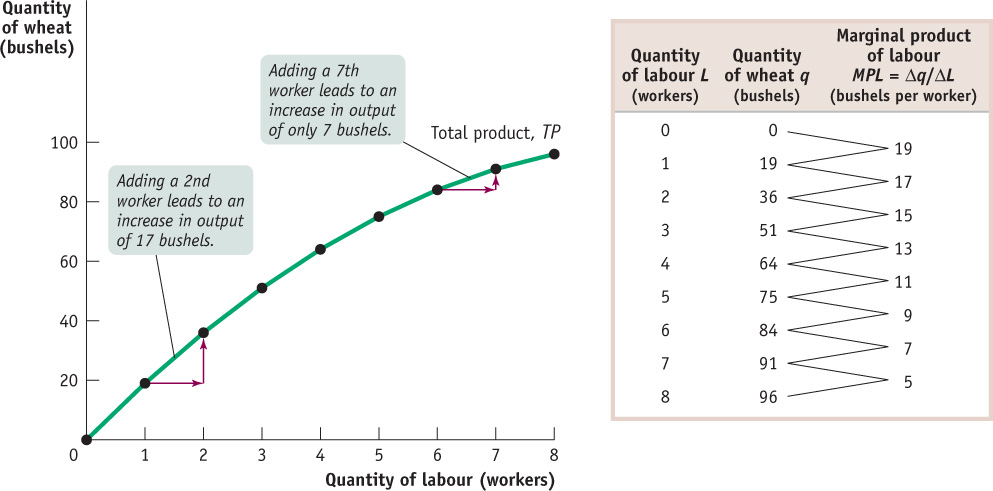
Alec and Janet know that the quantity of wheat they produce depends on the number of workers they hire. Using modern farming techniques, one worker can cultivate the 1 hectare farm, albeit not very intensively. When an additional worker is added, the land is divided equally among all the workers: each worker has ½ of a hectare to cultivate when 2 workers are employed, each cultivates 1/3 of a hectare when 3 are employed, and so on. So as additional workers are employed, the land is cultivated more intensively and more bushels of wheat are produced. The relationship between the quantity of labour and the quantity of output, for a given amount of the fixed input and the given production technology (i.e., farming techniques used in this example), constitutes the farm’s production function. The production function for Alec and Janet’s farm, where land is the fixed input and labour is a variable input, is shown in the first two columns of the table in Figure 11-1; the diagram there shows the same information graphically. The curve in Figure 11-1 shows how the quantity of output depends on the quantity of the variable input, for a given quantity of the fixed input and the given production technology; it is called the farm’s total product curve. The physical quantity of output, bushels of wheat, is measured on the vertical axis; the quantity of the variable input, labour (that is, the number of workers employed), is measured on the horizontal axis. The total product curve here slopes upward, reflecting the fact that more bushels of wheat are produced as more workers are employed.
The marginal product of an input is the additional quantity of output that is produced by using one more unit of that input, holding other inputs and the production technology fixed.
Although the total product curve in Figure 11-1 slopes upward along its entire length, the slope isn’t constant: as you move up the curve to the right, it flattens out. To understand why the slope changes, look at the third column of the table in Figure 11-1, which shows the change in the quantity of output that is generated by adding one more worker. This is called the marginal product of labour, or MPL: the additional quantity of output from using one more unit of labour (where one unit of labour is equal to one worker), holding other inputs and the production technology fixed. In general, the marginal product of an input is the additional quantity of output that is produced by using one more unit of that input.
In this example, we have data on changes in output at intervals of 1 worker. Sometimes data aren’t available in increments of 1 unit—

or
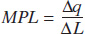
In this equation, Δ, the Greek uppercase delta, represents the change in a variable. Here, the lowercase q represents the quantity of output made by an individual firm, as opposed to an uppercase Q, which represents the quantity of output in the market as a whole.
WHEAT YIELDS AROUND THE WORLD
Wheat yields differ substantially around the world. The disparity between France and Canada that you see in this graph is particularly striking, given that they are both wealthy countries with comparable agricultural technology. Yet the reason for that disparity is straight-
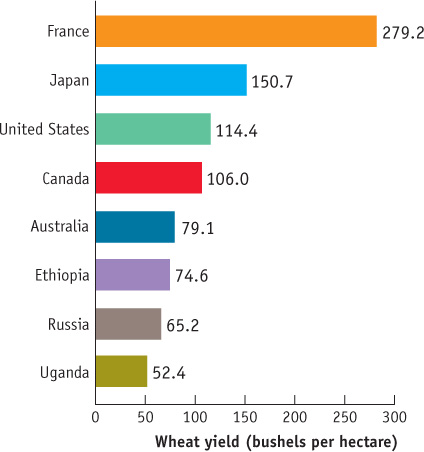
Source: Food and Agriculture Organization of the United Nations. Data are from 2012.
Now we can explain the significance of the slope of the total product curve: it is equal to the marginal product of labour. The slope of a line is equal to “rise” over “run” (see Appendix 2A). This implies that the slope of the total product curve is the change in the quantity of output (the “rise”, Δq) divided by the change in the quantity of labour (the “run”, ΔL). And this, as we can see from Equation 11-1, is simply the marginal product of labour. So in Figure 11-1, the fact that the marginal product of the first worker is 19 also means that the slope of the total product curve in going from 0 to 1 worker is 19. Similarly, the slope of the total product curve in going from 1 to 2 workers is the same as the marginal product of the second worker, 17, and so on.
In this example, the marginal product of labour declines as more workers are hired—
Figure 11-2 shows how the marginal product of labour depends on the number of workers employed on the farm. The marginal product of labour, MPL, is measured on the vertical axis in units of physical output—
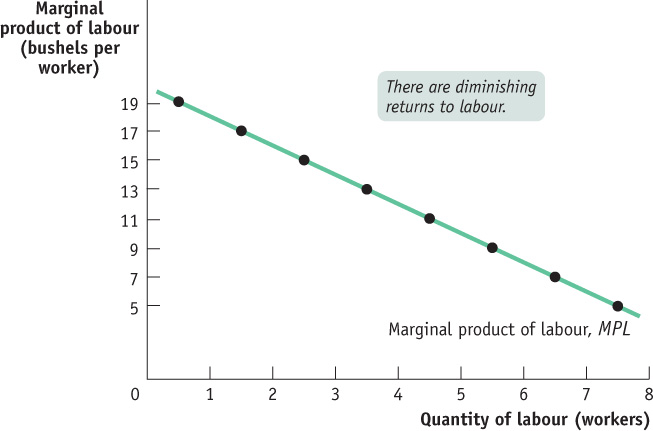
There are diminishing returns to an input when an increase in the quantity of that input, holding the levels of all other inputs and the production technology fixed, leads to a decline in the marginal product of that input.
In this example the marginal product of labour falls as the number of workers increases. That is, there are diminishing returns to labour on Alec and Janet’s farm. In general, there are diminishing returns to an input when an increase in the quantity of that input, holding the quantity of all other inputs and the production technology fixed, reduces that input’s marginal product. Due to diminishing returns to labour, the MPL curve is negatively sloped.
To grasp why diminishing returns can occur, think about what happens as Alec and Janet add more and more workers without increasing the amount of land or improving their farming techniques. As the number of workers increases, the land is farmed more intensively and the number of bushels produced increases. But each additional worker is working with a smaller share of the 1 hectare—
The crucial point to emphasize about diminishing returns is that, like many propositions in economics, it is an “other things equal” proposition: each successive unit of an input will raise production by less than the last if the quantity of all other inputs is and the production technology are held fixed.
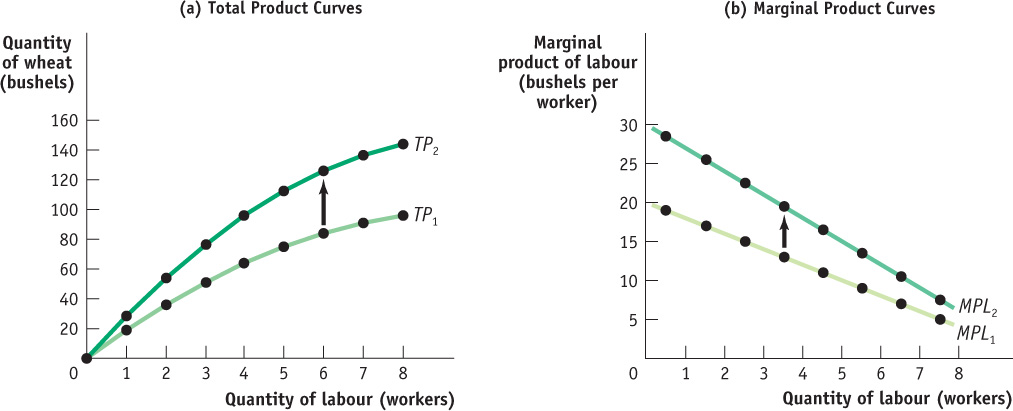
What would happen if the levels of other inputs or production techniques were allowed to change? You can see the answer illustrated in Figure 11-3. Panel (a) shows two total product curves, TP1 and TP2. TP1 is the farm’s total product curve when its total area is 1 hectare (the same curve as in Figure 11-1). TP2 is the total product curve when the farm has increased to 2 hectares, holding farming techniques fixed. Except when 0 workers are employed, TP2 lies above TP1 everywhere because with more land available, any given number of workers produces more output. Panel (b) shows the corresponding marginal product of labour curves. MPL1 is the marginal product of labour curve given 1 hectare to cultivate (the same curve as in Figure 11-2), and MPL2 is the marginal product of labour curve given 2 hectares. Both curves slope downward because, in each case, the amount of land is fixed, albeit at different levels. But MPL2 lies above MPL1 everywhere, reflecting the fact that the marginal product of the same worker is higher when he or she has more of the fixed input to work with.
WHAT’S A UNIT?
The marginal product of labour (or any other input) is defined as the increase in the quantity of output when you increase the quantity of that input by one unit, holding all other inputs and the production technology fixed. But what do we mean by a “unit” of labour? Is it an additional hour of labour, an additional week, or a person-
The answer is that it doesn’t matter, as long as you are consistent. One common source of error in economics is getting units confused—
Figure 11-3 demonstrates a general result: the position of the total product curve of a given input depends on the quantities of other inputs. If you change the quantity of the other inputs, both the total product curve and the marginal product curve of the remaining input will shift. Similarly, if there is an improvement in farming techniques (an example of an improvement in production technology), both the total product curve and the marginal product of labour curve would shift upward. With improved farming techniques, farmers can produce more bushels of wheat for any given amount of land.
The Average Product of Labour
Alec and Janet may also be interested in knowing how much wheat is harvested per worker. The average product of labour, or APL, is the amount of output produced per unit of labour. In general, the average product of an input is the amount of output produced per unit of that input employed in the production process:
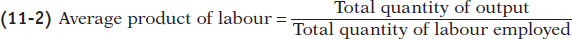
or
The average product of an input is the amount of output produced per unit of that input.
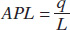
The average product of labour is important because it tells the producer how many units of output the average or typical unit of labour can produce. Recall, the marginal product of labour shows the change in the quantity of output that is generated by adding one more worker. Although these quantities sound similar, they are typically not the same and confusion between them is a common source of errors in economics. Figure 11-4 shows the average product of labour curve for Alec and Janet’s farm compared to the marginal product of labour.
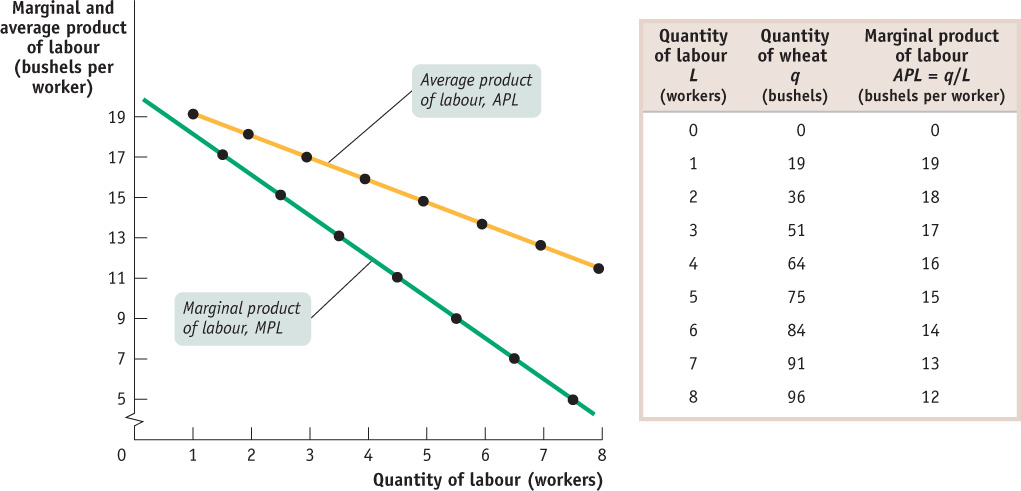
As you can see from the table in Figure 11-4, all other things equal, as the number of workers employed increases, output rises but the average product of labour falls. For example, when the number of workers employed increases from 4 to 5, output increases from 64 bushels to 75 bushels. However, the average product of labour falls from 16 bushels to 15 bushels. Why does this happen? It is because the marginal product is less than the average product; thus, the average product is decreasing. When the number of workers rises from 4 to 5, the marginal product of the fifth worker is 11 bushels (the table in Figure 11-1 shows the marginal product labour). The marginal contribution of that additional worker is below the average productivity of the first 4 workers, so the fifth worker lowers the average product of the whole group. On the farm, the marginal product of labour is less than the average product of labour for all quantities of labour, so the entire average product curve slopes downward.
Our example shows that both marginal product and average product are downward sloping. In practice, however, economists believe that both the marginal product and average product curves often slope upward as a firm increases its variable input from some low level (such as zero), sloping downward only at higher levels of this input: so they look like inverted U-
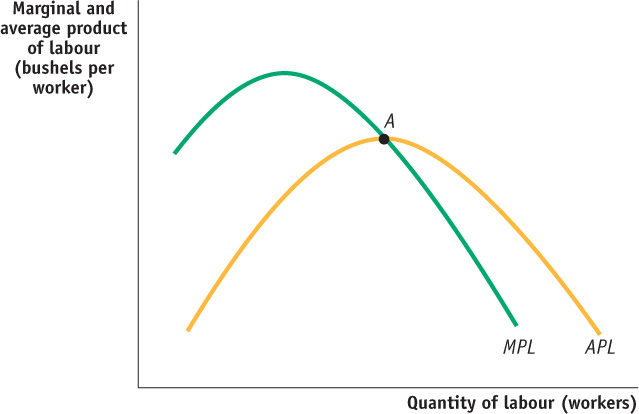
At a low level of the variable input, the marginal product is increasing because when the firm starts with a low number of workers. Adding more workers in the production process allows these workers to specialize in various tasks. Specialization can allow workers to become more productive, which raises their marginal product. For example, one individual producing salsa would have to perform all the tasks involved: selecting and preparing the ingredients, mixing the salsa, bottling and labelling it, packing it into cases, and so on. As more workers are employed, they can divide the tasks, with each worker specializing in one or a few aspects of salsa-
By looking at Figure 11-5, you can also observe the following key facts about the relationship between marginal product and average product:
When marginal product is greater than average product, average product rises.
When marginal product is less than average product, average product falls.
When marginal product is the same as average product, average product remains unchanged. Indeed, the average product is maximized when the marginal product is equal to the average product.
As a result, when the marginal product curve has an inverted U-
From the Production Function to Cost Curves
Once Alec and Janet know their production function, they know the relationship between inputs of labour and land and output of wheat for a given level of farming technique (or technology). But if they want to maximize their profits, they need to translate this knowledge into information about the relationship between the quantity of output and cost. Let’s see how they can do this.
To translate information about a firm’s production function into information about its costs, we need to know how much the firm must pay for its inputs. We will assume that Alec and Janet face either an explicit or an implicit cost of $400 for the use of the land. As we learned in Chapter 9, it is irrelevant whether Alec and Janet must rent the land for $400 from someone else or whether they own the land themselves and forgo earning $400 from renting it to someone else. Either way, they pay an opportunity cost of $400 by using the land to grow wheat. Moreover, since the land is a fixed input, the $400 Alec and Janet pay for it is a fixed cost, denoted by FC—a cost that does not depend on the quantity of output produced (in the short run). In business, fixed cost is often referred to as “overhead cost.”
A fixed cost is a cost that does not depend on the quantity of output produced. It is the cost of the fixed input.
We also assume that Alec and Janet must pay each worker $200. Using their production function, Alec and Janet know that the number of workers they must hire depends on the amount of wheat they intend to produce. So the cost of labour, which is equal to the number of workers multiplied by $200, is a variable cost, denoted by VC—a cost that depends on the quantity of output produced. Adding the fixed cost and the variable cost of a given quantity of output gives the total cost, or TC, of that quantity of output. We can express the relationship among fixed cost, variable cost, and total cost as an equation:
A variable cost is a cost that depends on the quantity of output produced. It is the cost of the least amount of the variable input needed to make the quantity of output.
The total cost of producing a given quantity of output is the sum of the fixed cost and the variable cost of producing that quantity of output.
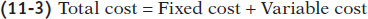
or
TC = FC + VC
The table in Figure 11-6 shows how total cost is calculated for Alec and Janet’s farm. The second column shows the number of workers employed, L. The third column shows the corresponding level of output, q, taken from the table in Figure 11-1. The fourth column shows the variable cost, VC, equal to the number of workers multiplied by $200. The fifth column shows the fixed cost, FC, which is $400 regardless of how many workers are employed. The sixth column shows the total cost of output, TC, which is the variable cost plus the fixed cost.
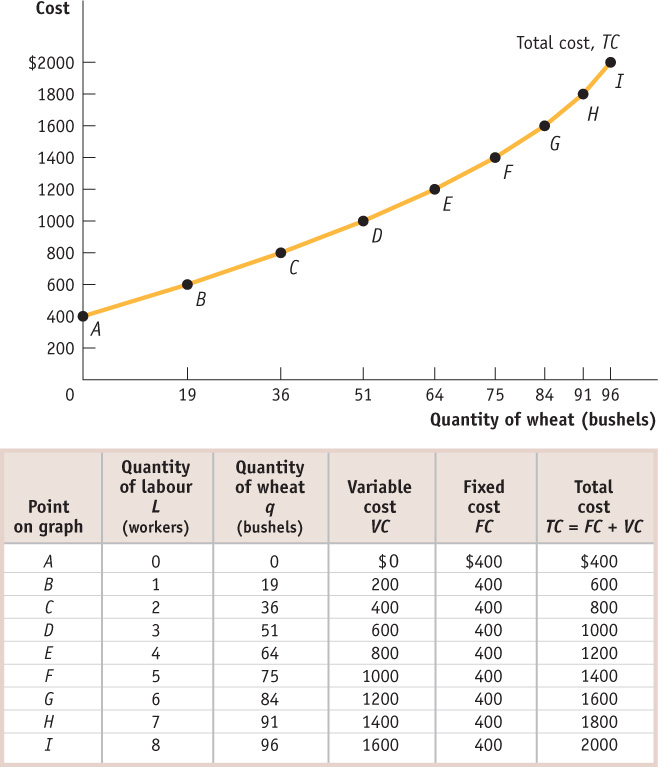
The first column labels each row of the table with a letter, from A to I. These labels will be helpful in understanding our next step: drawing the total cost curve, a curve that shows how total cost depends on the quantity of output.
The total cost curve shows how total cost depends on the quantity of output.
Alec and Janet’s total cost curve is shown in the diagram in Figure 11-6, where the horizontal axis measures the quantity of output in bushels of wheat and the vertical axis measures total cost in dollars. Each point on the curve corresponds to one row of the table in Figure 11-6. For example, point A shows the situation when 0 workers are employed: output is 0, and total cost is equal to fixed cost, $400. Similarly, point B shows the situation when 1 worker is employed: output is 19 bushels, and total cost is $600, equal to the sum of $400 in fixed cost and $200 in variable cost.
Like the total product curve, the total cost curve slopes upward: due to the variable cost, the more output produced, the higher the farm’s total cost. But unlike the total product curve, which gets flatter as employment rises, the total cost curve gets steeper. That is, the slope of the total cost curve is greater as the amount of output produced increases. As we will soon see, the steepening of the total cost curve is also due to diminishing returns to the variable input. Before we can understand this, we must first look at the relationships among several useful measures of cost.
THE MYTHICAL MAN-MONTH
The concept of diminishing returns to an input was first formulated by economists during the late eighteenth century. These economists, notably including Thomas Malthus, drew their inspiration from agricultural examples. Although still valid, examples drawn exclusively from agriculture can seem somewhat old-fashioned in our modern economy.
However, the idea of diminishing returns to an input applies with equal force to the most modern of economic activities—such as, say, the design of software. In 1975 Frederick P. Brooks Jr., a project manager at IBM during the days when it dominated the computer business, published a book titled The Mythical Man-Month that soon became a classic—so much so that a special anniversary edition was published 20 years later.
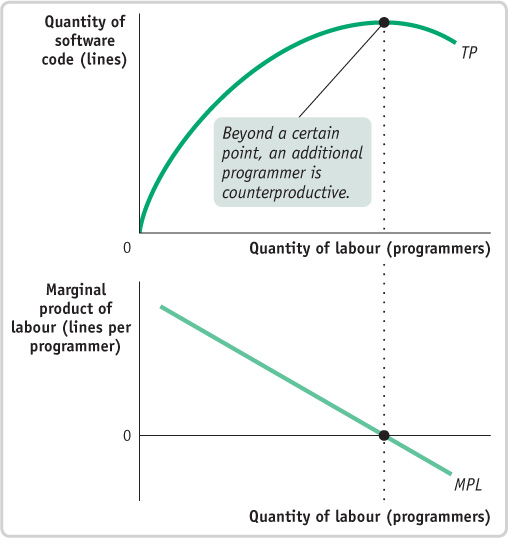
The chapter that gave its title to the book is basically about diminishing returns to labour in the writing of software. Brooks observed that multiplying the number of programmers assigned to a project did not produce a proportionate reduction in the time it took to get the program written. A project that could be done by 1 programmer in 12 months could not be done by 12 programmers in 1 month— hence the “mythical man-month,” the false notion that the number of lines of programming code produced was proportional to the number of code writers employed. In fact, above a certain number, adding another programmer on a project actually increased the time to completion.
The argument of The Mythical Man-Month is summarized in Figure 11-7. The upper part of the figure shows how the quantity of the project’s output, as measured by the number of lines of code produced per month, varies with the number of programmers. Each additional programmer accomplishes less than the previous one, and beyond a certain point an additional programmer is actually counterproductive. The lower part of the figure shows the marginal product of each successive programmer, which falls as more programmers are employed and eventually becomes negative. In other words, programming is subject to diminishing returns so severe that at some point more programmers actually have negative marginal product. The source of the diminishing returns lies in the nature of the production function for a programming project: each programmer must coordinate his or her work with that of all the other programmers on the project, leading to each person spending more and more time communicating with others as the number of programmers increases. In other words, other things equal, there are diminishing returns to labour. It is likely, however, that if fixed inputs devoted to programming projects are increased—say, installing a faster Wiki system—the problem of diminishing returns for additional programmers can be mitigated.
A reviewer of the reissued edition of The Mythical Man-Month summarized the reasons for these diminishing returns: “There is an inescapable overhead to yoking up programmers in parallel. The members of the team must ‘waste time’ attending meetings, drafting project plans, exchanging e-mail, negotiating interfaces, enduring performance reviews, and so on. … At Microsoft, there will be at least one team member that just designs T-shirts for the rest of the team to wear.”

Quick Review
The firm’s production function is the relationship between quantity of inputs and output for a given state of production technology. The total product curve shows how the quantity of output depends on the quantity of the variable input for a given quantity of the fixed input and production technique, and its slope is equal to the marginal product of the variable input. In the short run, the fixed input cannot be varied; in the long run all inputs are variable.
When the levels of all other inputs and production technique are fixed, diminishing returns to an input may arise, yielding a downward-sloping marginal product curve and a total product curve that becomes flatter as more output is produced.
The average product of an input is the amount of output produced per unit of that input.
The total cost of a given quantity of output equals the fixed cost plus the variable cost of that output. The total cost curve becomes steeper as more output is produced due to diminishing returns to the variable input.
Check Your Understanding 11-1
CHECK YOUR UNDERSTANDING 11-1
Question 11.1
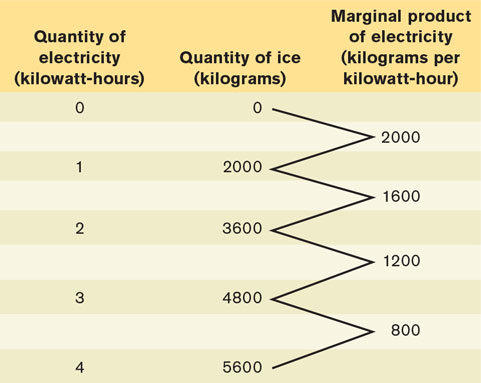
Bernie’s ice-making company produces ice cubes using a 10-tonne machine and electricity. The quantity of output, measured in terms of kilograms of ice, is given in the accompanying table.
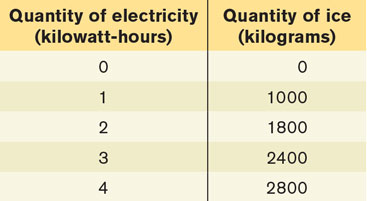
What is the fixed input? What is the variable input?
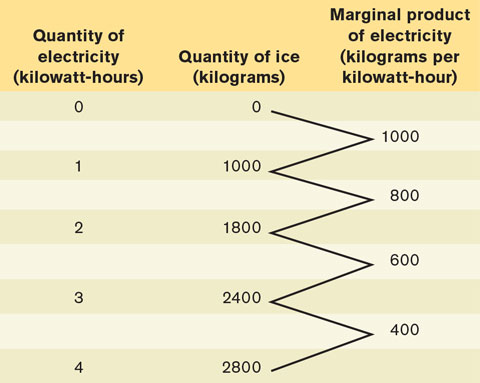
Construct a table showing the marginal product of the variable input. Does it show diminishing returns?
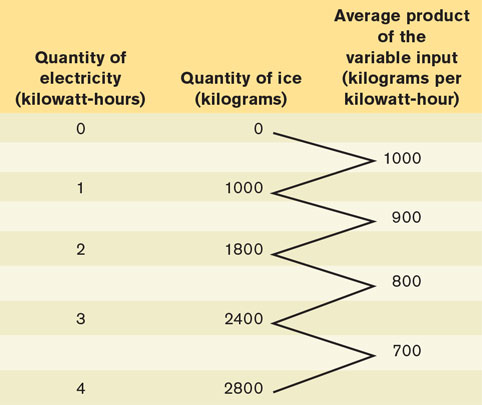
Construct a table showing the average product of the variable input. What happens to the average product as the quantity of variable input used increases?
Suppose a 50% increase in the size of the fixed input increases output by 100% for any given amount of the variable input. What is the fixed input now? Construct a table showing the quantity of output, marginal product, and average product in this case.
The fixed input is the 10-tonne machine, and the variable input is electricity.
As you can see from the declining numbers in the third column of the accompanying table, electricity does indeed exhibit diminishing returns: the marginal product of each additional kilowatt-hour of electricity is less than that of the previous kilowatt-hour.
As you can see from the accompanying table, the average product of the variable input falls as the quantity of variable input used increases.
A 50% increase in the size of the fixed input means that Bernie now has a 15-tonne machine. So the fixed input is now the 15-tonne machine. Since it generates a 100% increase in output for any given amount of electricity, the quantity of output and marginal product are now as shown in the accompanying table.