Chapter 12. Corn Genetics
Objectives
By the end of the period, students will be able to:
- determine the crosses of corn plants that produced phenotypic ratios observed in lab.
- learn to do a Chi-square test.
- continue working genetics problems.
Introduction
With many genetics problems, you will be given genotype, phenotype, and possibly which genes are dominant and which are recessive. However, in most life situations, one may not know ahead of time the genotype, phenotype, or pattern of inheritance. Additionally, there are frequently challenges in scoring individuals for a particular character, as there can be a lot of individual variability.
In lab today we will use corn as our model organism.
Each lab will have several ears of corn, where each kernel is a fertilization event. Working in pairs you will count kernels and determine the cross that produced that seed. In some cases the crosses are a simple monohybrid cross; in other cases they are a dihybrid cross.
Your task is to deduce the phenotype and genotype of the parents for the ears of corn at your table. Remember to handle the ears carefully!!
Here are the steps:
- Look at the ears of corn and decide on how many different phenotypes are present. There may be differences in color (purple or yellow) and there may be differences in shape (starchy or sweet).
- Count and record the number of each different phenotype.
- Look at your data and make a hypothesis about what the parental genotypes could be.
- For your hypothesized parental genotypes, make a prediction about what the ratio of phenotypes in the offspring will be. From the ratio, calculate the expected number for each phenotype given the number of kernels. (For example, if there were 200 kernels and you expected a 3:1 ratio in the phenotype, you would expect the ratio of actual numbers would be 150:50.)
- Do a Chi-square analysis. The formula for the Chi-square is on the inside front cover of your book and your TA will explain the steps. In a Chi-square analysis you are calculating a measure of how different your observed numbers are from your expected numbers given a particular hypothesis. If your Chi-square is a large number, the differences between your observed and expected values are large and your hypothesis is not supported. If you calculate a small value for the Chi-square, the difference between your observed and expected values is small and your hypothesis is supported. You will need to compare your Chi-square value to the table provided in class. You know where to look in the table by first knowing your degrees of freedom (df). In this test, degrees of freedom is the number of phenotypic classes –1. Once you know your df, read across the row and see where your calculated Chi-square value falls. Finally, look across the top row to determine the probability that chance alone could cause the difference between observed and expected. Scientists often take a probability of 0.05 as a cutoff point for statistical significance.
- Complete a Chi-square test for the ear of corn your group is assigned. As time permits, do the same for other ears as well. Show your work. Remember that a Chi-square is always testing a specific hypothesis. Be sure to restate your hypothesis.
Example
Let’s say you have an ear of corn that has some yellow kernels and some purple kernels. You count them and find that there are 85 purple and 15 yellow kernels. You know that they all came from one set of parents. What cross could result in these numbers? Your first hypothesis may be that two heterozygotes were crossed. If that hypothesis was correct you would predict a 3:1 phenotypic ratio, or for 100 individuals, you would predict a 75:25 ratio.
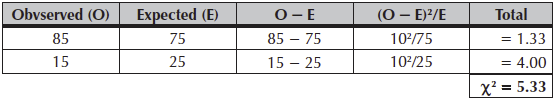
You then compare the calculated X2 with the X2 table to see if this is significant.
Degrees of freedom in this situation is the number of phenotypic classes minus 1 or
For 1 degree of freedom, the cutoff point for significance is 3.84. Our number is 5.33, which is bigger than 3.84, and so the differences are significant. That is, there is a low probability (<0.05) that chance alone would cause the differences in the observed and expected values, so something else must be causing the difference.
We reject our hypothesis.
How do you calculate the expected numbers if you don’t have 100 individuals?
Let’s say you have 250 individuals and you expect a 3:1 ratio.
In this case, 3/4 of 250 = 187.5 are expected to be purple and 62.5 or 250/4 are expected to be yellow.
Note: For each ear of corn, provide a count of each phenotype and suggest what parental genotype could account for what you see. You must complete a Chi-square test for two of the ears, a monohybrid cross and a dihybrid cross. We will have one group for each ear come up and present their Chi-square for the cross.