Chapter 1.

Overview

Generality

It is easy to see the physical differences between solid water (ice), liquid water, and gaseous water (steam). The solid exists at low temperatures and takes a shape that is essentially independent of the container it is place in, the gas exists at high temperatures and entirely fills whatever volume it is placed in, and the liquid is in-between (it takes the shape of its container but it does not generally fill the entire container). It is fortunate that this kind of simple model of three-phases is what we find in most pure substances.

This model includes not only the three phases but also the changes of phase that we see and will be important in our understanding of thermal and bond energies.

Focus

We will generally use this model to understand physical systems to which we are adding or removing heat to change the equilibrium state. Our focus with this model is on how the temperature changes when heat is added to a pure substance. When the phase is not changing then adding heat changes the temperature. Conversely, adding heat during a change in phase does not change the temperature.

Model Summary

Graphical Representation

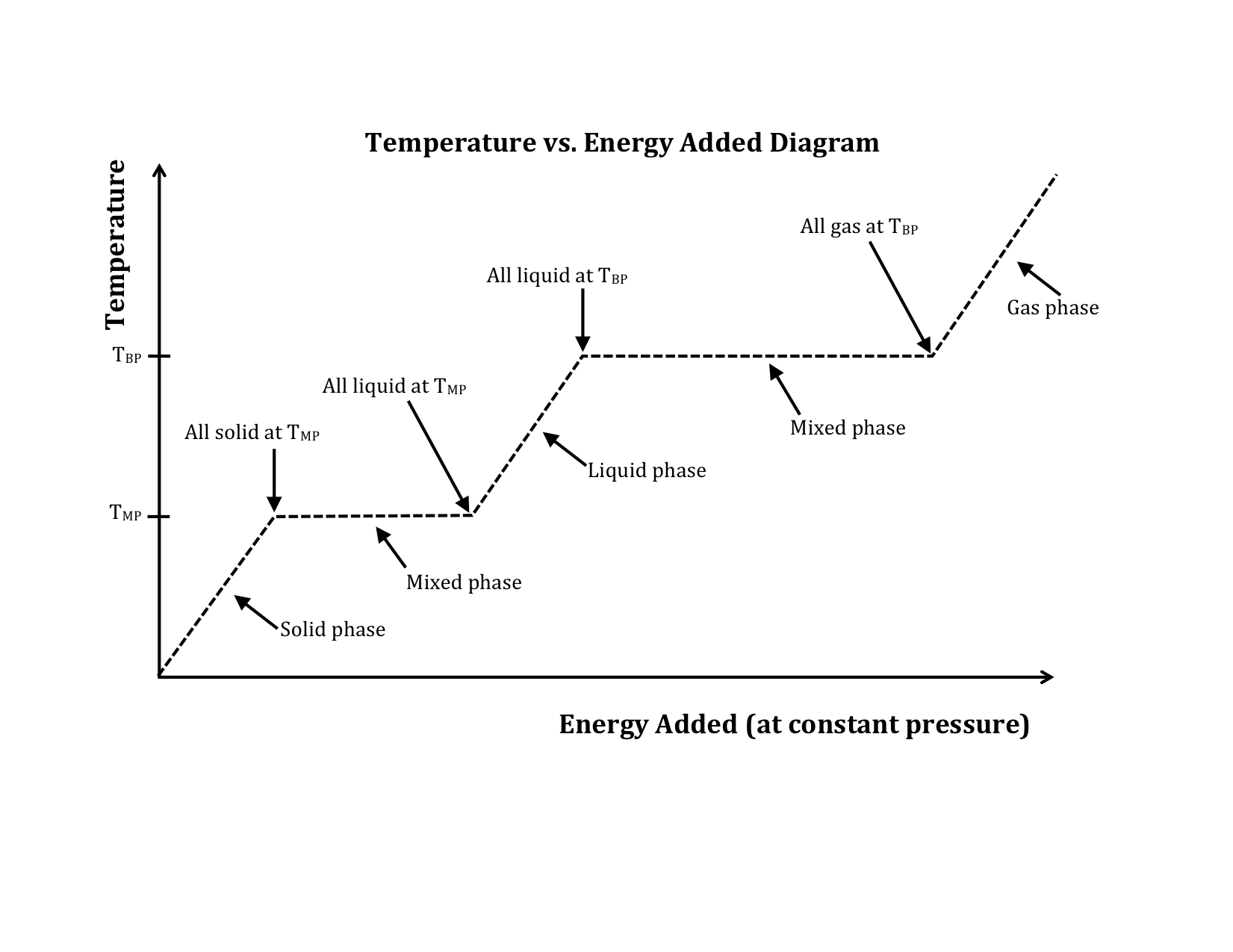
Algebraic Representations

Change in temperature of a substance when heat is added or removed:

Amount of a substance that changes phase when heat is added or removed:

Elaboration

Pure substances, Three phases, Solid, Liquid, Gas

Pure substances exist in one of three phases, depending on the temperature: solid, liquid, or gas. The statement above is the simplest statement that can be made regarding ordinary matter at one atmosphere of pressure. It captures the behavior of the vast majority of pure substances. Mixtures or composites, which are composed of more than one kind of pure substance will often behave differently as the temperature changes, because one component can begin to change phase or even decompose chemically, before the other component reaches the next phase change temperature.

The following information in this paragraph is interesting to know, but is not important in terms of the understanding the model. The common exception to the statement that all substances exist in all three phases is CO₂, which does not exist as a liquid at a pressure of one atmosphere, but does exist as a liquid at higher pressures. Solid carbon dioxide passes directly from the solid phase to the gas phase at its sublimation temperature when it is at one atmosphere of pressure. Another exception is the common isotope of the element helium, which does not exist as a solid, even if it is cooled to as close to the absolute zero of temperature as we wish to define zero. At one atmosphere it remains a liquid, albeit, a very strange liquid, called a superfluid, all the way down to absolute zero.

Temperature, Energy, Energy added as Heat or Work, Change of phase,

In order to change either the temperature or phase of a substance, energy must be added or removed. Often this energy is transferred to or from the substance as heat, Q, but can also be transferred as work, W. The first relationship, discussed above, could be interpreted simply as a common data pattern that is observed for pure substances. The model part really comes into its own with this second and the following third and fourth relationships. This second relationship “establishes a reason” for why substances don’t just willy-nilly change their temperature or change their phase. Neither can happen unless energy is added or removed.

Change of phase, Phase change temperature, Thermal equilibrium, Mixed phase

Changes of phase (solid↔liquid and liquid↔gas or at some values of pressure, solid↔gas) occur at specific temperatures, the phase change temperatures (T_MP, T_BP, and T_SP), which have particular values for each pure substance. The values of these temperatures are the same “going through” the phase change in “both directions.” Phase change temperatures are, however, dependent on the pressure. The amount of energy added or removed at a phase change (usually written as ΔH to signify constant pressure) is unique to each substance and has been measured for most substances.

If the substance is in thermal equilibrium (i.e., if all of the substance is at the same temperature) at the phase change temperature, both phases will remain at the phase change temperature as the phase change occurs. Mixed phases can exist in thermal equilibrium only when the temperature has the value of the phase-change temperature. There is a lot of meaning packed into the three parts of this third relationship. There is something unique about each kind of substance that determines how much energy is required to cause the substance to change phase. Also, in this simple model, the amount of change in energy is the same (except for the algebraic sign) whether you go through the phase transition by adding energy or by removing energy. Finally, there is a lot of meaning packed into the last part of the statement regarding when and under exactly what circumstances two phases can co-exist without one turning into the other. Think about your cold soft drink about half filled with ice and half filled with cola. If there is sufficient ice when the warmer cola is put in, and if the drink is in a well-insulated container, some ice does melt, but then the cola and ice seem to peacefully co-exist for quite some time. According to this relationship, this can only happen if what condition holds? Only if both phases are at the phase-change temperature. Additionally, this last part of the relationship tells us what must happen if energy is added sufficiently slowly so that the system is nearly in thermal equilibrium. What happens to the temperature when energy is slowly added to the mixed phase? The temperature remains at the phase change temperature until all of the substance has changed phase. When you seriously think about it, this is kind of weird. Normally, when we heat something, the temperature rises. But in this special case of mixed phases, the temperature does not rise. There is much predictive power in this last relationship.

Heat capacity, Specific heat

Changes of temperature of a substance occur when energy is added or removed whenever the substance is not at a phase-change temperature. This last relationship tells us what we are already familiar with. When you add energy, the temperature of the substance goes up. When the energy added is in the form of heat, the change in temperature, ΔT, is related to the amount of energy added by a property of the substance called heat capacity, C, that has a particular value for each substance. The specific heat capacity (or just “specific heat”) is the heat capacity divided by the amount of the specific substance (usually one divides by either the mass, in kg, or the number of moles of the substance). Specific heat capacities have been measured for most substances.

The graphical representation shown on the Model Summary compactly summarizes all of the relationships of the model. It is very easy to be lulled into thinking this all makes sense, however, without actually digging into the meaning. You need to ask questions like, what exactly is the meaning of the horizontal portions of the graph. Can you explain in you own words “what is going on” physically in your ice cola drink during a horizontal portion of the graph? Which horizontal portion would correspond to your cold drink? What about the three slanted portions of the graph? What exactly is happening there? Where did the ice start out when you first put it into your cup? When was your drink system in thermal equilibrium, or was it ever in thermal equilibrium?

Can you picture what is happening in terms of this representation when you boil water to make tea? How is this different from putting ice trays into the freezer compartment of your refrigerator? How do the algebraic relationships relate to the graph? Which parts? What is the relationship? These are the kinds of questions you need to be asking yourself and getting confident about. You want to practice using this representation enough so that it really does become a useful tool to make sense of thermal phenomena and to be comfortable using it to construct explanations for particular phenomena.