Chapter 6.

Overview

Generality

The very simple particle model of thermal energy developed here predicts the value of the thermal energy as a function of temperature for practically all substances. It relates the macroscopic variables of thermal energy and heat capacity to the energies in the “jiggling” microscopic particles of any substance when they are in equilibrium at some temperature. It provides a precise connection between temperature and the microscopic energies involved in the random jiggling of the particles.

Focus

Our focus with this introductory particle model of thermal energy is on developing a solid understanding of several new constructs, particularly the construct of “mode” as a “way of having energy,” that will allow us to make sense of thermal energy, heat capacity, and temperature and how they are related to the atoms and molecules that make up substances.

Model Summary

Algebraic Representations

Relationship (6) E_thermal/mode = ½k_BT

This is the Big One!

Relationship (7) E_thermal (total) = (total number of active modes) × ½k_BT

Relationship (8) C_V = dE_thermal/dT, (but only if Q = ΔE_thermal)

Diagramatic Representations

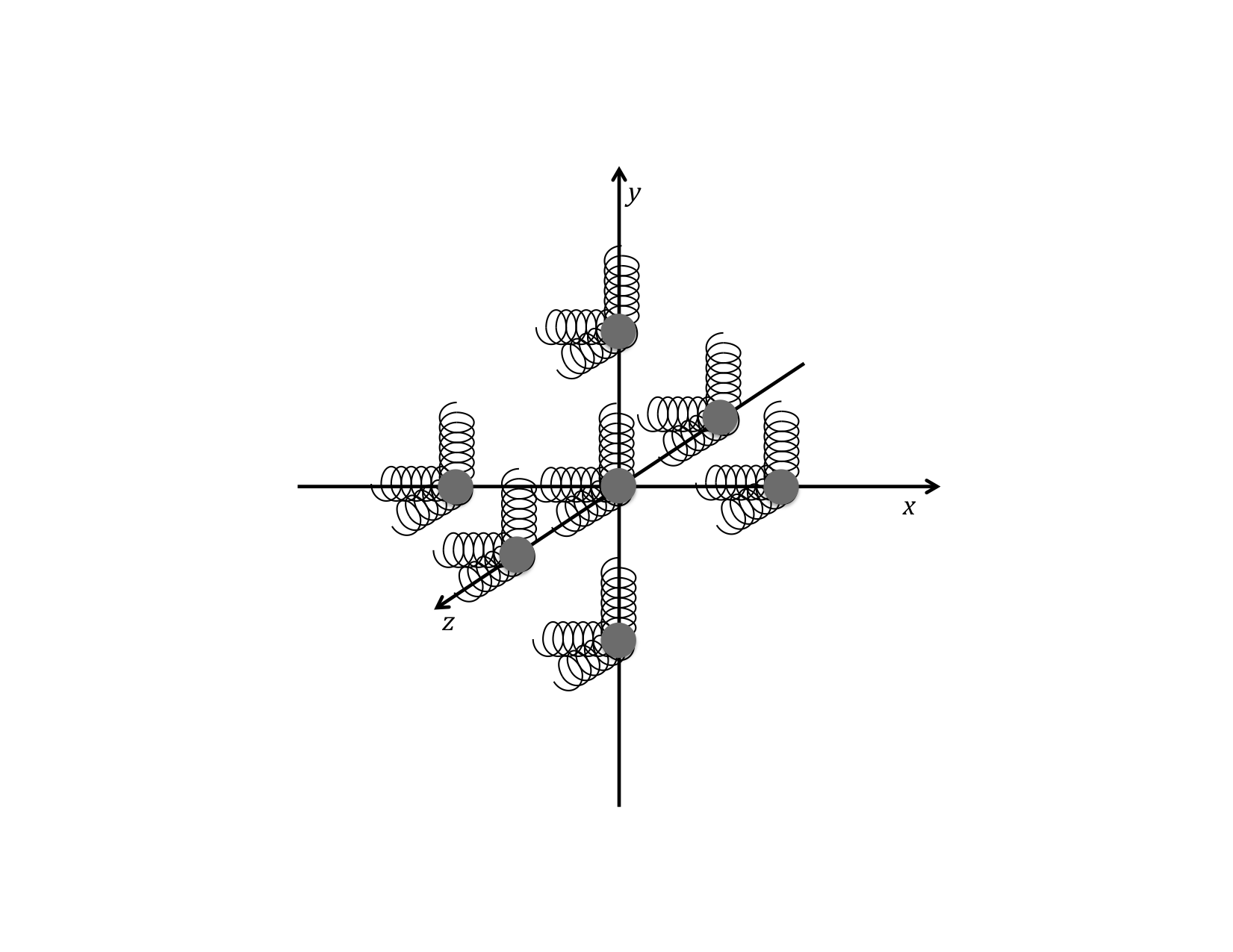
The important point to remember here, is that, regardless of the actual kind of chemical bonding (types of bonds and the geometrical configuration of the bonds) the thermal energy due to the oscillation of the atoms or molecules (treated as rigid particles) is modeled as if they are connected by three independent springs (corresponding to the three independent spatial dimensions in a 3-D universe). This diagram helps us to remember this aspect of the model. Note, however, that the springs in each of the different directions can have different spring constants, which does not, however, have any affect on the this model for thermal energy. The strength simply doesn’t directly matter.

Elaboration

Thermal Equilibrium and Equipartition of Energy Among Modes

There are several important ideas here that all go together. By thermal equilibrium we mean that the random energy fluctuations associated with the motions of the atoms and molecules about their equilibrium positions in a solid or liquid or their random motions when in the gas phase, will over time, become uniformly distributed throughout the entire sample. That is, there will be about as much energy associated with the random energies of a small piece of the sample, but still containing 10¹⁵ or so particles, as in any other same size small piece. This is what we mean by thermal equilibrium on a particle basis. It is also similar to what we would say about the temperature. If we wait for a sufficiently long time, the temperature will become uniform throughout the sample. There would seem to be a direct connection between temperature and the disordered random motion associated with thermal energy. In fact there is a very definite connection.

In the Intro Particle Model of Matter we saw that each atom in a liquid or solid acted as if it vibrated like a spring-mass in each of three dimensions. An interesting question is how many ways does each of these particles “have energy?” We need to think about how many ways a spring-mass has energy. It has a KE and also a PE. We simply stated at the time that the average PE was the same as the average KE. We will simply take this as reasonable at this point. Because of the randomness or disordered-ness of the thermal motions of all the little mass springs in all three of the directions in space, it is plausible that on average, each spring would have the same average PE as would any other spring. And also the same KE as any other spring. In fact this is exactly what happens. It can be rigorously proven for all energies that depend on the square of a position or speed variable. Thus, in addition to working for spring-mass systems, it works for unbound atoms in the gas phase, which will have translational kinetic energies, since these energies depend on the square of their translational speed. It works for molecules that rotate, which will have rotational kinetic energies, since these energies depend on a square of a rotational speed.

So back to our question. How many ways does each spring have “to have” energy? The answer is two: one KE and one PE. How many ways does each particle in a solid or liquid have to have energy? Well, there are three springs and two ways per spring, so it must be six. Each particle in a solid or liquid has six ways to have energy. Now combine this with what we just argued regarding thermal equilibrium. On average, each “way to have energy” would have the same amount of energy when averaged over a sufficiently long period of time. There is a name, or label, for “way to have energy.” The name is “mode.” So we say that each particle has six modes in a solid or liquid. And on average when the sample is in thermal equilibrium, each mode has the same amount of energy (on average). This principle is referred to as the principle of equipartition of energy.

Freezing out of modes

Sometimes, however, the modes don’t “get excited” due to the quantization of energy levels. At low temperatures, the quantum splitting between energy levels, which you are familiar with from chemistry, keeps all but the ground state level from being populated, or having any energy. When this happens, we say that mode is “frozen out.” It is as if it didn’t exist. Frozen out modes cannot share thermal energy. So in the following statements, we usually put in the qualifier, “active modes,” meaning that only active modes share the thermal energy equally among themselves.

Heat Capacity at constant volume

Because we will want to compare values of heat capacity to our predicted values of thermal energy from the particle model of matter, we need to be careful that we are actually comparing the same things. We know that if a force acts through a distance, work will be done by one physical object on another. When we make a heat capacity measurement, we don’t want the sample doing work on the atmosphere or the container it is in. Therefore, we specify that the sample be kept at constant volume during the heat capacity measurement. This is designated with a subscript “v.” The important point here is that we have a way to directly measure the change in the thermal energy by measuring the heat capacity of a sample at constant volume, ensuring all the heat we put into the sample goes to changing its thermal energy and not doing some work by expanding the container or pushing against the air in the room.

Relationship 1

In gases, the translational kinetic energy of each particle can be divided into three independent modes, each one corresponding to one of the three independent spatial dimensions; each particle in a gas has at least these three independent modes due to its kinetic energy.

Because there are no springs connecting the different particles of the gas, there are not any potential energy modes associated with the translational motion of the molecules. However, if the particles are molecules, rather than single atoms, they potentially have rotational and vibrational modes (see Relationship 3).

Relationship 2

In liquids and solids, the oscillations of each particle in its single-particle potential can be modeled as a mass held in place by three perpendicular springs, regardless of the actual bonding arrangement with surrounding particles. The potential and kinetic energies that are associated with each spring act independently of those of the other springs. Each particle in a liquid or solid has at least these six independent modes corresponding to the PE and KE of the three springs.

It is easy to miss the significance of this relationship. It is saying that it doesn’t matter how the actual chemical bonds that hold the molecules and the liquid and solid together are arranged. It doesn’t matter if the solid is crystalline or amorphous. It doesn’t even matter if the substance is in the liquid or solid phase. Every molecule in a liquid or solid behaves the same way in terms of having thermal energy. Each acts as if it were attached to its surroundings with three independent springs. There are two modes for each of these three springs regardless of how many “real” bonds there are.

Relationship 3

In all phases (s, l, g) polyatomic molecules may have additional energies associated with rotations and/or internal vibrations of the molecule. These might contribute additional modes, depending on whether they are active or not at a particular temperature.

Whether or not a mode is active is a quantum mechanical phenomenon and is discussed in Relationship 6. A diatomic molecule, for example, might have vibrational modes that are not active at room temperature, but would typically have rotational modes that are active.

Relationship 4

All of the energies described above are given the label “mode.” The thermal energy of a substance is the total energy in all the active modes of all the particles comprising the substance.

This relationship, in conjunction with the next two, firmly establishes the connection between the macroscopic construct of thermal energy with the microscopic motions and energies of the atomic particles that constitute ordinary matter.

Note: In the model of thermal energy we describe here, we consider only the contributions to the thermal energy (and heat capacity at constant volume) that arise from the motions of the atoms and molecules. It was historically the failure of this model to account for some interesting empirical deviations from the model that led to its extension and the discovery and understanding of the role of energies associated with electrons, magnetic entities, etc. in many solids, especially at low temperatures. This is a classic example of how models have been and continue to be used in science.

Relationship 5

In thermal equilibrium all active modes (of the type considered here) have, on average, the same amount of energy. This principle is referred to as “equipartition of energy.”

This is a rather astounding idea. It says that it doesn’t matter what different kinds of molecules or atoms constitute a substance, or substances, if it is all in thermal equilibrium then each active mode will on average have the same energy.

Relationship 6

The amount of energy, on average, in an active mode is directly proportional to the temperature. The proportionality constant, for historical reasons, is written k_B/2, where k_B is the Boltzmann constant. (k_B = 1.38 × 10^-23 J/K).

In equation form, we can write this relation as E_thermal/mode = ½k_BT

• Since energy is quantized, some modes may not be active, and thus not contribute to the thermal energy at “lower” temperatures. This occurs when k_BT is not considerably greater than the spacing of the quantized energy levels. This is often referred to as “freezing out of modes.”

Now we have a way to numerically estimate when freezing out of modes will start occurring as the temperature is lowered. When the thermal energy per mode, as given approximately as k_BT, approaches several times the size of the energy splitting between the ground state and the first excited state, that mode will begin to be found in the ground state more and more of the time. As the temperature is reduced further, the odds of that mode being bumped into an excited state become less and less and that mode becomes inactive in terms of being able to accept or hold thermal energy.

Relationship 7

The total thermal energy of a substance in thermal equilibrium is equal to the product of [the total number of active modes] and [the average energy per mode].

This relationship is simply the combination of the previous three relationships. The thermal energy is simply the total of the energies in all active modes. On average, the amount of energy in each mode is the same. The value of this average amount of energy is simply a constant times the absolute temperature.

In equation form this relationship is:

E_thermal (total) = (total number of active modes) × ½k_BT

There are various ways to count up the total number of active modes. We can write down an equation for E_thermal for each of these different ways. The equations might look different, but they are all the same basic equation. If you understand this basic relationship as written here, you can quickly write down the expression in whatever form you need. For example, if you need to know the thermal energy of some number of moles of some particular gas at a certain temperature, you would multiply the number of moles by Avogadro’s number by the number of active modes per molecule to get the total number of active modes.

The truly amazing feature of Relationship 7 for the thermal energy is what the thermal energy does not depend on. It doesn’t depend on what kinds of modes are present; any mode is as good as any other. It doesn’t depend on the particular kind of molecule or atom; the modes in a nitrogen molecule contribute the same as those in oxygen. It doesn’t depend on the kinds or strengths of bonds. It doesn’t depend on how hard or soft a substance is. The only thing that matters is how many active modes there are. Consequently, we will see differences in the thermal energy per mole of molecules if we compare molecules with different numbers of atoms, because more atoms contribute more modes per molecule. And we will also see differences due to factors that determine the size of the quantized energy splittings if the temperature is in the range where modes are becoming inactive.

This simple particle model of thermal energy predicts that there will be a small number of classes of substances in which all substances in that class have the same molar thermal energy at the same temperature.

Relationship 8

When the change in thermal energy is due solely to the addition or removal of energy as heat, the heat capacity (C_V) is given by the derivative of thermal energy with respect to temperature.

C_V is the macroscopic variable that corresponds to how many modes there are on a particle basis. The corresponding statement to the last statement in the discussion of Relationship 7 is that there will be a small number of classes of substances in which all substances in that class have the same molar constant volume heat capacity. This is indeed what we empirically find. Significant deviations from these predictions are due to a quantum effect, the freezing out of modes, which occurs as the temperature is lowered closer to absolute zero.