Building on Theory
Learning is rapid. By age 11, some children beat their elders at chess, play music that adults pay to hear, publish poems, or win trophies for spelling or sports or some other learned skill. Others survive on the streets or kill in wars, mastering lessons that no child should have to study. How do they learn so quickly?
Piaget and School-Age Children

Piaget called the cognition of middle childhood concrete operational thought, characterized by new concepts that enable children to use logic. Operational comes from the Latin word operare, meaning “to work; to produce.” By calling this period operational, Piaget emphasized productive thinking.
The school-
A Hierarchy of Categories
One logical operation is classification, the organization of things into groups (or categories or classes) according to some characteristic that they share. For example, family includes parents, siblings, and cousins. Other common classes are animals, toys, and food. Each class includes some elements and excludes others; each is part of a hierarchy.
Food, for instance, is an overarching category, with the next-
Piaget devised many classification experiments. For example, a child is shown a bunch of nine flowers—
Until about age 7, most children answer, “More daisies.” The youngest children offer no justification, but some 6-
Other Logical Concepts

Several logical concepts were already discussed in Chapter 9—in the explanation of ideas that are beyond preoperational children, such as conservation and reversibility.
Another example of concrete logic is seriation, the knowledge that things can be arranged in a logical series. Seriation is crucial for using (not merely memorizing) the alphabet or the number sequence. By age 5, most children can count up to 100, but because they do not yet grasp seriation, they cannot correctly estimate where any particular two-
Concrete operational thought correlates with primary school math achievement, although many other factors contribute (Desoete et al., 2009). For example, logic helps with arithmetic: Children at the stage of concrete operational thought eventually understand that 12 + 3 = 3 + 12 and that 15 is always 15 (conservation), that all the numbers from 20 to 29 are in the 20s (classification), that 134 is less than 143 (seriation), and that if 5 × 3 = 15, then 15 ÷ 5 is 3 (reversibility). (Developmental Link: These concepts are explained in Chapter 9 and detailed in a recently reissued classic, Inhelder & Piaget, 1964/2013a.)
The Significance of Piaget’s Findings

Video Activity: The Balance Scale Task shows children of various ages completing the task and gives you an opportunity to try it, too.
Logic connects to math ability, as just shown. However, researchers find more continuity than discontinuity as children master number skills. Thus, Piaget’s stage idea was mistaken: There is no sudden shift in logic between preoperational and concrete operational intelligence.
Nonetheless, Piaget’s experiments revealed something important. School-
Vygotsky and School-Age Children
Like Piaget, Vygotsky felt that educators should consider children’s thought processes, not just the outcomes. He appreciated the fact that children are curious, creative learners. For that reason, Vygotsky believed that an educational system based on rote memorization rendered the child “helpless in the face of any sensible attempt to apply any of this acquired knowledge” (Vygotsky, 1994a, pp. 356–
The Role of Instruction
Unlike Piaget, Vygotsky stressed the centrality of instruction. Vygotsky believed school could be crucial for cognitive growth. He thought that peers and teachers provide the bridge between developmental potential and needed skills via guided participation and scaffolding, in the zone of proximal development. (Developmental Link: Vygotsky’s theory is discussed in Chapter 2 and Chapter 9.)
Confirmation of the role of social interaction and instruction comes from a U.S. study of children who, because of their school’s entry-
Especially for Teachers How might Piaget’s and Vygotsky’s ideas help in teaching geography to a class of third-
Here are two of the most obvious ways. (1) Use logic. Once children can grasp classification and class inclusion, they can understand cities within states, states within nations, and nations within continents. Organize your instruction to make logical categorization easier. (2) Make use of children’s need for concrete and personal involvement. You might have the children learn first about their own location, then about the places where relatives and friends live, and finally about places beyond their personal experience (via books, photographs, videos, and guest speakers).
Internationally as well, children who begin first grade earlier tend to be ahead in academic achievement compared to those who enter later, an effect noted even at age 15. The author of this study noted that Vygotsky’s theory is not the only one that explains this finding and that these results were not found in every nation (Sprietsma, 2010). However, no matter what explanation is correct, children’s academic achievement seems influenced by social context.
Vygotsky would certainly agree with that, and he would explain those national differences by noting that education before first grade in some nations is far better than in others. Remember that Vygotsky believed education occurs everywhere, not only in school. Children learn as they play with peers, watch television, eat with their families, walk down the street. Every experience, from birth on, teaches them something, with some contexts much more educational than others.

For instance, a study of the reading and math achievement of more than one thousand third-
Families (e.g., parents read to them daily when they were toddlers)
Preschool programs (e.g., a variety of learning activities)
First-
grade curriculum (e.g., emphasis on literacy, with individualized evaluation and instruction)
In this study, most children from families of low socioeconomic status did not experience all three sources of stimulation, but those who did showed more cognitive advances by fifth grade than the average high-
Generally, poverty reduces children’s achievement because they are less likely to have these three sources of stimulation. However, for low-
International Contexts
Vygotsky’s emphasis on sociocultural contexts contrasts with Piaget’s maturational, self-
The same applies to math. If children learn math in school, they are proficient at school math; if they learn math out of school, they are adept at solving mathematical problems in situations similar to the context in which they learned (Abreu, 2008). Ideally, though, children learn math both in and out of school.
Context affects more than academic learning. A stunning example of knowledge acquired from the social context comes from Varanasi, a city in northeast India. Many Varanasi children have an extraordinary sense of spatial orientation: They know whether they are facing north or south, even when they are inside a room with no windows. In one experiment, children were blindfolded, spun around, and led to a second room, yet many still knew which way they were now facing (Mishra et al., 2009). How did they know? Perhaps social context.
In Varanasi, everyone refers to the spatial orientation to locate objects. (The English equivalent might be, not that the dog is sleeping by the door, but that the dog is sleeping southeast.) From their early days, children learn north/south/east/west in order to communicate. By middle childhood, they have an internal sense of direction.
Culture affects how children learn, not just what they learn. This was evident in a two-

In the first session of this study, a Spanish-
A week later, each child individually was told there was some extra material to make the toy that his or her sibling had made the week before, and was encouraged to make the mouse or the frog (whichever one that child had not already made). In this second session, the toy lady did not give the children step-
The purpose of this experiment was to see how much the children had learned by observation the week before. The children from indigenous backgrounds scored higher, needing fewer hints, because they had been more attentive when their siblings made the toy (Silva et al., 2010) (see Figure 12.1).
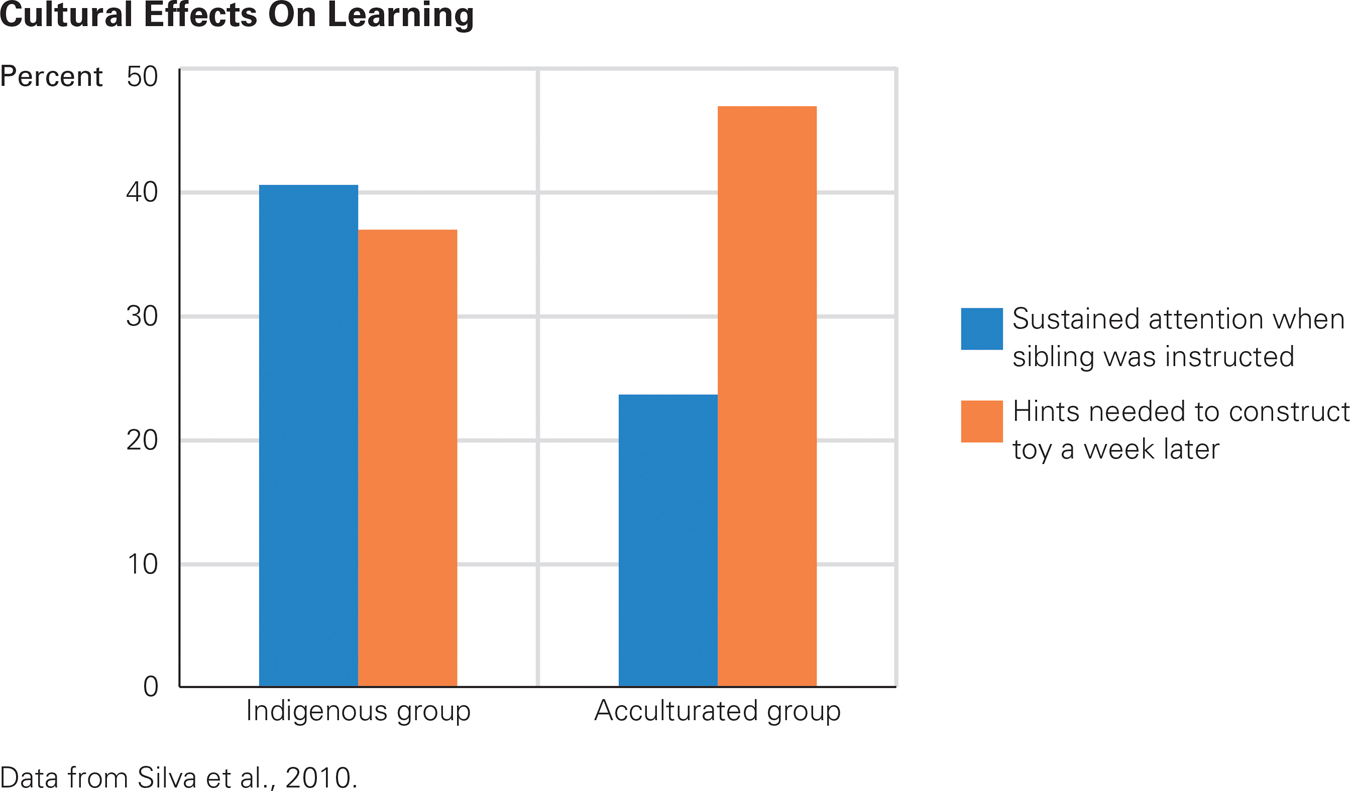
Two Ways to Learn Even when children currently live in the same settings and attend the same schools, they follow family cultural traditions in the way they learn.
The same conclusions have been found in other research. For example, in another study, children born and raised in the United States who are accustomed to learning by observation (as in some Native American cultures) were more proficient at remembering an overheard folktale (Tsethlikai & Rogoff, 2013).
Information Processing
Today’s educators and psychologists regard both Piaget and Vygotsky as insightful. International research confirms the merits of their theories. Piaget described universal changes; Vygotsky noted cultural impact.
A third approach to understanding cognition adds crucial insight. The information-
Thousands of researchers who study cognition can be said to use the information-
The basic assumption of all such research programs is that, like computers, people can access large amounts of information. They then: (1) seek relevant units of information (as a search engine does), (2) analyze (as software programs do), and (3) express their conclusions so that another person can understand (as a networked computer or a printout might do). By tracing the paths and links of each of these functions, scientists better understand the learning process.
The brain’s gradual growth, now seen in neurological scans, confirms the usefulness of the information-

One of the leaders of the information-
Apparently, children do not suddenly grasp the logic of the number system, as Piaget expected at the concrete operational stage. Instead, number understanding accrues gradually, with new and better strategies for calculation tried, ignored, half-
One example is the ability to estimate where a number might fall on a line, such as where the number 53 would be placed on a line from zero to 100. This skill predicts later math achievement (Libertus et al., 2013). U.S. kindergartners are usually lost when asked to do this task; Chinese kindergartners are somewhat better (Siegler & Mu, 2008).
Everywhere, proficiency gradually builds from the first grade on, predicting later math skills (Feigenson et al., 2013). This has led many information-

Arithmetic Strategies: The Research of Robert Siegler
VIDEO PROVIDED BY GEETHA RAMANI AND ROBERT SIEGLER,
CARNEGIE MELLON UNIVERSITY
Curiously, knowing how to count to high numbers seems less important for math mastery than being able to estimate magnitude (Thompson & Siegler, 2010). For example, understanding the size of fractions (e.g., that 3/16 is smaller than 1/4) is connected to a thorough grasp of the relationship between one number and another, a skill that predicts later math achievement internationally, according to a study of school children in China, Belgium, and the United States (Torbeyns et al., 2014). Overall, information processing guides teachers who want to know exactly which concepts and skills are crucial foundations for mastery, not only for math but for reading and writing as well.
Memory
Many scientists who study memory take an information-
Sensory memory (also called the sensory register) is the first component of the human information-
Once some sensations become perceptions, the brain selects the meaningful ones and transfers them to working memory for further analysis. It is in working memory (formerly called short-
Especially for Teachers How might your understanding of memory help you teach a 2,000-
Children this age can be taught strategies for remembering by forming links between working memory and long-
As Siegler’s waves metaphor suggests, memory strategies for processing information do not appear suddenly. Gradual improvement occurs from toddlerhood through adolescence (Schneider & Lockl, 2008). Children develop strategies to increase working memory (Camos & Barrouillet, 2011), and they use these strategies occasionally at first, then consistently.
Cultural differences are evident. For example, many Muslim children are taught to memorize all 80,000 words of the Quran, so they learn strategies to remember long passages. These strategies are unknown to non-
Finally, information from working memory may be transferred to long-
Crucial to long-
Knowledge

Research on information processing finds that the more people already know, the more information they can learn. Having an extensive knowledge base, or a broad body of knowledge in a particular subject, makes it easier to remember and understand related new information. As children gain knowledge during the school years, they become better able to judge what is true or false, what is worth remembering, and what is insignificant (Woolley & Ghossainy, 2013).
Three factors facilitate increases in the knowledge base: past experience, current opportunity, and personal motivation. The last item in this list explains why children’s knowledge base is not what their parents or teachers might prefer. Some schoolchildren memorize words and rhythms of hit songs, know plots and characters of television programs, or can recite the names and histories of basketball players, and yet they do not know whether World War I was in the nineteenth or twentieth century or whether Pakistan is in Asia or Africa.
Motivation provides a clue for teachers: New concepts are learned best if they are connected to personal and emotional experiences. Children who themselves are from South Asia, or who have classmates who are, learn the boundaries of Pakistan more readily.
Control Processes
The mechanisms that put memory, processing speed, and the knowledge base together are control processes they regulate the analysis and flow of information within the system. Control processes include emotional regulation and selective attention (explained in Chapter 10 and Chapter 11, respectively).
Equally important is metacognition, sometimes defined as “thinking about thinking,” understanding how to learn. Metacognition is the ultimate control process because it allows a person to evaluate a cognitive task, determine how to accomplish it, monitor performance, and then make adjustments. According to scholars of cognition, “Middle childhood may be crucial for the development of metacognitive monitoring and study of control processes” (Metcalfe & Finn, 2013, p. 19).
Control processes require the brain to organize, prioritize, and direct mental operations, much as the CEO (chief executive officer) of a business organizes, prioritizes, and directs business operations. For that reason, control processes are also called executive processes, and the ability to use them is called executive function (already mentioned in Chapter 9), which allows a person to step back from the specifics of learning and thinking and consider more general goals and strategies. Executive function is evident whenever people concentrate on the relevant parts of a task, using the knowledge base to comprehend new information and apply memory strategies.
Executive function ability is a foundation for learning in early and middle childhood, measurable already by age 5, and more evident among 10-
Deliberate selectivity is a control process at work. A child can decide to do homework before watching television or to review spelling words before breakfast, creating mnemonics to remember the tricky parts. All these signify executive function.
Both metacognition and control processes improve with age and experience. For instance, in one study, children took a fill-
Sometimes, experience that is not directly related has an impact. This seems to be true for fluently bilingual children, who must learn to inhibit one language while using another. They are advanced in control processes, obviously in language but also in more abstract measures of control (Bialystok, 2010).
Control processes develop spontaneously as the prefrontal cortex matures, but they can also be taught. Sometimes teaching is explicit, more so in some nations (e.g., Germany) than in others (e.g., the United States) (Bjorklund et al., 2009). Examples that may be familiar include spelling rules (“i before e except after c”) and ways to remember how to turn a light bulb (lefty-
Many factors beyond specific instruction affect learning. For example, if children do not master emotional control in early childhood, their school achievement is likely to suffer for years (Bornstein et al., 2013).
Given the complexity of factors and goals, educators disagree as to what should be deliberately taught versus what is best discovered by the child. However, understanding the early steps that lead to later knowledge, as information processing seeks to do, may guide instruction and hence improve learning. Exactly how to do that is an important topic of current research, as you will see in A View from Science on page 391. But first, consider one specific domain of learning that advances during middle childhood, language.
SUMMING UP Every theory of cognitive development recognizes that school-
An information-
WHAT HAVE YOU LEARNED?
Question 12.1
Why did Piaget call cognition in middle childhood concrete operational thought?
Children this age can apply logic to concrete (tangible) things. They cannot yet deal with abstractions or hypothetical situations.Question 12.2
How would one express classification in a category other than those listed in the text, such as transportation or plants?
Ground transportation could be classified as vehicles, mass transit, trains, or bicycles. Plants could be classified as edible and non–edible, or flowering and non– flowering. Question 12.3
How do Vygotsky and Piaget differ in their explanation of cognitive advances in middle childhood?
Piaget contended that cognitive advances occur naturally, as a result of maturation and self–exploration. Vygotsky believed that cognitive advances rely on scaffolding by peers and mentors and guided participation in the zone of proximal development. Question 12.4
How are the children of Varanasi an example of Vygotsky’s theory?
Vygotsky believed that cultures (tools, customs, and mentors) are powerful educators. Moreover, context affects more than academic learning. An example of knowledge acquired from the social context comes from Varanasi, a city in northeast India. Many Varanasi children have an extraordinary sense of spatial orientation: They know whether they are facing north or south, even when they are inside a room with no windows. In one experiment, children were blindfolded, spun around, and led to a second room, yet many still knew which way they were now facing. Researchers believe that social context likely contributes to this extraordinary skill. Specifically, in Varanasi, everyone refers to the spatial orientation to locate objects. From their early days, children learn north/south/east/ west in order to communicate. By middle childhood, they have an internal sense of direction.Question 12.5
How does information-
processing theory differ from traditional theories of cognitive development? Piaget described universal changes; Vygotsky noted cultural impact. The basic assumption of the information processing approach is that, like computers, people can access large amounts of information. They seek specific units of information, analyze, and express their conclusions.Question 12.6
According to Siegler, what is the pattern of learning math concepts?
Number understanding accrues gradually, with new and better strategies for calculation tried, ignored, half–used, abandoned, and finally adopted. Siegler compared the acquisition of knowledge to waves on a beach when the tide is rising. There is substantial ebb and flow, although eventually a new level is reached. Question 12.7
What aspects of memory improve markedly during middle childhood?
Working memory—the processing of current, conscious material— improves markedly in childhood. By the end of childhood, long– term memory is extensive. Question 12.8
How and why does the knowledge base increase in middle childhood?
Having an extensive knowledge base, or a broad body of knowledge in a particular subject, makes it easier to remember and understand related new information. As children gain knowledge during the school years, they become better able to judge what is true or false, what is worth remembering, and what is insignificant. Three factors facilitate increases in the knowledge base: past experience, current opportunity, and personal motivation.Question 12.9
How might executive function help a student learn?
Executive function allows a person to step back from the specifics of learning and thinking and consider more general goals and strategies. Executive function is evident whenever people concentrate on the relevant parts of a task, using the knowledge base to comprehend new information and apply memory strategies. Executive function ability is a foundation for learning in early and middle childhood, measurable already by age 5, and more evident among 10–year– olds than among 4– or 6– year– olds. For example, fourth– grade students can listen to the teacher talk about the river Nile, ignoring classmates who are chewing gum or passing notes. In addition, deliberate selectivity is a control process at work. A child can decide to do homework before watching television or to review spelling words before breakfast, creating mnemonics to remember the tricky parts. All these signify executive function.