Chapter 1.

1.1 Problem Statement

Use the figure below to find all angles between 0 and 2π satisfying the given condition.

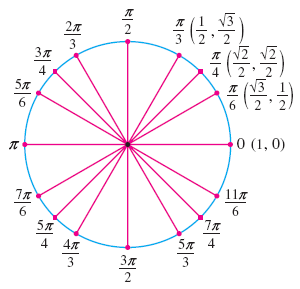
1.2 Step 1

Question Sequence

Question 1.1

Recall that csc(θ) = is tlhXe1fkNqXdqLAu09V4zt1nrKKdBdu2

Incorrect
Correct

Question 1.2

And thus, sin(θ) = is hiSbMvmJe/RtAmcjPbEVlU2Q6gjKgqAp

Incorrect
Correct

1.3 Step 2

Question Sequence

Question 1.3

Since and we are given that , if follows that sin(θ)=2121aHagpiu+V7f7C0spLha8A7NBeMRa

2
Incorrect
Correct

1.4 Step 3

Question Sequence

Question 1.4

The figure shows that in the first quadrant, when θ = 3h1r3BovJpGHdfHF1e45PgxphjpwTJo/RfpbcA==.

2
Correct.
Incorrect.

Question 1.5

The only other quadrant where sin(θ) is positive is the cgdjgMwzgdsN/2V28SBxLETxfR3f1IZ8zuIV8A== quadrant.

Correct.
Incorrect.

1.5 Step 4

We need a second quadrant angle, , for which .

Question Sequence

Question 1.6

From the figure,

θ = 5tzDXePpEG7sPma4qrPqyyLH0PD10LXs2oa5ObfsjWM=

Correct.
Incorrect.

Question 1.7

Thus, for the following values of θ (0 < θ < 2π).

θ = 3h1r3BovJpGHdfHF1e45PgxphjpwTJo/RfpbcA== and 5tzDXePpEG7sPma4qrPqyyLH0PD10LXs2oa5ObfsjWM=

Correct.
Incorrect.