Perfect Competition: Short-Run Decisions
Figure 1 in the previous section presented a perfectly competitive market with an equilibrium price of $200. This translates into a horizontal demand curve for individual firms at that price. Individual firms are price takers in this competitive situation: They can sell as many units of their product as they wish at $200 each.
Marginal Revenue
marginal revenue The change in total revenue from selling an additional unit of output. Because competitive firms are price takers, P = MR for competitive firms.
Economists define marginal revenue as the change in total revenue that results from the sale of one additional unit of a product. Marginal revenue (MR) is equal to the change in total revenue (ΔTR) divided by the change in quantity sold (Δq); thus,
MR = ΔTR/Δq
Total revenue (TR), meanwhile, is equal to price per unit (p) times quantity sold (q); thus:
TR = p × q
In a perfectly competitive market, we know that price will not change based on the output of any one firm. And because marginal revenue is defined as the change in revenue that comes from selling one more unit, marginal revenue is simply equal to price. To verify, suppose a firm sells ten units of a good at $200 each, earning a total revenue of $2,000. If the firm sells eleven units at $200 each, total revenue becomes $2,200. Using our marginal revenue equation, the added revenue a firm receives from selling the 11th unit is $200, which is equal to the product price. Thus determining marginal revenue in a perfectly competitive market is easy. As we will see in later chapters, this gets more complicated in market structures in which firms have some control over price.
Profit-Maximizing Output
Suppose that you own a windsurfing sail manufacturing firm in a perfectly competitive market. Figure 2 shows the price and marginal cost curve that your firm faces while it seeks to maximize profits. As the price and cost curve show, you can sell all you want at $200 a sail. Our first instinct might be to conclude that you will produce all that you can, but this is not the case. Given the marginal cost curve shown in Figure 2, if you produce 85 units, profit will be less than the maximum possible. This is because revenue from the sale is $200, but the 85th sail costs $210 to produce (point b). This means producing this last sail reduces profits by $10.
FIGURE 2
Profit Maximization in the Short Run in Perfectly Competitive Markets If the firm produces 85 standardized windsurfing sails, the marginal cost to produce the last sail exceeds the revenue from its sale, thus reducing the firm’s profits. For the 84th unit produced, marginal cost and price are both equal to $200, therefore the firm earns a normal return from producing this unit. Producing only 83 units means relinquishing the normal return that could have been earned from the 84th sail. Hence, the firm maximizes profits at an output of 84 (point e), where MC = MR = P = $200.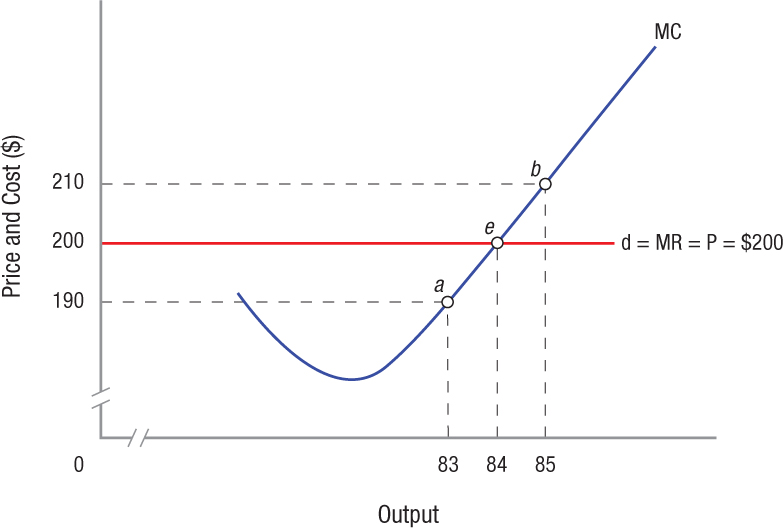
Suppose instead that you produce 84 sails. The revenue from selling the 84th unit (MR) is $200. This is precisely equal to the added cost (MC) of producing this unit, $200 (point e). Therefore, your firm earns zero economic profit by producing and selling the 84th sail. Zero economic profit, or normal profits, mean that your firm is earning a normal return on its capital by selling this 84th sail. If you produce only 83 sails, however, the additional cost (point a) will be less than the price, and you will have to relinquish the normal return associated with the 84th sail. Profits from selling 83 sails will therefore be lower than if 84 sails are sold because the normal return on the 84th sail is lost.
profit maximizing rule Firms maximize profit by producing output where MR = MC. No other level of output produces higher profits.
These observations lead us to a profit maximizing rule: A firm maximizes profit by continuing to produce and sell output until marginal revenue equals marginal cost (MR = MC). As we will see in subsequent chapters, this rule applies to all firms, regardless of market structure.
Economic Profits
Continuing with the example of your windsurfing sail manufacturing firm, assume that the market has established a price of $200 for each sail. Your marginal revenue and cost curves are shown in Figure 3 (the MR and MC curves are the same as those shown in Figure 2).
FIGURE 3
A Perfectly Competitive Firm Earning Economic Profits Profits are maximized where marginal revenue equals marginal cost (MR = MC), or at an output of 84 and a price of $200. Price minus average total cost equals average profit per unit, represented by the distance ab. Average profit per unit times the number of units produced equals total profit; this is represented by area cfab.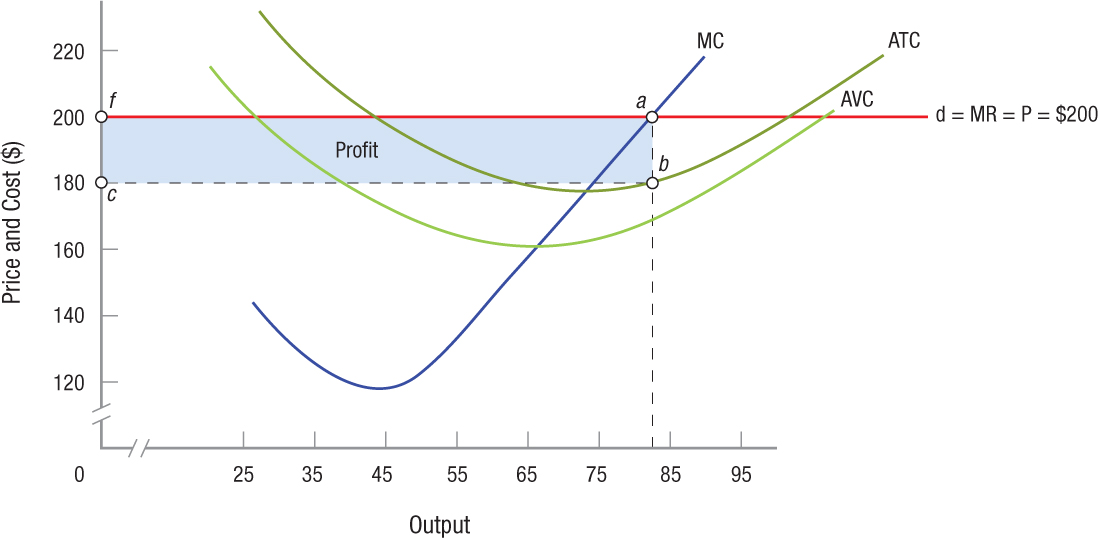
Earlier, we found that profits are maximized when your firm is producing output such that MR = MC, in this case, 84 sails. Looking at Figure 3, we see that profits are maximized at point a, because this is where MR = MC at $200. We can compute the profit in this scenario by multiplying average profit (profit per unit) by output. Average profit equals price minus average total costs (P − ATC). In the figure, the average total cost of producing 84 sails is $180 (point b). Thus, when 84 sails are produced, average profit is the distance ab in Figure 3, or $200 − $180 = $20. Total profit, or average profit times output [(P − ATC) × Q], is $20 × 84 = $1,680; this is represented in the figure by area cfab. This area also is equivalent to total revenue minus total cost (TR − TC).
Note that there is a profit-maximizing point. The competitive firm cannot produce and produce—it has to take into consideration its costs. Therefore, for the price-taking competitive firm, its cost structure is crucial.
Five Steps to Maximizing Profit
Profit maximization is an important goal of any firm in any market structure. Yet, understanding revenue and cost curves can be frustrating given the many market structures and the different types of curves facing each market. Wouldn’t it be useful to have a single approach when solving for a profit-maximizing outcome?
Let’s review how we solved for the profit-maximizing equilibrium in the previous section, but this time using five simple steps that are illustrated in Figure 4.
FIGURE 4
Five-Step Process to Determine Maximum Profit The five steps shown provide a consistent approach to determining the profit-maximizing output and price and the area representing profit using revenue and cost curves in any market structure.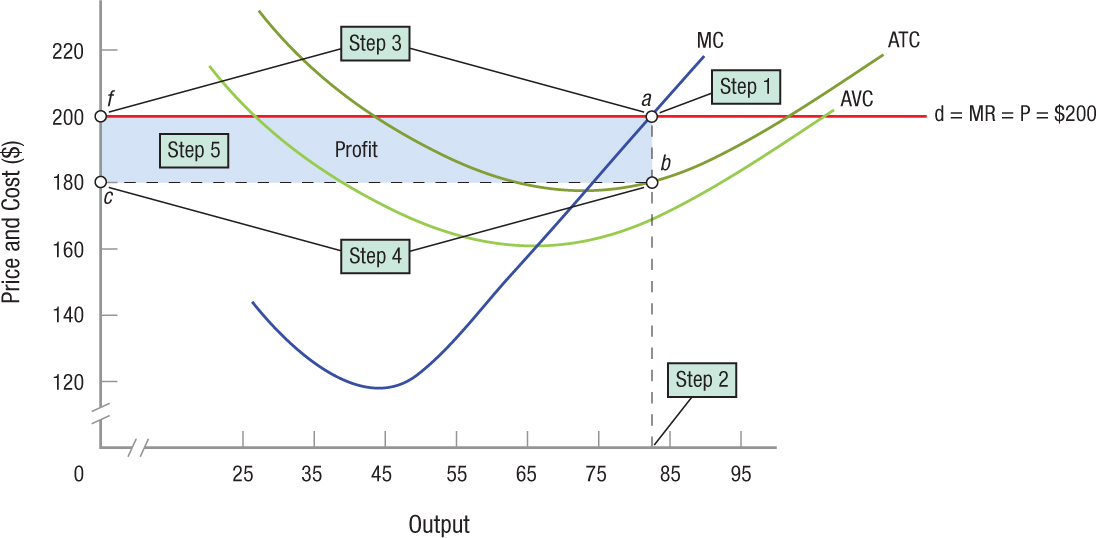
Step 1: Find the point at which marginal revenue (MR) equals marginal cost (MC). Remember that in a perfectly competitive market, MR equals price.
Step 2: At the point at which MR = MC, find the corresponding point on the horizontal axis; this is the profit-maximizing output.
Step 3: At the profit-maximizing output, draw a line straight up to the demand curve (which is equal to MR in a perfectly competitive market) and then to the vertical axis. This is the profit-maximizing price.
Step 4: Again using the profit-maximizing output, draw a line straight up to the average total cost curve, and then to the vertical axis. This is the average total cost per unit.
Step 5: Find the profit, which is the rectangle formed between the profit-maximizing price and average total cost on the vertical axis, and the profit-maximizing output on the horizontal axis.
Some of these steps might seem redundant. However, using this approach will help you to identify the profit-maximizing price, output, and profit in any type of market structure, as we’ll see in the next two chapters. It is therefore useful to remember these steps now when the diagrams are easier to follow.
Normal Profits
When the price of a windsurfing sail is $200, your firm earns economic profits. Consider what happens, however, when the market price falls to $175 a sail. This price happens to be the minimum point on the average total cost curve, corresponding to an output of 75 sails. Figure 5 shows that at the price of $175, the firm’s demand curve is just tangent to the minimum point on the ATC curve (point e), which means that the distance between points a and b in Figure 3 has shrunk to zero. By producing 75 sails, your firm earns normal profits, or zero economic profit.
FIGURE 5
A Perfectly Competitive Firm Earning Normal Profits (Zero Economic Profit) If the market sets a price of $175, the firm’s demand curve is tangent to the minimum point on the ATC curve (point e). The best the firm can do under these circumstances is to earn normal profits on the sale of 75 windsurfing sails.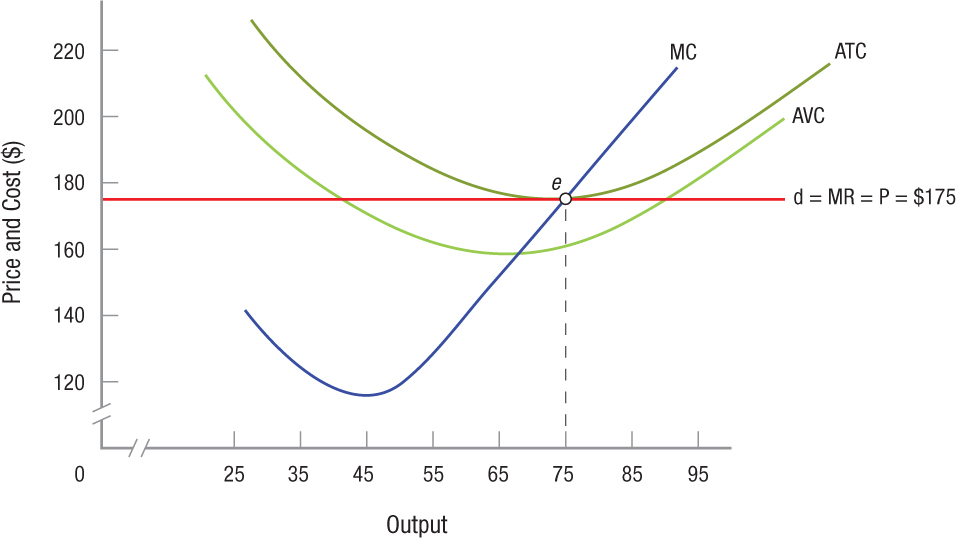
normal profits Equal to zero economic profits; where P = ATC.
Remember that when a firm earns zero economic profit, it is generating just enough income to keep investor capital in the business. When the typical firm in an industry is earning normal profits, there are no pressures for firms to enter or leave the industry. As we will see in the next section, this is an important factor in the long run.
Loss Minimization and Plant Shutdown
Assume for a moment that an especially calm summer with little wind leads to a decline in the demand for windsurfing sails. Assume also that, as a consequence, the price of windsurfing sails falls to $170. Figure 6 illustrates the impact on your firm. Market price has fallen below your average total cost of production (which takes into account the fixed cost, which in this case is $1,000, an amount determined in the previous chapter), but remains above your average variable cost. Profit maximization—or, in this case, loss minimization—requires that you produce output at the level at which MR = MC. That occurs at point e, where output falls to 65 units.
FIGURE 6
A Perfectly Competitive Firm Minimizing Losses Assume that the price of windsurfing sails falls to $170 a sail. Loss minimization requires an output at which MR = MC, in this case at 65 units (point e). Average total cost is $178 (point c), therefore loss per unit is equal to $8. Total loss is equal to $8 × 65 = $520. Notice that this is less than the fixed costs ($1,000) that would have to be paid even if the plant was closed.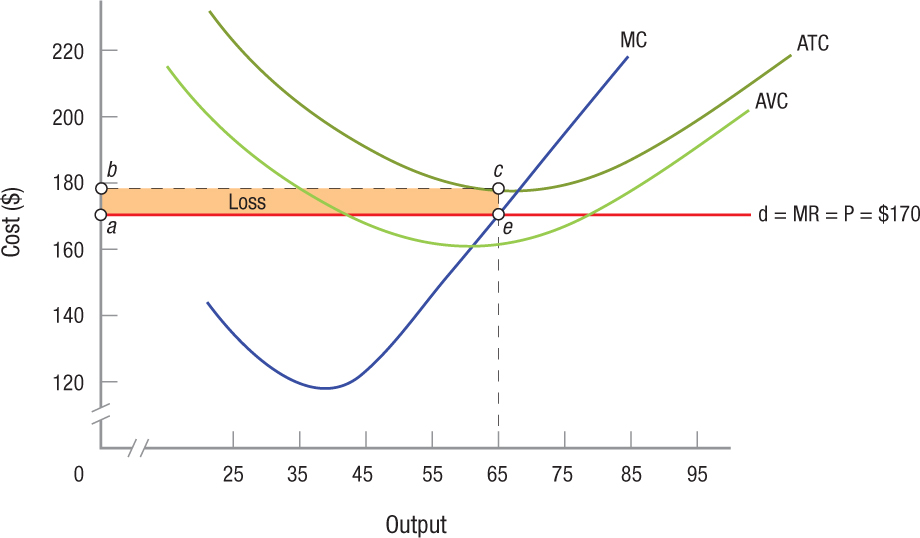
Using Figure 6, at 65 units the average total cost at this production level is $178, thus with a market price of $170, loss per unit is $8. The total loss on 65 units is $8 × 65 = $520, corresponding to area abce.
shutdown point When price in the short run falls below the minimum point on the AVC curve, the firm will minimize losses by closing its doors and stopping production. Because P < AVC, the firm’s variable costs are not covered, therefore by shutting the plant, losses are reduced to fixed costs only.
These results may look grim, but consider your alternatives. If you were to produce more or fewer sails, your losses would just mount. You could, for instance, furlough your employees. But you will still have to pay your fixed costs of $1,000. Without revenue, your losses would be $1,000. Therefore, it is better to produce and sell 65 sails, taking a loss of $520, thereby cutting your losses nearly in half.
But what happens if the price of windsurfing sails falls to $160? Such a scenario is shown in Figure 7. Your revenue from the sale of sails has fallen to a level just equal to variable costs. If you produce and sell 60 units of output (where MR = MC), you will be able to pay your employees their wages, but have nothing left over to pay your overhead; thus your loss will be $1,000. Point e in Figure 7 represents a shutdown point, because your firm will be indifferent to whether it operates or shuts down—you lose $1,000 either way.
FIGURE 7
Plant Shutdown in a Perfectly Competitive Industry When prices fall below $160, or below the minimum point of the AVC curve, losses begin to exceed fixed costs. The firm will close if price falls below this minimum point (point e); this is the firm’s shutdown point.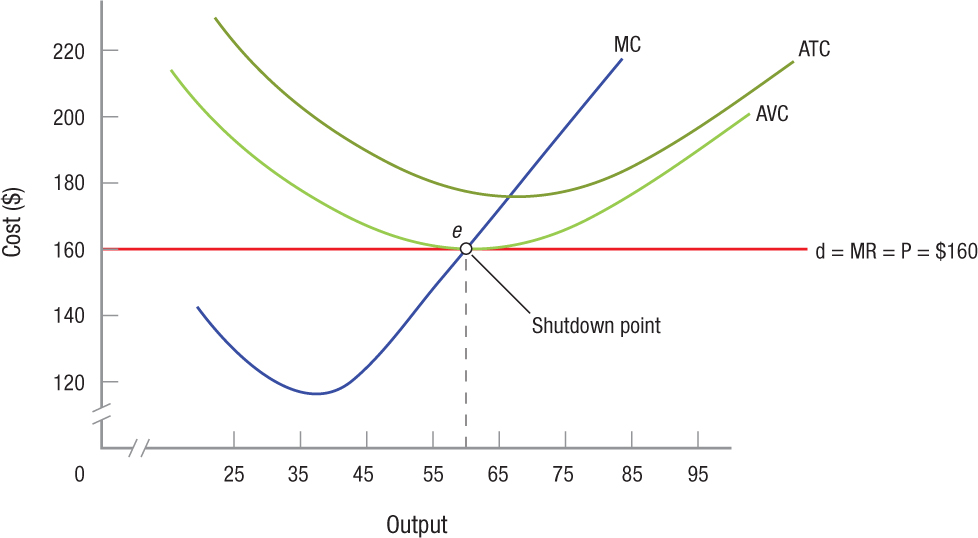
NOBEL PRIZE HERBERT SIMON (1916–2001)

When he was awarded the Nobel Prize for Economics in 1978, Herbert Simon (1916–2001) was an unusual choice on two fronts. First, he wasn’t an economist by trade; he was a professor of computer science and psychology at the time of his award. Second, Simon’s major contribution to economics was a direct challenge to one of the basic tenets of economics: Firms, in fact, do not always act to maximize profits.
In his book Administrative Behavior, Simon approached economics and the behavior of firms from his outsider’s perspective. Simon thought real-world experience showed that firms are not always perfectly rational, in possession of perfect information, or striving to maximize profits. Rather, he proposed, as firms grow larger and larger, the access to perfect information becomes a fiction and that firms are run by individuals whose decisions are altered by their inability to remain perfectly and completely rational.
To Simon, the reality is not that firms tilt at the mythical windmill of maximizing profits, but that, as he said in his Nobel Prize acceptance speech, they recognize their limitations and instead try to come up with an “acceptable solution to acute problems” by setting realistic goals and making reasonable assessments of their successes or failures.
Simon’s views provided a new approach to analyzing market structure models. Simon brought data, theories, and knowledge from other disciplines and paved the way for behavioral economics (discussed in Chapter 6) to eventually become a prominent field of economics by broadening its scope and applying more realistic conditions found in the marketplace.
If prices continue to fall below $160 a sail, your losses will grow still further, because revenue will not even cover wages and other variable expenses. Once prices drop below the minimum point on the AVC curve (point e in Figure 7), losses will exceed total fixed costs and your loss minimizing strategy must be to close the plant. It follows that the greatest loss a firm is willing to suffer in the short term is equal to its total fixed costs. Remember that the firm cannot leave the industry at this point, because market participation is fixed in the short run, but it can shut down its plant and stop production.
How practical is it to shut down production? That depends on the size of the firm and whether short-run losses are temporary or indicative of a permanent trend. The owner of a small kiosk selling decorative baskets might shut down and lay off the staff if losses mount and prospects for future profits are grim, while the owner of a fishing boat might furlough her employees during the off-season, with the expectation of resuming operations later on when prices recover and profits return.
The Short-Run Supply Curve
A glance at Figure 8 will help to summarize what we have learned so far. As we have seen, when a competitive firm is presented with a market price of P0, corresponding to the minimum point on the AVC curve, the firm will produce output of q0. If prices should fall below P0, the firm will shut its doors and produce nothing. If, on the other hand, prices should rise to P1, the firm will sell q1 and earn normal profits (zero economic profits). And if prices continue climbing above P1, say, to P2, the firm will earn economic profits by selling q2. In each instance, the firm produces and sells output where MR = MC.
FIGURE 8
The Short-Run Supply Curve for a Perfectly Competitive Firm If prices fall below P0, the firm will shut its doors and produce nothing. For prices between P0 and P1, the firm will incur losses, but these losses will be less than fixed costs, thus the firm will remain in operation and produce where MR = MC. At a price of P1, the firm earns a normal return. If price should rise above P1 (e.g., to P2), the firm will earn economic profits by selling an output of q2. The portion of the MC curve above the minimum point on the AVC curve, here thickened, is the firm’s short-run supply curve.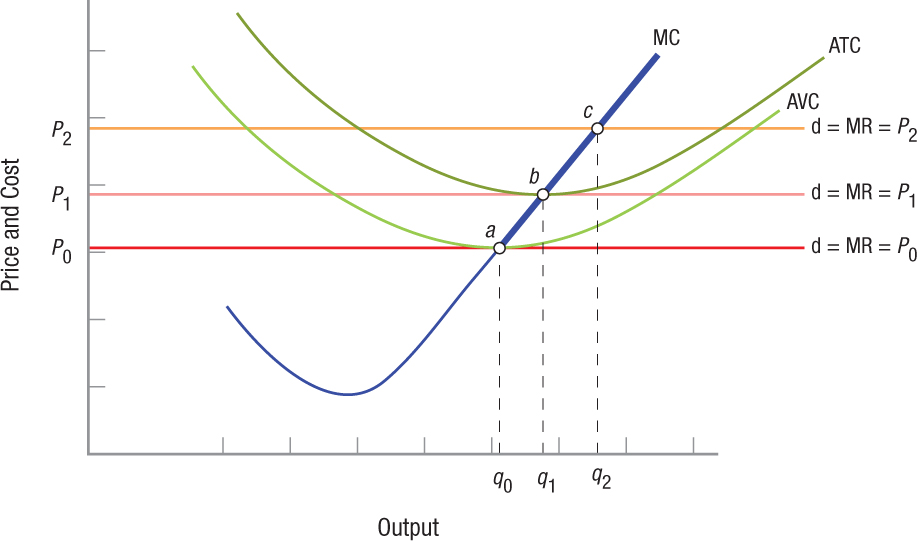
short-run supply curve The marginal cost curve above the minimum point on the average variable cost curve.
From this quick summary, we can see that a firm’s short-run supply curve is equivalent to the MC curve above the minimum point on the AVC curve. This curve, shown as the thickened portion of the MC curve in Figure 8, shows how much the firm will supply to the market at various prices, keeping in mind that it will supply no output at prices below the shutdown point.
Keep in mind also that the short-run supply curve for an industry is the horizontal summation of the supply curves of the industry’s individual firms. To obtain industry supply, in other words, we add together the output of every firm at various price levels.
PERFECT COMPETITION: SHORT-RUN DECISIONS
- Marginal revenue is the change in total revenue from selling an additional unit of a product.
- Perfectly competitive firms are price takers, getting their price from markets, enabling them to sell all they want at the going market price. As a result, their marginal revenue is equal to product price and the demand curve facing the perfectly competitive firm is a horizontal straight line at market price.
- Perfectly competitive firms maximize profit by producing that output where marginal revenue equals marginal cost (MR = MC).
- When price is greater than the minimum point of the average total cost curve, firms earn economic profits.
- When price is just equal to the minimum point of the average total cost curve, firms earn normal profits.
- When price is below the minimum point of the average total cost curve, but above the minimum point of the average variable cost curve, the firm continues to operate, but earns an economic loss.
- When price falls below the minimum point on the average variable cost curve, the firm will shut down and incur a loss equal to total fixed costs.
- The short-run supply curve of the firm is the marginal cost curve above the minimum point on the average variable cost curve.
QUESTION: Describe why profit-maximizing output occurs where MR = MC. Does this explain why perfectly competitive firms do not sell “all they can produce”?
Keep in mind that marginal cost is the additional cost to produce another unit of output, and price equals marginal revenue and is the additional revenue from selling one more unit of the product. If MR is greater than MC, the firm earns more revenue than cost by selling that next unit, therefore the firm will sell up to the point at which MR = MC. At that last unit at which MR = MC, the firm is earning a normal profit on that unit (a positive accounting profit). When MC > MR, the firm is spending more to produce that unit than it receives in revenue and is losing money on that last unit, lowering overall profits. Thus, firms will not produce and sell all they can produce: They will produce and sell up to the point at which MR = MC.