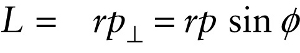Magnitude of the angular momentum of a particle (8-23)

Question 1 of 3

Question

Component of the particle’s linear momentum perpendicular to the vector $\vec{r}$ from rotation axis to particle

{"title":"Magnitude of the angular momentum of a particle","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,3,24,35\"}]"} {"title":"Component of the particle’s linear momentum perpendicular to the vector r from rotation axis to particle","description":"Correct!","type":"correct","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"105,9,128,41\"},{\"shape\":\"rect\",\"coords\":\"228,6,273,37\"}]"} {"title":"Distance from the rotation axis to the particle","description":"Incorrect","type":"incorrect","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"89,10,107,35\"},{\"shape\":\"rect\",\"coords\":\"182,12,201,37\"}]"}

Review

To relate angular momentum to Equation 26-18, we express $L$ in terms of the mass of the electron $m_{\mathrm{e}}$ , its speed $v$ , and its distance from the center of the atom $r$ using Equation 8-23:

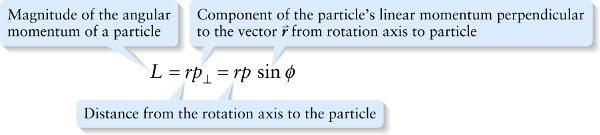
Since the electron moves in a circle, the vector from the rotation axis to the particle always has the same radius $r$ and is always perpendicular to the momentum vector.