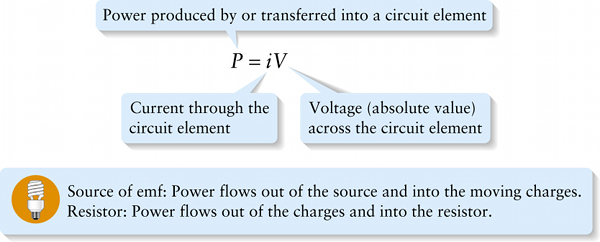
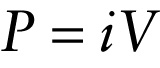Chapter 21. Power for a circuit element (18-23)

Question

cycX4QBT7hELuiaIbHfyW2kot8BbdB5J6Sr2ifCQXCfCm09b4rGv4cFNa2hW8msxt1SHcxT/NEFEfUYB

{"title":"Power produced by or transferred into a circuit element","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,8,37,55\"}]"} {"title":"Voltage (absolute value) across the circuit element","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"121,5,159,56\"}]"} {"title":"Current through the circuit element","description":"Incorrect","type":"incorrect","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"97,9,121,55\"}]"}

Question

pYqsB7Y/f7GtcioDqEK+flVt3ljsSrls9BBB7i5VpIu2RA3i+PQq6rS4J48dvGIdYYZ14RfQjYs=

{"title":"Power produced by or transferred into a circuit element","description":"Incorrect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,8,37,55\"}]"} {"title":"Voltage (absolute value) across the circuit element","description":"Correct!","type":"correct","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"121,5,159,56\"}]"} {"title":"Current through the circuit element","description":"Incorrect","type":"incorrect","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"97,9,121,55\"}]"}

Question

JgRerD921jrPkHYxLorY8eyLfWUOU9oWRui1fZ97JnYJI7Vmr9aMEQ==

{"title":"Power produced by or transferred into a circuit element","description":"Incorrect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,8,37,55\"}]"} {"title":"Voltage (absolute value) across the circuit element","description":"Incorrect","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"121,5,159,56\"}]"} {"title":"Current through the circuit element","description":"Correct!","type":"correct","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"97,9,121,55\"}]"}

Review

Equation 18-23 tells us that the same amount \(P\) of electric power can be delivered at any voltage \(V\), provided that the product of current and voltage remains the same. This is true for ac circuits as well as dc circuits. For a power line, it’s best to use high voltage and low current. The reason is that a long power line has a substantial resistance \(R\), and so some of the energy being carried by the current goes into heating the power line rather than being transmitted to the end user. The rate at which energy is dissipated in a resistor with resistance \(R\) is given by Equation 18-24 (see Section 21-2), which we can write as \(P = i^2R\). To minimize this energy loss, the current \(i\) should be as small as possible. It then follows from Equation 18-23 that the voltage \(V\) must be as large as possible.