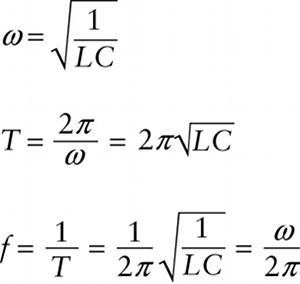Loading [MathJax]/jax/output/HTML-CSS/jax.js

Angular frequency, period, and frequency for an LC circuit (21-23)

Balloon Art Concept Check: Click on the part of the equation that is described by the bubble text shown

Question 1 of 3

Question

Frequency
Feedback
{"title":"Angular frequency","description":"Incorrect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,27,29,50\"}]"} {"title":"Period","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"1,127,23,153\"}]"} {"title":"Frequency","description":"Correct!","type":"correct","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"1,232,15,267\"}]"}

Review

Table 21-1 tells us that k corresponds to 1/C and m corresponds to L. So the angular frequency, period, and frequency for the oscillations of an LC circuit are

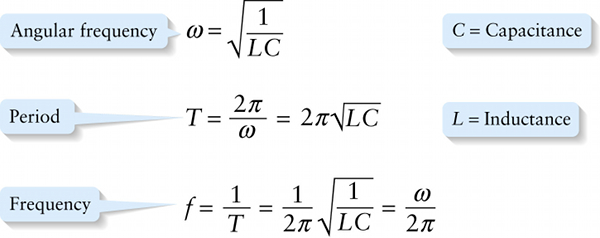
The smaller the product LC of inductance and capacitance, the greater the angular frequency and frequency of the oscillations and the shorter the oscillation period.