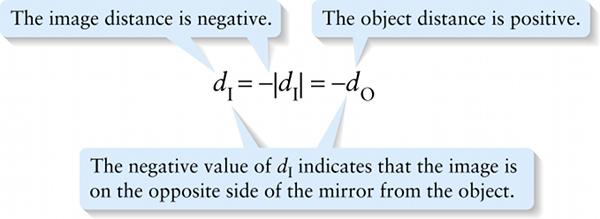
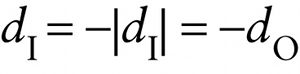Image distance for a plane mirror (24-1)

Question 1 of 3

Question

The image distance is negative.

{"title":"The image distance is negative.","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"3,2,31,45\"},{\"shape\":\"rect\",\"coords\":\"122,3,153,47\"}]"} {"title":"The object distance is positive.","description":"Incorrect","type":"incorrect","color":"#008000","code":"[{\"shape\":\"rect\",\"coords\":\"245,3,277,46\"}]"} {"title":"The negative value of d sub I indicates that the image is on the opposite side of the mirror from the object.","description":"Incorrect","type":"incorrect","color":"#000080","code":"[{\"shape\":\"rect\",\"coords\":\"235,1,288,54\"},{\"shape\":\"rect\",\"coords\":\"2,2,49,57\"}]"}

Review

The geometry of light rays requires that an image be as far behind the front of the mirror as the object is in front of the mirror. In other words, the image distance $d_{\mathrm{I}}$ has the same magnitude as the object distance $d_{\mathrm{O}}$ , We’ll use the convention that a point on the reflective side of the mirror (to the left of the mirror in Figure 24-5) is at a positive distance, while a point on the back side of the mirror (to the right of the mirror in Figure 24-5) is at a negative distance. So in Figure 24-5 the object distance $d_{\mathrm{O}}$ is positive but the image distance $d_{\mathrm{I}}$ is negative. We can write the relationship between the image and object distances for a plane mirror as