Chapter 3
Question 3.1
The O=O bond is stronger; its atoms are closer together.
Question 3.2
The enantiomers have the same chemical formula, so the answer in each case is yes.
Question 3.3
(d) It lowers the activation energy for a reaction.
Question 3.4
pH 5
Question 3.5
No. Peptides have directionality, established by the N-
Question 3.6
Activation energy is larger in the reverse direction.
Question 3.7
The number of moles of NaCl remains unchanged; the concentration of NaCl is reduced by 50%.
Question 3.8
The second law of thermodynamics, which states that in any chemical or physical process, the entropy of the universe tends to increase.
Question 3.9
A and B are of equal length. Resonance structures, by definition, represent the shared distribution of electrons between sets of bonding atoms, and hence the average bond lengths are the same.
Question 3.10
(b) Measuring rates.
Question 3.11
(a) 4.76. (b) 9.19. (c) 4.0. (d) 4.82.
Details: pH = −log [H+]
−log [1.75 × 10−5] = 4.76
−log [6.50 × 10−10] = 9.19
−log [1.0 × 10−4] = 4.0
−log [1.5 × 10−5] = 4.82
Question 3.12
(a) 1.5 × 10−4 m. (b) 3.0 × 10−7 m. (c) 7.8 × 10−12 m.
Details: [H+] = 10−pH
[H+] = 10−3.82 = 1.5 × 10−4 mol/L
[H+] = 10−6.53 = 3.0 × 10−7 mol/L
[H+] = 10−11.11 = 7.8 × 10−12 mol/L
Question 3.13
(a) 5.00. (b) 4.22. (c) 5.40. (d) 4.70. (e) 3.70.
Details: pH = pKa + log [acetate]/[acetic acid]
pH = 4.70 + log (2/1) = 5.00
pH = 4.70 + log (1/3) = 4.22
pH = 4.70 + log (5/1) = 5.40
pH = 4.70 + log 1 = 4.70
pH = 4.70 + log (1/10) = 3.70
Question 3.14
(a) 4.3. (b) pH decrease of 0.12. (c) pH decrease of 4.4.
Details:
pH = pKa + log [lactate]/[lactic acid] = 3.60 + log (0.05/0.01) = 3.60 + 0.69897 = 4.3
Strong acids ionize completely:
0.005 L × 0.5 mol/L = 0.0025 mol of H+ added
The added acid converts some of the salt form (lactate) to the acid form (lactic acid):
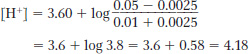
The change in pH = 4.30 − 4.18 = 0.12.
pH = −log [H+]
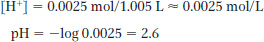
The pH of pure water is 7.0, so change in pH = 7.0 − 2.6 = 4.4.
Question 3.15
pKa 7.2
Details: At pH 2.0, 50% of the group with pKa = 2.0 is ionized (pKa = pH). Amount of base added = 0.075 L × 0.1 mol/L = 0.0075 mol. Amount of compound = 0.1 L × 0.1 mol/L × 0.01 mol. A pH increase to 6.72 completely titrates the remainder of the group with the low pKa (because pH >> pKa). So, 50% × 0.01 mol of compound requires 0.005 mol of base to titrate the rest of that group. Thus, 0.0075 mol of base added − 0.005 mol of base used = 0.0025 mol of base remaining to titrate the second group.
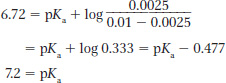
Question 3.16
The ring is flat/planar. The conjugated double bonds in the ring produce considerable resonance, such that all bonds in the ring have a partial double-
S-
Question 3.17
Free rotation is restricted about the bond with angle ω. Due to resonance between the N—
Question 3.18
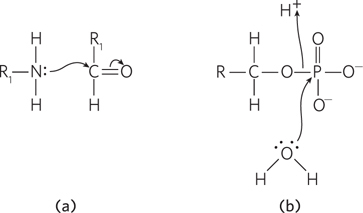
Question 3.19
The molecule is l-alanine (see Figure 3-23). This is an example of an l-amino acid that is dextrorotatory.
Question 3.20
The biochemist must add 13 mL of 1.0 m NaOH.
Details: pH = pKa + log [base] / [acid]. The solution contains 0.01 mol of histidine. The beginning pH is 1.82, identical to one of the pKa values. Since log [base] / [acid] = 0, [base] / [acid] = 1; the concentrations of base and acid are the same. For the ionizable group (α-carboxyl), there are effectively 0.005 mol of the acid form and 0.005 mol of the basic form. This group is titrated first by the NaOH, requiring 0.005 mol of NaOH (5 mL of 1 m solution) for complete titration. To reach pH 6.60, part of the next ionizable group (side-