KEPLER’S AND NEWTON’S LAWS
Until Kepler’s time, astronomers had assumed that heavenly objects move in circles. For philosophical and aesthetic reasons, circles were considered the most perfect and most harmonious of all geometric shapes. However, using circular orbits failed to yield accurate predictions for the positions of the planets. For years, Kepler tried to find a shape for orbits that would fit Tycho’s observations of the planets’ positions against the background of distant stars. Finally, he began working with a geometric form called an ellipse.
2-5 Kepler’s laws describe orbital shapes, changing speeds, and the lengths of planetary years
You can draw an ellipse as shown in Figure 2-8a. Each thumbtack is at a focus (plural foci). The longest diameter (major axis) across an ellipse passes through both foci. Half of that distance is called the semimajor axis. In astronomy, the length of the semimajor axis is also the average distance between a planet and the Sun.
 To Kepler’s delight, the ellipse turned out to be the curve for which he had been searching. Predictions of the locations of planets based on elliptical paths were in very close agreement with where the planets actually were. Keep in mind that the following three laws that Kepler discovered merely quantified (gave equations for) observations that Tycho had made—
To Kepler’s delight, the ellipse turned out to be the curve for which he had been searching. Predictions of the locations of planets based on elliptical paths were in very close agreement with where the planets actually were. Keep in mind that the following three laws that Kepler discovered merely quantified (gave equations for) observations that Tycho had made—

Kepler published his discovery of elliptical orbits in 1609 in a book known today as New Astronomy. This important discovery is now considered the first of Kepler’s laws:
Kepler’s First Law: The orbit of each planet around the Sun is an ellipse with the Sun at one focus.
The shapes of ellipses have two extremes. The roundest ellipse, occurring when the two foci merge, is a circle. The most elongated ellipse is nearly a straight line. The shape of a planet’s orbit around the Sun is described by its orbital eccentricity, designated by the letter e, which ranges from 0 (a circular orbit) to just under 1.0 (nearly a straight line). Figure 2-
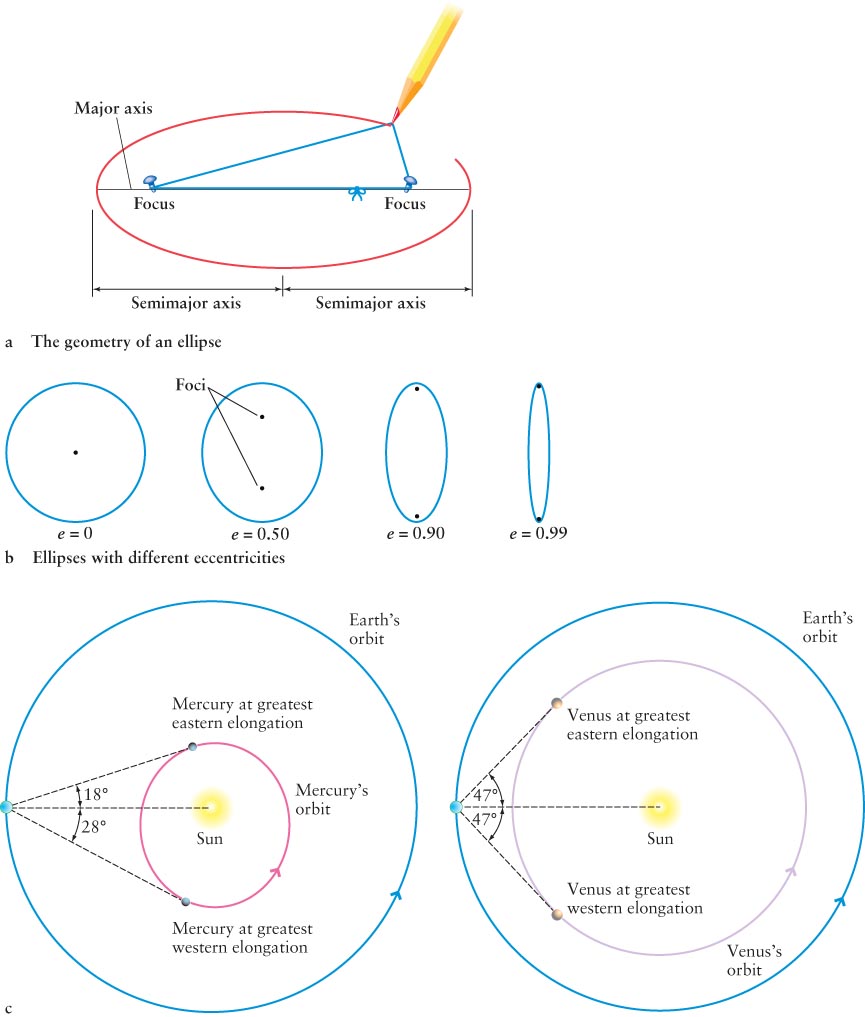
38
 Tycho’s observations also showed Kepler that planets do not move at uniform speeds along their orbits. Rather, a planet moves fastest when it is nearest the Sun, a point on its orbit called perihelion. Conversely, a planet moves most slowly when it is farthest from the Sun, a point called its aphelion.
Tycho’s observations also showed Kepler that planets do not move at uniform speeds along their orbits. Rather, a planet moves fastest when it is nearest the Sun, a point on its orbit called perihelion. Conversely, a planet moves most slowly when it is farthest from the Sun, a point called its aphelion.
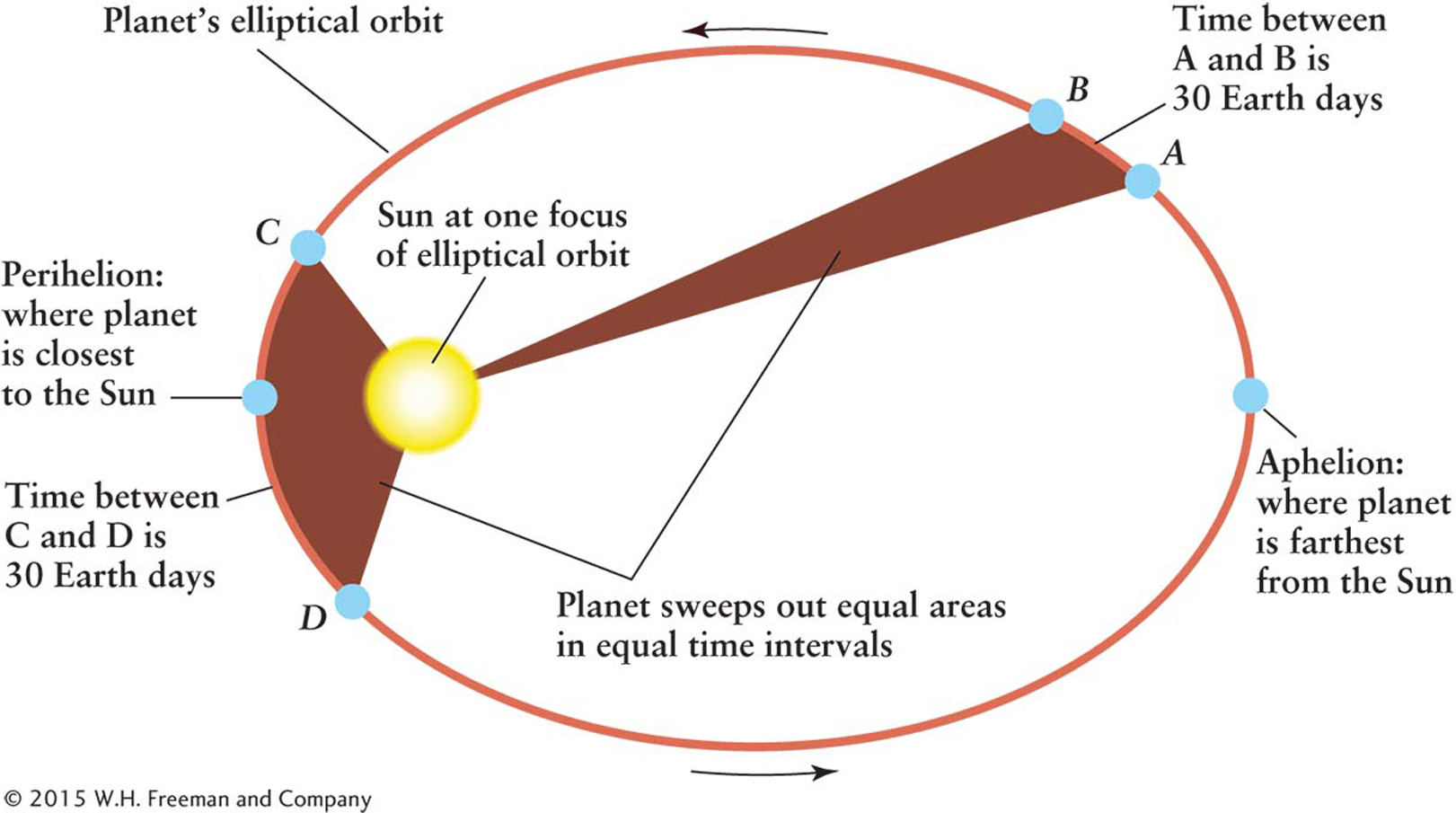
After much trial and error, Kepler discovered a way to describe how fast a planet moves anywhere along its orbit. This discovery, also published in New Astronomy, is illustrated in Figure 2-9. Suppose that it takes 30 days for a planet to go from point A to point B. During that time, the line joining the Sun and the planet sweeps out a nearly triangular area (shaded in Figure 2-
Kepler’s Second Law: A line joining a planet and the Sun sweeps out equal areas in equal intervals of time.
39
A consequence of Kepler’s second law is that each planet’s speed decreases as it moves from perihelion to aphelion. The speed then increases as the planet moves from aphelion toward perihelion.
Kepler was also able to relate a planet’s year to its distance from the Sun. This discovery, published in 1619, is Kepler’s third law. This relationship predicts the planet’s sidereal period if we know the length of the semimajor axis of the planet’s orbit:
Kepler’s Third Law: The square of a planet’s sidereal period around the Sun is directly proportional to the cube of the length of its orbit’s semimajor axis.
Focus Question 2-5
What is the shape of the International Space Station’s orbit around Earth?
Focus Question 2-6
We saw in Chapter 1 that the Moon’s orbit around Earth is not circular. Where in its orbit is the Moon moving fastest, and where is it moving slowest?
The relationship is easiest to use if we let P represent the sidereal period of an object’s orbit around the Sun in Earth years and a represent the length of its semimajor axis (that is, its average distance from the Sun) measured in astronomical units (AU). One astronomical unit is the average distance from Earth to the Sun; hence, a = 1 for Earth. The astronomical unit is used when measuring distances between objects in the solar system, because no powers of ten are needed, as they would be if these distances were referred to in kilometers or miles. (See Discovery 2-2: Units of Astronomical Distance for more details.) Now we can write Kepler’s third law for all objects orbiting the Sun as
P2 = a3
 This equation says that a planet closer to the Sun has a shorter year than does a planet farther from the Sun. Using this equation with Kepler’s second law reveals that planets closer to the Sun move more rapidly than those farther away. Using data from Table 2-
This equation says that a planet closer to the Sun has a shorter year than does a planet farther from the Sun. Using this equation with Kepler’s second law reveals that planets closer to the Sun move more rapidly than those farther away. Using data from Table 2-
|
|
Sidereal period P (year) |
Semimajor axis a (AU) |
P2 |
= |
a3 |
|---|---|---|---|---|---|
|
Mercury |
0.24 |
0.39 |
0.06 |
|
0.06 |
|
Venus |
0.61 |
0.72 |
0.37 |
|
0.37 |
|
Earth |
1.00 |
1.00 |
1.00 |
|
1.00 |
|
Mars |
1.88 |
1.52 |
3.53 |
|
3.51 |
|
Jupiter |
11.86 |
5.20 |
140.7 |
|
140.6 |
|
Saturn |
29.46 |
9.54 |
867.9 |
|
868.3 |
|
Uranus |
84.01 |
19.19 |
7058 |
|
7067 |
|
Neptune |
164.79 |
30.06 |
27,160 |
|
27,160 |
40
UNITS OF ASTRONOMICAL DISTANCE
Throughout this book we will find that some of our traditional units of measure become cumbersome. It is fine to use kilometers to measure the diameters of craters on the Moon or the heights of volcanoes on Mars. However, it is as awkward to use kilometers to express the large distances to planets, stars, or galaxies as it is to talk about the distance from New York City to San Francisco or Sydney to Perth in millimeters. Astronomers have therefore devised new units of measure.
When discussing distances across the solar system, astronomers use a unit of length called the astronomical unit (AU), which is the average distance between Earth and the Sun:
1 AU ≈ 1.5 × 108 km ≈ 9.3 × 107 mi
Jupiter, for example, is an average of 5.2 times farther from the Sun than is Earth. Thus, the average distance between the Sun and Jupiter can be conveniently stated as 5.2 AU. This value can be converted into kilometers or miles using the previous relationship.
When talking about distances to the stars, astronomers choose between two different units of length. One is the light-
1 ly ≈ 9.46 × 1012 km ≈ 63,200 AU
The spaces between the planets, stars, and galaxies are nearly ideal vacuums. One light-
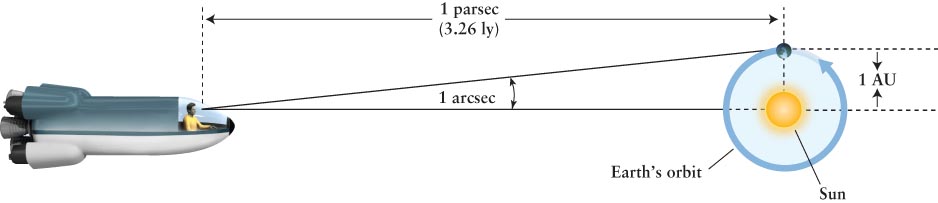
The second commonly used unit of length is the parsec (pc), the distance at which two objects separated by 1 AU make an angle of 1 arcsec (denoted 1″). Imagine taking a journey far into space, beyond the orbits of the outer planets. Watching the solar system as you move away, the angle between the Sun and Earth becomes smaller and smaller. When they are side by side from your perspective, and you measure the angle between them as 1/3600° = 1″, you have reached a distance that astronomers call 1 parsec, as shown in the accompanying figure. The parsec turns out to be longer than the light-
1 pc ≈ 3.09 × 1013 km ≈ 3.26 ly
Thus, the distance to the nearest star can be stated as 1.3 pc as well as 4.2 ly. Whether one uses light-
For larger distances, kilolight years (kly), megalight years (Mly), kiloparsecs (kpc), and megaparsecs (Mpc) are used. The prefixes kilo and mega simply mean “thousand” and “million,” respectively:

For example, the distance from Earth to the center of our Milky Way Galaxy is about 8.6 kpc, and the rich cluster of galaxies in the direction of the constellation Virgo is 20 Mpc away.
When Newton derived Kepler’s third law using the law of gravitation, discussed later in this chapter, he discovered that the mass of a planet affects the period of its orbit around the Sun. The mass of an object is a measure of the total number of particles of all different types that it contains and is expressed in units of kilograms. For example, the mass of the Sun is 2 × 1030 kg, the mass of a hydrogen atom is 1.7 × 10-27 kg, and the mass of the author of this book is 83 kg. At rest, the Sun, a hydrogen atom, and I have these same masses regardless of where we happen to be in the universe. It is important not to confuse the concept of mass with the concept of weight. Your weight is the force with which you push down on a scale due to the gravitational attraction of the world on which you stand.
41
However, the effect of the planet’s mass on the period of its orbit is exceedingly small for all the planets in the solar system, which is why the equation for Kepler’s third law, as shown in Table 2-
Kepler’s three laws apply not only to the planets orbiting the Sun but also to any object orbiting another under the influence of their mutual gravitational attraction. Thus, Kepler’s laws apply to moons orbiting planets, artificial satellites orbiting Earth, and even (with the above caveat) two stars revolving around each other.
2-6 Galileo’s discoveries strongly supported a heliocentric cosmology

While Kepler was in central Europe working on the laws of planetary orbits, an Italian physicist was making dramatic observations in southern Europe. Galileo Galilei did not invent the telescope, but he was one of the first people to point the new device toward the sky and publish his observations. He saw things that no one had ever imagined—
In 1610, Galileo also discovered four moons near Jupiter. Today, in honor of their discoverer, these are called the Galilean moons (or satellites, another term for moons). Galileo concluded that the moons orbit Jupiter because he saw them move in straight lines from one side of the planet to the other. (He did not see them move in elliptical orbits because from Earth we see their orbits from edge-

Galileo’s telescopic observations constituted the first fundamentally new astronomical data since humans began recording what they saw in the sky. In contradiction to then-
Insight Into Science
Theories and Explanations Scientific theories (or laws) based on observations can be useful for making predictions even if the reasons that these theories work are unknown. The explanation for Kepler’s laws came decades after Kepler deduced them, when Newton derived them in 1665 with his mathematical expression for gravitation, the force that holds the planets in their orbits.
42
A major stumbling block prevented seventeenth-
The scientific method clarified most of the issues surrounding planetary orbits, leading to the equations and laws developed by the brilliant and eccentric scientist Isaac Newton (for example, he believed in alchemy). He was born on Christmas Day in 1642, less than a year after Galileo died. In the decades that followed, Newton revolutionized science more profoundly than any person before him and, in doing so, he found physical and mathematical evidence in support of the heliocentric cosmology.
2-7 Newton formulated three laws that describe fundamental properties of physical reality
Until the mid-
Isaac Newton introduced a new approach. He began with three physical assumptions, now called Newton’s laws of motion, which led to equations that have since been tested and shown to be correct in many everyday situations. He also found a formula for the force of gravity (or gravitation), the attraction between all objects due to their masses. Putting the assumptions into mathematical form and combining them with the equation for gravity, Newton was able to derive Kepler’s three laws and use them to predict the orbits of bodies such as comets and other objects in the solar system. Newton also was able to use these same equations to predict the motions of bodies on and near Earth, such as the path of a projectile or the speed of a falling object.
Newton’s First Law—
If all of the external forces acting on an object do not cancel each other out, then there is a net external force acting on the object. Equivalently, we say that there is an unbalanced external force. For example, if you put a soccer ball between your hands and press on it so that it does not move, your hands represent a balanced pair of forces acting on the ball. In that case, you are exerting no net external force on the ball. Conversely, when your foot hits a soccer ball and the ball sails away, your foot has exerted a net external force on the ball.
At first, this law might seem to conflict with your everyday experience. For example, if you shove a chair, it does not move at a constant speed forever but comes to rest after sliding only a short distance. From Newton’s viewpoint, however, a “net external force” does indeed act on the moving chair—
43
Newton’s first law tells us why the planets keep moving in orbit around the Sun. First, they do not come to rest because there is virtually no air in space and hence no force from, for example, air friction opposing their motion. Second, they do not move in straight lines because there is an outside force acting on the planets to continually change their directions and keep them in orbit. As we shall see, that force is the Sun’s gravity.

Newton’s second law describes quantitatively how a force changes the motion of an object. To better appreciate the concepts of force and motion, we must first understand two related quantities: velocity and acceleration.
Imagine an object motionless in space. Push on it and it begins to move. At any moment, you can describe the object’s motion by specifying both its speed and direction. Speed and direction of motion together constitute an object’s velocity. If you continue to push on the object, its speed will increase—
Acceleration is the rate at which velocity changes with time. Because velocity involves both speed and direction, a slowing down, a speeding up, and a change in direction are all forms of acceleration.
Suppose, for example, an object revolved around the Sun in a perfectly circular orbit. As this object moved along its orbit, its speed would remain constant, but its direction of motion would be continuously changing. This body would have acceleration that involved only a change of direction. In general:
Newton’s Second Law—
In other words, the harder you push on something that can move, the faster it will accelerate. Also, an object of greater mass accelerates more slowly when acted on by a force than does an object of lesser mass acted on by the same force. That is why you can accelerate a child’s wagon faster than you can accelerate a car by pushing on them equally hard.
Newton’s second law can be succinctly stated as an equation. If a force acts on an object, the object will experience an acceleration such that
Force = mass × acceleration
 Force is usually expressed in pounds or newtons. For example, the force with which I am pressing down on the ground is 814 newtons (183 lb). But I weigh 814 newtons only on Earth. I would weigh 136 newtons (30.5 lb) on the Moon, which has less mass and so pulls me down with less gravitational force. Orbiting in the International Space Station, my weight (measured by standing on a scale in the space station) would be 0, but my mass would be the same as when I am on Earth. Whenever we describe the properties of planets, stars, or galaxies, we speak of their masses, never of their weights.
Force is usually expressed in pounds or newtons. For example, the force with which I am pressing down on the ground is 814 newtons (183 lb). But I weigh 814 newtons only on Earth. I would weigh 136 newtons (30.5 lb) on the Moon, which has less mass and so pulls me down with less gravitational force. Orbiting in the International Space Station, my weight (measured by standing on a scale in the space station) would be 0, but my mass would be the same as when I am on Earth. Whenever we describe the properties of planets, stars, or galaxies, we speak of their masses, never of their weights.
Newton’s final assumption, called Newton’s third law, is the law of action and reaction.
Newton’s Third Law—
For example, I weigh 183 lb on Earth, and so I press down on the floor with a force of 183 lb. Newton’s third law says that the floor is also pushing up against me with an equal force of 183 lb. (If it were less, I would fall through the floor, and if it were more, I would be lifted upward.) Even two objects that are not touching exert equal and opposite gravitational forces on each other. The Sun is exerting a force on each planet to keep it in orbit; hence, each planet exerts an equal and opposite force on the Sun. As each planet accelerates toward the Sun, the Sun in turn accelerates toward each planet.
Focus Question 2-7
Sitting in a moving car, how can you experimentally verify that your body has inertia?
Focus Question 2-8
How did people deduce that there is no air (and, hence, no air friction to slow planets down) in space before airplanes or even people-
Focus Question 2-9
If you are on a freely spinning merry-
Because the Sun is pulling on the planets, why don’t they fall onto it? Conservation of angular momentum provides the answer. Angular momentum is a measure of how much energy is stored in an object due to its rotation and revolution. The details of momentum are presented in Appendix P: Energy and Momentum. As the orbiting planets fall toward the Sun, their angular momentum provides them with motion perpendicular to that infall, meaning that the planets continually fall toward the Sun, but they continually miss it. Because their angular momentum is conserved, planets neither spiral into the Sun nor fly away from it. Angular momentum remains constant unless acted on by an external torque (also defined in Appendix P).
Angular momentum depends on three things: how fast an object rotates or revolves, how much mass it has, and how spread out that mass is. Consider, for example, a twirling ice skater. She rotates with a constant mass, practically free of outside forces. Because her angular momentum is therefore conserved, she can change how fast she is spinning by changing how spread out her mass is. According to conservation of angular momentum, as the spread of mass decreases, the rotation rate must increase. When she wishes to rotate more rapidly, she decreases the spread of her mass distribution by pulling her arms and outstretched leg in closer to her body (Figure 2-12). In astronomy, we encounter many instances of the same law, as giant objects, such as stars, contract.

44
We have now reconstructed the central relationships between matter and motion. Scientific explanation of the heliocentric cosmology still requires a force to hold the planets in orbit around the Sun and the moons in orbit around the planets. Newton identified that, too.
2-8 Newton’s description of gravity accounts for Kepler’s laws
Isaac Newton did not invent the idea of gravity. An observant seventeenth-
Newton succeeded in formulating a mathematical model that describes the behavior of the gravitational force that keeps the planets in their orbits (presented in Appendix R: Gravitational Force).
Newton’s Law of Universal Gravitation: Two objects attract each other with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
The inverse square part of this law means that gravitational force decreases with distance. Table 2-4 gives some examples of the inverse square law. Despite its weakening, the force of gravity from each object extends throughout the universe. Also, an object with twice the mass of another object exerts twice the gravitational force as the less massive object.
|
Distance between two objects |
The gravitational force between two objects compared to when they are a distance of 1 apart |
|---|---|
|
1 |
1 |
|
2 |
1/4 |
|
3 |
1/9 |
|
4 |
1/16 |
45
Using his law of gravity along with his three laws stated earlier, Newton found that he could mathematically explain Kepler’s three laws. For example, whereas Kepler discovered by trial and error that the period of orbit, P, and average distance between the Sun and planet, a, are related by P2 = a3, Newton mathematically derived this equation (corrected by including a tiny contribution due to the mass of the planet, as mentioned earlier). Bodies in elliptical orbits are bound by the force of gravity to remain in orbit.
 It seems plausible that astronauts floating in the International Space Station do not feel any force of gravity from Earth, but they do. Orbiting 330 km (approximately 200 mi) above Earth’s surface, they feel 90% as much gravitational force from the planet as we do standing on it. They are weightless, however, because as they fall earthward, their angular momentum carries them around the planet at just the right rate to continually miss it. The same effect occurs for all objects in orbit, as shown in the figure that opens this chapter.
It seems plausible that astronauts floating in the International Space Station do not feel any force of gravity from Earth, but they do. Orbiting 330 km (approximately 200 mi) above Earth’s surface, they feel 90% as much gravitational force from the planet as we do standing on it. They are weightless, however, because as they fall earthward, their angular momentum carries them around the planet at just the right rate to continually miss it. The same effect occurs for all objects in orbit, as shown in the figure that opens this chapter.
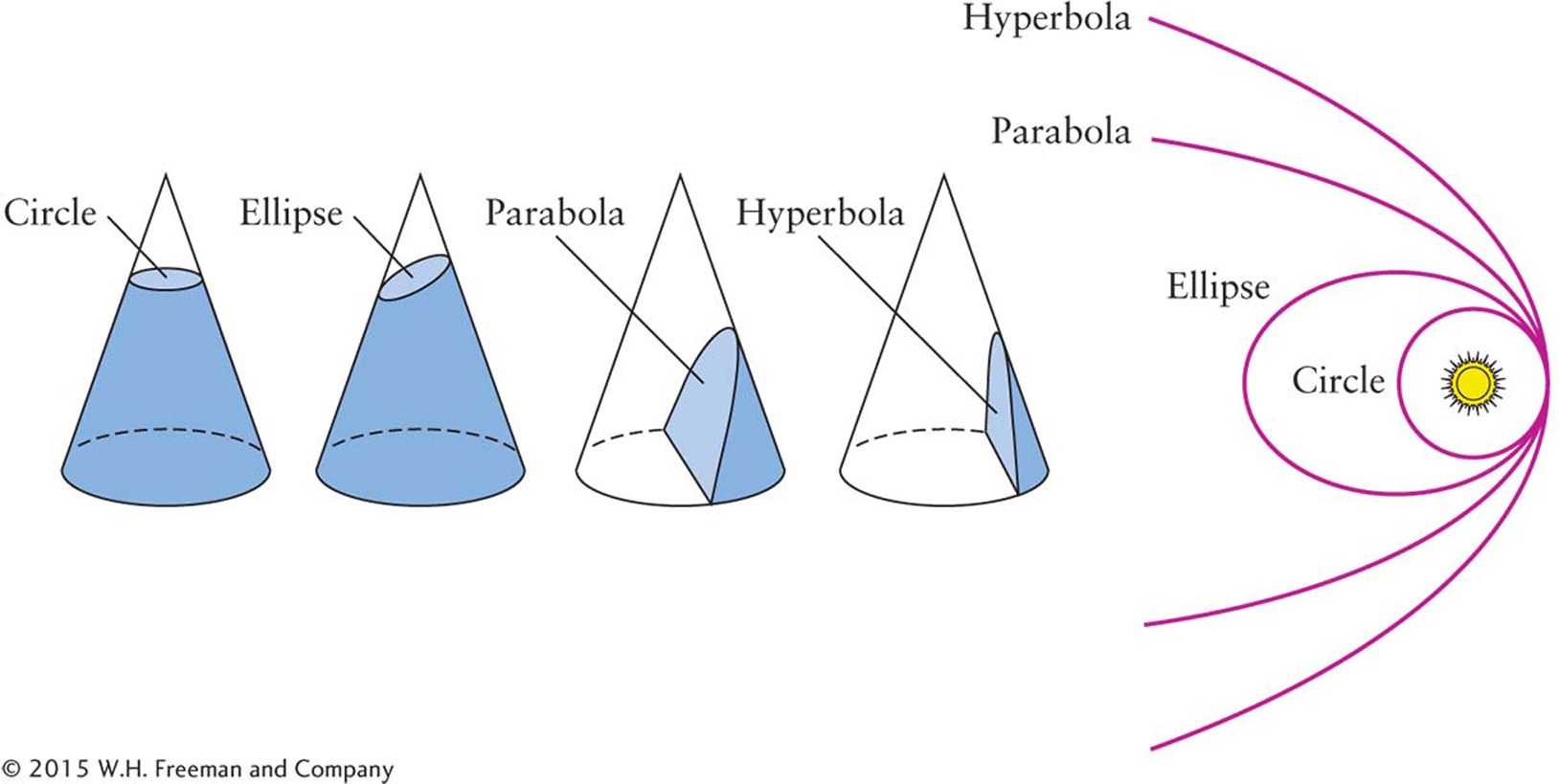
Newton also discovered that some objects orbiting the Sun can follow nonelliptical paths. His equations led him to conclude that orbits can also be parabolas or hyperbolas (Figure 2-13). In both cases, such bodies would make only one pass close to the Sun and then travel out of the solar system, never to return. To date, all of the objects observed in the solar system began their existence in elliptical orbits, but some comets (small bodies of rock and ice) have received enough energy from being pulled by planets or from expelling jets of gas to develop parabolic or hyperbolic orbits.

Using the equations Newton derived, the orbits of the planets and their satellites could be calculated with unprecedented precision. Using his laws, mathematicians showed that Earth’s axis of rotation must precess because of the gravitational pull of the Moon and the Sun on Earth’s equatorial bulge (recall Figure 1-
Perhaps the most dramatic early use of the scientific method with Newton’s ideas was its role in the discovery of the eighth planet in our solar system. The seventh planet, Uranus, had been discovered by William Hersch in 1781 during a systematic telescopic survey of the sky. Fifty years later, however, it was clear that Uranus was not following the orbit predicted by Newton’s laws. Two mathematicians, John Couch Adams in England and Urbain-
46
Insight Into Science
Quantify Predictions Mathematics provides a language that enables science to make quantitative predictions that can be checked by anyone. For example, in this chapter, we have seen how Kepler’s third law and Newton’s universal law of gravitation correctly predict the motion of objects under the influence of the Sun’s gravitational attraction.
It is a testament to Newton’s genius that his three laws were precisely the basic ideas needed to understand so much about the natural world. Newton’s process of deriving Kepler’s laws and the universal law of gravitation helped secure the scientific method as an invaluable tool in our process of understanding the universe. Figure 2-15 shows some of the effects of gravity at the scales of planets, stars, and galaxies.

 Jupiter and Its Largest Moons In 1610, Galileo discovered four “stars” that move back and forth across Jupiter. He concluded that they are four moons that orbit Jupiter just as our Moon orbits Earth. (a) Observations made by Jesuits in 1620 of Jupiter and its four visible moons. (b) Photograph of the four Galilean satellites alongside an overexposed image of Jupiter. Each satellite would be bright enough to be seen with the unaided eye were it not overwhelmed by the glare of Jupiter.
Jupiter and Its Largest Moons In 1610, Galileo discovered four “stars” that move back and forth across Jupiter. He concluded that they are four moons that orbit Jupiter just as our Moon orbits Earth. (a) Observations made by Jesuits in 1620 of Jupiter and its four visible moons. (b) Photograph of the four Galilean satellites alongside an overexposed image of Jupiter. Each satellite would be bright enough to be seen with the unaided eye were it not overwhelmed by the glare of Jupiter.