EXAMPLE 14 Performing a two-way ANOVA

cellsmartphone

Perform a two-way ANOVA for the cell phone and smartphone ratings data in Table 9, using level of significance . Assume the requirements are met.

Solution

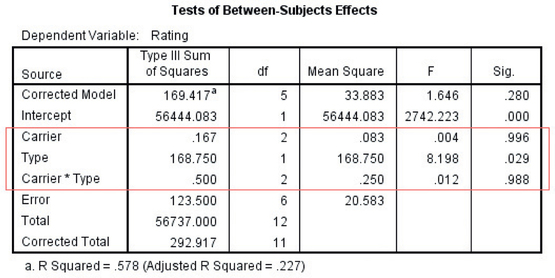
The Minitab results are provided in Figure 39, and the SPSS results are provided in Figure 40.

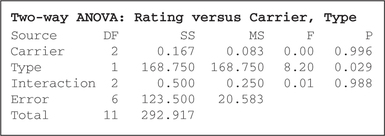
image
Figure 12.39: FIGURE 39 Minitab two-way ANOVA results for the phone ratings data.
image
Figure 12.40: FIGURE 40 SPSS two-way ANOVA results for the phone ratings data.

The null hypothesis in the test for interaction always states that there is no interaction between the factors.

  • Step 1 Test for interaction. The hypotheses are:

    Reject if the . The -value in the Interaction row in Figure 39 is 0.988, which is not ≤0.05; therefore, do not reject . There is insufficient evidence of interaction between carrier and type at level of significance . Therefore, we may proceed with the tests for the main effects.

    705

  • Step 2 Test for Factor A. The hypotheses are:

    Reject if the . According to the two-way ANOVA table in Figure 39:

    • -value in the Carrier (Factor A) row = 0.996, which is not ≤0.05

    Therefore, we do not reject . There is insufficient evidence for a carrier (Factor A) effect. Thus, we do not find a statistically significant difference in Consumer Reports ratings among the three carriers, AT&T, T-Mobile, and Verizon.

  • Step 3 Test for Factor B. The hypotheses are

    Reject if the . The -value in the type (Factor B) row in Figure 39 is 0.029, which is ≤0.05; therefore, reject . There is evidence for a type (Factor B) effect. Thus, we can conclude that there is significant difference in Consumer Reports ratings between the types: cell phones versus smartphones.

NOW YOU CAN DO

Exercises 17–20.