Example 22

EXAMPLE 22 Using rank correlation to detect a nonlinear pattern

scrabble

In the Chapter 13 Case Study, “How Fair Is the Scoring in Scrabble?” we noted from a scatterplot of Scrabble point values versus English-language letter frequencies that the relationship between the variables was not linear. Thus, we could not use a linear regression analysis. However, we can use rank correlation to test whether the two variables are associated, because linearity is not a condition for applying the rank correlation test.

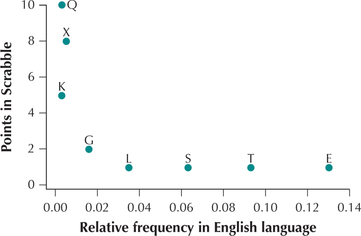
For the following random sample of letters, use the rank correlation test to investigate whether an association exists between English-language frequencies and Scrabble point values. Use level of significance $α = 0.05$ . Note from Figure 24 that the relationship between the variables is certainly nonlinear.

Page 14-49

Letter	Frequency	Scrabble points
Q	0.003	10
L	0.035	1
G	0.016	2
E	0.130	1
X	0.005	8
T	0.093	1
S	0.063	1
K	0.003	5

FIGURE 24 A linear relationship.

Solution

The data come from a random sample, so we may proceed with the hypothesis test.

Step 1 State the hypotheses.
- $H_{0} : No rank correlation exists between language frequency and Scrabble points.$
- $H_{α} : A rank correlation exists between language frequency and Scrabble points.$
Step 2 Find the critical value and state the rejection rule. There are $n = 8$ letters in the random sample, so we apply the small-sample case $(n \leq 30)$ . Using Appendix Table K, we select the column with level of significance $α = 0.05$ and the row with $n = 8$ . Our critical value is $r_{crit} = 0.738$ . We will reject $H_{0}$ if $r_{data} ≥ 0.738$ or if $r_{data} ≤ − 0.738$ .

Step 3 Find the value of the test statistic. See Table 17.

Table 14.78: Table 17 Table of calculations to find

$\sum d^{2}$

Letter	Frequency	Scrabble points	Frequency rank	Scrabble rank	Difference $d$	$d^{2}$
Q	0.003	10	1.5	8	−6.5	42.25
L	0.035	1	5	2.5	2.5	6.25
G	0.016	2	4	5	−1	1
E	0.130	1	8	2.5	5.5	30.25
X	0.005	8	3	7	−4	16
T	0.093	1	7	2.5	4.5	20.25
S	0.063	1	6	2.5	3.5	12.25
K	0.003	5	1.5	6	−4.5	20.25
						$\sum d^{2} = 148.5$

There are $n = 8$ letters in the sample, so the value of the test statistic is given by

$r_{data} = 1 - \frac{6 Σ d^{2}}{n (n^{2} - 1)} = 1 - \frac{6 (148.5)}{8 (63)} ≅ - 0.7679$

Step 4 State the conclusion and the interpretation. Because $- 0.7679 ≤ − 0.738$ , we reject $H_{0}$ . There is evidence for a rank correlation between the frequency of letters in the English language and the number of points each letter is worth in the game of Scrabble. Because $r_{data}$ is negative, the relationship is also negative. That is, high point values are associated with low frequency in English, and vice versa.