Example 24

EXAMPLE 24 Conducting the runs test for randomness

Test whether the sequence from Example 23 is random by conducting the runs test for randomness, using level of significance $α = 0.05$ .

Solution

We know that the data are time-ordered, and that each data value represents one of two distinct outcomes. We may thus proceed with the hypothesis test.

Step 1 State the hypotheses.
- $H_{0} : The sequence of data is random.$
- $H_{α} : The sequence of data is not random.$
Step 2 Find the critical values, and state the rejection rule. We have $n_{1} = 12$ females and $n_{2} = 8$ males, so the small-sample case applies ( $n_{1} ≤ 20$ and $n_{2} ≤ 20$ ). In Appendix Table L we find the row with $n_{1} = 12$ and the column with $n_{2} = 8$ , giving us the critical values $G_{crit, lower} = 6$ and $G_{crit, upper} = 16$ (see Figure 26). We will reject $H_{0}$ if $G_{data} ≤ 6$ or if $G_{data} ≥ 16$ .

Page 14-58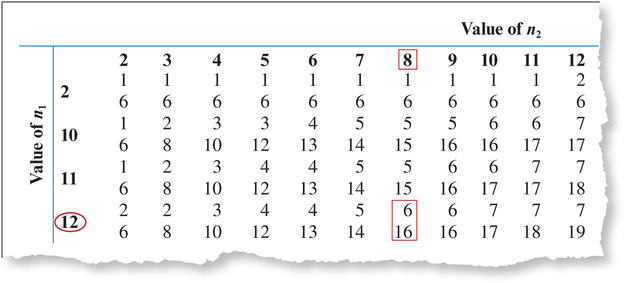

FIGURE 26 Finding the critical values for the runs test for randomness.
Step 3 Find the value of the test statistic. We have the small-sample case, so the test statistic $G_{data}$ is simply the number of runs, $G$ :
$G_{data} = G = 10$
Step 4 State the conclusion and the interpretation. Because $G_{data} = 10$ is not $\leq 6$ and is not $\geq 16$ , we do not reject $H_{0}$ . There is insufficient evidence that the sequence is not random.

NOW YOU CAN DO

Exercises 9–20.