EXAMPLE 35Find the values of that mark the boundaries of the middle 95% of the area
Find the two values of that mark the boundaries of the middle 95% of the area under the standard normal curve.
Solution
Note: Is it a coincidence that the two values of that determine the middle 95% of the area under the standard normal curve are 1.96 and 21.96? Not at all. The standard normal curve is symmetric about the mean 0, so the values −1.96 and 1.96 that form the boundaries of the middle 95% must be equidistant from zero.
- Step 1 Draw the standard normal curve, showing the desired middle area (95%) with boundaries labeled as and , as shown in Figure 41. By symmetry, there is in each tail.
- Step 2 Look up 0.025 on the inside of the table. Find by moving to the left and up from 0.025 in the table, giving us .
- Step 3 The area in the right tail is also 0.025, so the area to the left of is . Looking up 0.975 in the table gives us .
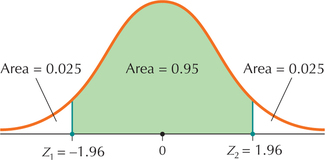
Figure 6.43: FIGURE 41 and mark the middle 95% of the distribution.
NOW YOU CAN DO
Exercises 87–90.