EXAMPLE 14 Constructing a confidence interval for

cerealsodium

Research has shown that the amount of sodium consumed in food has been associated with hypertension (high blood pressure). The table provides a list of 16 breakfast cereals, along with their sodium contents, in milligrams per serving.

  1. Determine whether the conditions are met for constructing a interval for the population mean sodium content per serving for all breakfast cereals.
  2. Find the value of for 99% confidence and degrees of freedom .
  3. Construct a 99% confidence interval for the population mean sodium content.
  4. Interpret the meaning of this confidence interval.
Cereal Sodium
(grams)		 Cereal Sodium
(grams)
Apple Jacks 125 Grape Nuts Flakes 140
Cap'n Crunch 220 Kix 260
Cinnamon Toast Crunch 210 Life 150
Corn Flakes 290 Lucky Charms 180
Count Chocula 180 Raisin Bran 210
Cream of Wheat 80 Rice Chex 240
Fruit Loops 125 Special K 230
Fruity Pebbles 135 Total Whole Grain 200

453

Solution

  1. Figure 17 contains the normal probability plot for the data set. Though not perfect, all points lie within the bounds, indicating acceptable normality. Thus, we proceed to construct the 99% confidence interval.
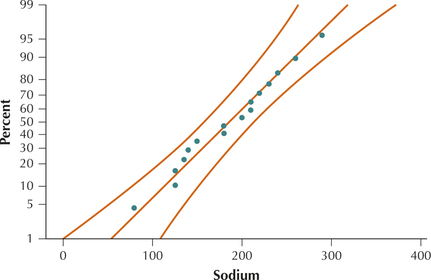    image
    Figure 8.17: FIGURE 17 Normal probability plot for sodium in cereal.
  2. The value of for 99% confidence and 15 degrees of freedom is 2.947.

  3. A 99% confidence interval for is given by the interval

    From the Minitab output in Figure 18, we have , , and . Substituting, we get:

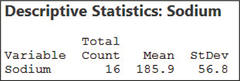    image
    Figure 8.18: FIGURE 18 Minitab ouput.
  4. We are 99% confident that , the population mean sodium content per serving of all breakfast cereals, lies between 144.1 grams and 227.7 grams.

NOW YOU CAN DO

Exercises 13–28.