EXAMPLE 11 Test for , critical-value method, two-tailed test

When the level of hemoglobin in the blood is too low, a person is anemic. Unusually high levels of hemoglobin are also undesirable and can be associated with dehydration. The optimal hemoglobin level is 13.8 grams per deciliter (g/dl). Suppose a random sample of women at a certain college showed a sample mean hemoglobin of , the population standard deviation of hemoglobin level is , and hemoglobin level is normally distributed. We are interested in testing whether the population mean hemoglobin level differs from 13.8 g/dl. Perform the appropriate hypothesis test, using level of significance .

Solution

We may use the test, because the population of hemoglobin levels is normally distributed, and the population standard deviation σ is known.

  • Step 1 State the hypotheses.

    The key words “differs from” indicate a two-tailed test, with . Thus, our hypotheses are

    where represents the population mean hemoglobin level.

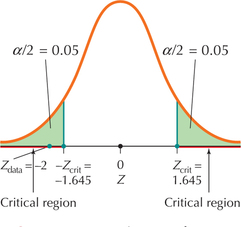    image
    Figure 9.5: FIGURE 5 Critical region for a two-tailed test.

  • Step 2 Find and state the rejection rule.

    We have a two-tailed test and level of significance . Using this information, Table 4 tells us that the critical value and that we will reject if or if (Figure 5).

  • Step 3 Calculate .

    We have , , , and . Substituting:

  • Step 4 State the conclusion and the interpretation.

    , which is ≤−1.645. Therefore we reject . There is evidence at level of significance that the population mean hemoglobin level differs from 13.8 g/dl.

NOW YOU CAN DO

Exercises 45–48.