Example 27

EXAMPLE 27 $Z$ test for $p$ using the $p$ -value method

We report $Z_{data}$ to two decimal places to allow the use of the $Z$ table to calculate the $p$ -value.

The National Transportation Safety Board publishes statistics on the number of automobile crashes that people in various age groups have. Young people ages 18–24 have an accident rate of 12%, meaning that on average 12 out of every 100 young drivers per year had an accident. A researcher claims that the population proportion of young drivers having accidents is greater than 12%. Her study examined 1000 young drivers ages 18–24 and found that 134 had an accident this year. Perform the appropriate hypothesis test using the $p$ -value method with level of significance $α = 0.05$ .

Solution

First, we check that both of our normality conditions are met. We are interested in whether the proportion has increased from 12%, so we have $p_{0} = 0.12$ .

$\begin{array}{l} n \cdot p_{0} = (1000) (0.12) = 120 \geq 5 & and & n \cdot q_{0} = (1000) (0.88) = 880 \geq 5 \end{array}$

The normality conditions are met and we may proceed with the hypothesis test.

Step 1 State the hypotheses and the rejection rule.

Our hypotheses are

$\begin{array}{l} H_{0} : p = 0.12 & versus & H_{a} : p > 0.12 \end{array}$

where $p$ represents the population proportion of young people ages 18–24 who had an accident. We reject the null hypothesis if the $p-value \leq α = 0.05$ .
Step 2 Calculate $Z_{data}$ .

Our sample proportion is $\hat{p} = 134 / 1000 = 0.134$ . Because $p_{0} = 0.12$ , the standard error of $\hat{p}$ is

$σ_{\hat{p}} = \sqrt{\frac{p_{0} \cdot q_{0}}{n}} = \sqrt{\frac{(0.12) (0.88)}{1000}} \approx 0.0103$

Thus, our test statistic is

$Z_{data} = \frac{\hat{p} - p_{0}}{\sqrt{\frac{p_{0} . q_{0}}{n}}} = \frac{0.134 - 0.12}{\sqrt{\frac{(0.12) (0.88)}{1000}}} \approx 1.36$

Page 548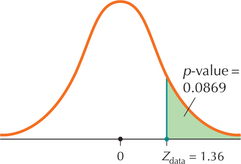

FIGURE 36 $p$ -Value for a right-tailed test equals area to right of $Z_{data}$ .

That is, the sample proportion $\hat{p} = 0.134$ lies approximately 1.36 standard errors above the hypothesized proportion $p_{0} = 0.12$ .
Step 3 Find the $p$ -value.

We have a right-tailed test, so our $p$ -value from Table 12 is $P (Z > Z_{data})$ . This is a Case 2 problem from Table 8 in Chapter 6 (page 355), where we find the tail area by subtracting the $Z$ table area from 1 (Figure 36):

$P (Z > Z_{data}) = P (Z > 1.36) = 1 - 0.9131 = 0.0869$
Step 4 State the conclusion and the interpretation.

The $p$ -value 0.0869 is not ≤ $α = 0.05$ , so we do not reject $H_{0}$ . There is insufficient evidence that the population proportion of young people ages 18–24 who had an accident has increased.

NOW YOU CAN DO

Exercises 19–22.