18 Growth and Form

18.3 18.2 How Much Is That in …?

We are interested in the limits of size, so we need to compare objects of different sizes, such as a gorilla with King Kong. However, it is not easy to compare objects measured in different units—say, the gorilla is measured in inches and pounds, but King Kong is measured in centimeters and kilograms. Consequently, we explore how to convert units from one measurement system to another.

We introduce two systems of units in which physical quantities are commonly measured and give a table of conversion factors and examples of how to convert from one system to the other.

U.S. Customary System

Table 18.1 lists units of the U.S. customary system of measurement and their abbreviations. Please note in the table the systematic way of converting from one unit to another and the use of scientific notation. The symbol ≈ means “is approximately equal to.”

Table 18.1: **TABLE 18.1** Units of the U.S. Customary System
Distance: $\begin{array}{l} 1 mile (mi) & = 1760 yards (yd) = 5280 feet (ft) \\ 1 yard (yd) & = 3 feet (ft) = 36 inches (in .) \\ 1 foot (ft) & = 12 inches (in .) \end{array}$
Area: $\begin{array}{l} 1 square mile & = 1 mi \times 1 mi = 5280 ft \times 5280 ft \approx 2 .8 \times 10^{7} {ft}^{2} \\ = 640 acres \\ 1 acre & = 43, 500 {ft}^{2} \end{array}$
Volume: $\begin{array}{l} 1 cubic mile & = 1 mi \times 1 mi \times 1 mi \\ = 5280 ft \times 5280 ft \times 5280 ft \approx 1 .5 \times 10^{11} {ft}^{3} \\ 1 U .S . gallon (gal) & = 4 U .S . quarts (qt) = 231 {in}^{3} . \end{array}$
Mass: $\begin{array}{l} 1 ton (t) & = 2000 pounds (lb) \end{array}$

Metric System

The world generally uses the metric system in science, industry, and commerce. It was first adopted in France in 1795. The fundamental unit of length, the meter (m), was originally 1/10,000,000 of the distance from the North Pole to the equator, along the meridian through Paris. The length is now defined as the distance that light travels in a vacuum in $\frac{1}{299, 792, 458}$ second. The second (s), in turn, is defined as the time that it takes an atom of the metal cesium to vibrate 9,192,631,770 times.

All other units of length, area, and volume are defined in terms of the meter. For example, a centimeter (cm) is one-hundredth of a meter.

Mass is the quantity of matter. The metric unit of mass, the kilogram (kg), is defined as the mass of a platinum-iridium standard kept in Paris. Since you can’t determine the mass of a sack of potatoes by comparing it to that, we measure the mass indirectly by seeing how much force gravity exerts on it—that is, we weigh it on a scale calibrated in pounds or kilograms. However, a mass of 1 kg would “weigh” (register on the scale) only one-sixth as much on the moon, because of the weaker gravity there.

Page 744

Table 18.2 lists the units of the metric system.

Table 18.2: **TABLE 18.2** Units of the Metric System
Distance: $\begin{array}{l} 1 meter (m) & = 100 centimeters (cm) \\ 1 kilometer (km) & = 1000 meters (m) \\ = 100, 000 centimeters (cm) = 1 \times 10^{5} cm \\ = 1, 000, 000 millimeters (mm) = 1 \times 10^{6} mm \end{array}$
Area: $\begin{array}{l} 1 {square meter (m}^{2}) & = 1 m \times 1 m \\ = 100 cm \times 100 cm = 10, 000 (cm 2) = 1 \times 10^{4} {cm}^{2} \\ 1 hectare (ha) & = 10, 000 m^{2} \end{array}$
Volume: $\begin{array}{l} 1 liter (L) & = 1000 {cm}^{3} = 0 {.001 m}^{3} \\ 1 cubic meter {(m}^{3}) & = 1 m \times 1 m \times 1 m \\ = 100 cm \times 100 cm \times 100 cm \\ = 1,000,000 {cm}^{3} = 1 \times 10^{6} {cm}^{3} (or cc) \end{array}$
Mass: $\begin{array}{l} 1 kilogram (kg) & = 1000 grams (g) = 1 \times 10^{3} g \\ 1 metric ton (tonne) & = 1000 kg \end{array}$

Converting Between Systems

The fundamental units of the U.S. customary system, the yard (for length) and the pound (for mass), are defined in terms of metric units, so that we have exactly

$\begin{matrix} 1 yd = 0.9144 m \\ 1 lb = 0.45359237 kg \end{matrix}$

Table 18.3 illustrates the conversion factors.

Most Internet search engines offer conversions; try entering, for example, “180 cm in ft.” You do not need to memorize conversion factors, but you will find it useful in life to memorize a few rough approximations:

$\begin{array}{l} 1 cm \approx 0.4 in & 1 m \approx 3 ft & 1 km \approx 0 .6 mi & 1 mi \approx 1 .6 km \\ 1 m^{2} \approx 10 {ft}^{2} & 1 L \approx 1 qt & 1 gal \approx 4 L & 1 kg \approx 0 .5 lb \end{array}$

Page 745

In the following examples, we explain how to convert measurements between systems. For such conversions, you may also find it useful to be acquainted with various prefixes used to denote multiples of units. From your experience with computers, you are no doubt familiar with the prefixes kilo-, mega-, and giga- (as in kilobytes, megabytes, gigabytes), indicating, respectively, the numbers $10^{3}$ , $10^{6}$ , and $10^{9}$ . Perhaps you are also familiar with tera- (hard drives are now sold that can contain several terabytes, where $1 terabyte = 1000 gigabytes = 10^{12}$ bytes of data). The corresponding scientific notation prefixes are k (thousand), M (million), G (billion), and T (trillion). What’s beyond that? P (peta-), E (exa-), Z (zetta-), and Y (yotta-) for $10^{15}, 10^{18}, 10^{21}$ , and $10^{24}$ , respectively. (A hint on proper usage: “Gazillion” is not a scientific unit prefix!) There are also corresponding prefixes for ever-smaller quantities: m (milli-), μ (micro-), n (nano-), and p (pico-) for $10^{- 3}, 10^{- 6}, 10^{- 9}$ , and $10^{- 12}$ .

Table 18.3: **TABLE 18.3** Conversions Between the U.S. Customary System and the Metric System
Distance: $\begin{array}{l} 1 in . & = 2 .54 cm \\ 1 ft & = 12 in . = 12 \times 2 .54 cm = 30 .48 cm = 0 .3048 m \approx 0 .3 m \\ 1 yd & = 0 .9144 m \approx 1 m \\ 1 mi & = 5280 ft = 5280 \times 30 .48 cm \\ = 160,934 .4 cm \approx 1 .609 km \approx 1 .6 km \\ 1 cm & \approx 1/2 .54 in . \approx 0 .4 in . \\ 1 cm & \approx 39.37 in . \approx 3 .281 ft \approx 3 ft \\ 1 km & \approx 0.621 mi \approx 0 .6 mi \end{array}$
Area: $\begin{array}{l} 1 {ft}^{2} & \approx 1000 {cm}^{2} \\ 1 m^{2} & \approx 10 {ft}^{2} \\ 1 hectare (ha) & \approx 2 .5 acres \end{array}$
Volume: $\begin{array}{l} 1 {ft}^{3} & \approx 30 liters (L) \\ 1 gallon & \approx 3 .785 liters (L) \approx 4 L \\ 1 cubic meter {(m}^{3}) & = 1000 liters \approx 264 U .S . gallon \approx 35 {ft}^{3} \\ 1 liter (L) & = 1000 {cm}^{3} \approx 1.06 U .S quarts (qt) \approx 0 .26 U .S . gallons \approx 1 qt \end{array}$
Mass: $\begin{array}{l} 1 lb & = 0 .45359237 kg \approx 0 .5 kg \\ 1 kg & \approx 2 .205 lb \approx 2 lb \end{array}$

EXAMPLE 2 What’s That in Feet?

An international student tells his American student friends that he is 180 cm tall. They ask, “How much is that in feet and inches?”

We approach this conversion by using the scaling factor $1 cm = \frac{1}{2.54}$ in:

$\begin{matrix} 180 cm = 180 \times 1 cm \\ = 180 \times \frac{1}{2.54} in . \\ \approx 70 .9 in . = 70.9 in . \times \frac{1 ft}{12 in .} = \frac{70.9}{12} ft \approx 5 .9 ft \end{matrix}$

However, because we normally give height in feet and a whole number of inches, the height is

$70.9 in . = 5 \times (12 in .) + 10.9 in .$

or approximately 5 ft 11 in.

Page 746

Another way to approach the problem is by means of a proportion:

$\frac{height in in .}{height in cm} = \frac{length of 1 in . in in .}{length of 1 in . in cm} = \frac{1 in .}{2 .54 cm}$

so that

$height in in . = height in cm \times \frac{1 in .}{2 .54 cm} = 180 cm \times \frac{1 in .}{2 .54 cm} \approx 70.9 in .$

where the cm in the numerator cancels with the cm in the denominator.

A strategy for a calculation requiring two or more conversions is first to plan the calculation: Write out an equation that involves just the units involved in the proportions, leaving out the numbers. Then, after checking that the units cancel correctly, insert the corresponding numbers and do the resulting arithmetic.

Self Check 2

Suppose that you are 5 ft 3 in. tall. How much is that in cm?

160 cm (rounded)

EXAMPLE 3 Got Gas?

Although in the United States we have traditionally measured the efficiency of cars in miles per gallon (mpg), the rest of the world measures it in liters per 100 km. The conversion between these two measures is more complicated than other conversions because the U.S. measure has distance (mi) in the numerator and quantity of fuel (gal) in the denominator, whereas the other measure has quantity of fuel (L) in the numerator and distance (km) in the denominator. We need to take this difference into account when doing the conversion.

For example, according to the Environmental Protection Agency (EPA), the most efficient gasoline vehicle of all time was the two-passenger 2000 Honda Insight, at 61 mpg on the highway. (This model was discontinued in 2006 due to poor sales!) What is the equivalent in liters per 100 km?

$\begin{matrix} 61 mpg = 61 \times \frac{1 mi}{1 gal} \\ = 61 \times \frac{1.609 k m}{3.785 L} \approx 25.93 \frac{k m}{L} \\ = \frac{25.93}{1} \times \frac{100 k m}{100 L} = \frac{1}{\frac{1}{25.93}} \times \frac{100 k m}{100 L} \\ \approx \frac{100 k m}{3 .9 L} \end{matrix}$

In other words, 100 km requires about 4 L. (Europeans would call such a car a “4-liter car.”) The key steps in the solution are to multiply both units by 100 and then divide both the numerator and the denominator of the fraction by 25.93, so as to get exactly 100 km in the numerator of the result.

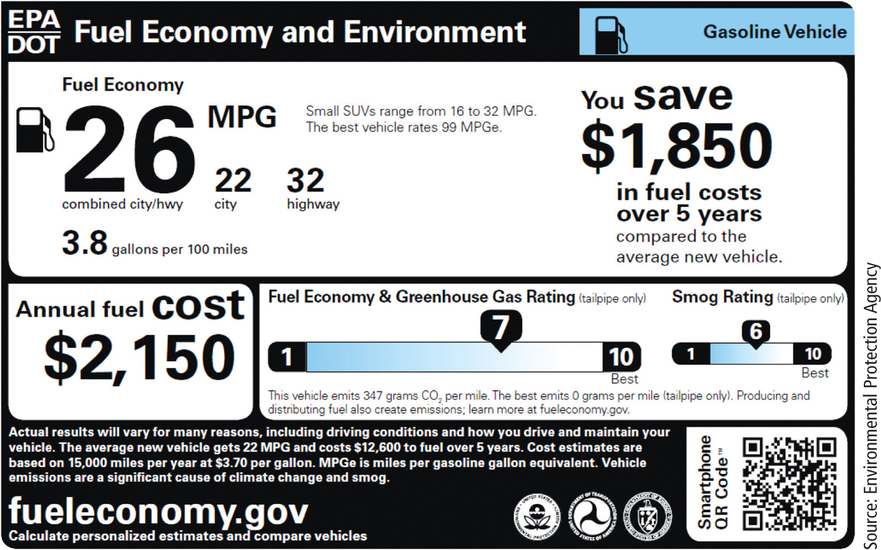
U.S. new-car labels, as shown in Figure 18.5, indicate fuel economy in terms of mpg but also—in much smaller print—gallons per 100 miles.

Self Check 3

The car indicated in Figure 18.5 uses 3.8 gallons per 100 miles. How many liters does it use for 100 km?

8.9 L/100 km

Page 747

Figure 18.5 Fuel-economy label for a 2015-model U.S. gasoline-powered car.