21.1 Simple Interest and Arithmetic Growth

1. In Example 2 on page 871, we considered a private student loan at 6.49%, but the rate for such a loan could have been as high as 12.99%. Under the same circumstances as in Example 1 ($10,000 principal, interest-only repayment), and at the higher rate, how much interest would you pay over 51 months of deferred payments on the principal?

2. Suppose that you need $30,000 for your last year of college. You could go to a private lending institution and apply for a signature student loan; rates range from 7% to 14%. However, your Aunt Sally is willing to lend you the money from her retirement savings, with no repayment until after graduation. All she asks is that in the meantime, you pay her each month the amount of interest that she would otherwise get on her savings (since she needs that to live on), which is 4%. What is your monthly payment to her, and how much interest will you pay her over the academic year (9 months)? (Aunt Sally will be glad to hear from you every month, anyway!)

3. In April 2014, you could buy a 10-year U.S. Treasury note (“T-note,” a kind of bond) for $10,000 that pays 2.726% simple interest every year through April 4, 2024. How much total interest would it earn by then?

4. In April 2014, you could buy a 30-year U.S. Treasury bond for $10,000 that pays 3.59% simple interest every year through April 2044. How much total interest would it earn by then?

5. In 2015, the concentration of the greenhouse gas carbon dioxide in Earth’s atmosphere reached 400 parts per million (ppm) by volume.

21.2 Compound Interest and Geometric Growth

6. You deposit $100,000 at 0.70% per year in a money market account. What is the interest at the end of one year if the interest paid is

7. Repeat Exercise 6, but for $100,000 at 1.2% per year.

8. In 2015, the concentration of the greenhouse gas carbon dioxide in the Earth’s atmosphere reached 400 parts per million (ppm) by volume. Although the concentration has been increasing at about 2 ppm per year in recent years, that annual increase itself has been steadily increasing, due to increased burning of fuels. The rate of increase of carbon dioxide in 2015 was about 0. 57% per year. If that trend continues from the 2015 level of 400 ppm, what will the concentration be when you retire, in, say, 2060?

9. Your Uncle Blake wants to help you get a start in life after college by setting aside $10,000 now—but you get it only after you finish college, three years from now. Since you are taking this course (and have an interest in what happens with the money in the meantime!), he asks for your advice about three fairly safe investment opportunities:

Which investment do you recommend to your uncle?

10. Suppose that you inherit $100,000 and invest it until you retire 40 years from now, in a fund that averages 6.5% annual earnings, which you reinvest.

11. The rule of 72 is a handy rule of thumb for estimating how long it takes money to double with annual compounding: If is the annual interest rate, expressed as a decimal, then the doubling time is approximately years. If you express the interest rate as , then the doubling time is approximately years.

12. If carbon dioxide in the atmosphere continues to increase at 0.57% per year, how long will it take for the concentration to double from 400 ppm in 2015?

13. An urban legend is that “the amount of information in the world doubles every three days.” Presumably the claim refers to the amount in bits, the smallest unit of storage in a computer. Show that the claim is absolutely preposterous by doing a little arithmetic and comparing your result with (the number of particles in the universe).

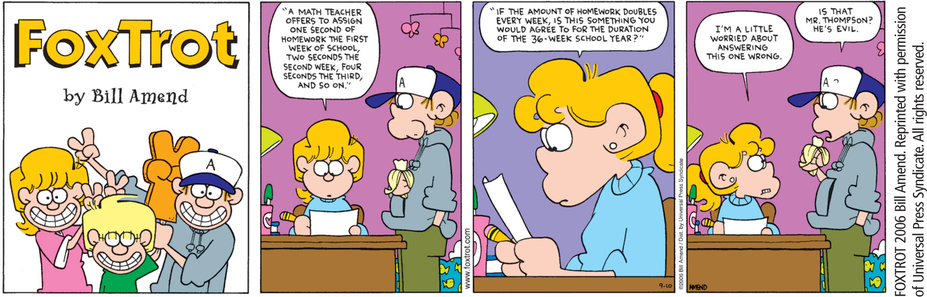
14. In a FoxTrot cartoon by Bill Amend (9/10/2006), shown below, Paige confronts a math problem in which “a math teacher assigns one second of homework the first week of school, two seconds the second week, four seconds the third, and so on.” She is asked whether she would agree to this weekly homework doubling for the duration of the 36-week school year. How much homework (in hours) would this plan require in week 36?

15. (Requires a spreadsheet) Write a spreadsheet program that reproduces Table 21.1 (page 873).

16. (Requires a spreadsheet) Write a spreadsheet program that reproduces Table 21.1 but rounds the interest added to the nearest cent.

17. (Requires a spreadsheet) Write a spreadsheet program that reproduces Table 21.2 (page 873).

18. (Requires a spreadsheet) Write a spreadsheet program that reproduces Table 21.2 but rounds to the nearest cent the interest added for yearly and quarterly compounding.

21.3 Effective Rate and APY

19. For the deposit in Exercise 6c, what is the APY?

20. For the deposit in Exercise 7b, what is the APY?

21. I had a CD with National City Bank through 2010 that paid 4.69% interest compounded daily. What was the APY for this rate?

22. First Community Credit Union of Beloit, Wisconsin, currently pays dividends at 0.10% per year, compounded monthly. What is the APY for such a rate?

23. U.S. savings bonds are sometimes used as patriotic presents or awards, since they help the government by loaning it money. The interest is exempt from state and local income taxes and from federal income tax if used to pay for college tuition and fees. In December 2013, the Rodel Exemplary Teacher Initiative, which addresses the shortage of effective teachers in Arizona’s neediest schools and encourages excellent teachers to stay in the profession, chose 11 teachers each to receive a U.S. savings bond. These bonds cost $5000 each and earn 0.10% annual interest for up to 30 years. Unlike many other bonds, the interest on these bonds is compounded semiannually. What will be the value of such a bond after 30 years?

24. A Series I savings bond earns a variable rate of interest that can change every six months, with compounding done semiannually. The initial rate was 1.48% in early 2015. If that rate continues unchanged for the 30 years of the bond’s duration, what will be the final accumulation on a $10,000 bond? What is the APY?

25. Suppose that on the statement for a money market account, the initial balance was $7744.70, the statement was for 34 days, the final balance was $7770.84, and there were no deposits or withdrawals. Calculate the APY.

26. Repeat Exercise 25, but for the preceding month, which had initial balance $7722.54, a period of 27 days, final balance $7744.70, and no deposits or withdrawals. Calculate the APY.

21.4 A Limit to Compounding

27. Use your calculator to evaluate for , 10, 100, 1000, and 1,000,000:

As gets larger, what number do these powers seem to be approaching?

28. Repeat Exercise 27 but for . Hint:

29. (Contributed by John Oprea of Cleveland State University.) Use your calculator to evaluate for :

As gets larger, what number do these powers seem to be approaching? Hint:

30. Repeat Exercise 27 but for . Hint: See the hints for Exercises 28 and 29.

31. Suppose that you have $1000 on deposit at your bank at an annual rate of 1.2%. How much interest do you receive after one year if the bank compounds

32. Suppose that you have a bank account with a balance of $4532.10 at the beginning of the year and $4632.10 at the end of the year. Your bank advertises “continuous compounding,” but in fact, it compounds continuously over each 24-hour day and posts interest to accounts daily.

33. Suppose that you have an investment that earns 0% in the first year, but 10% in the second year.

34. Suppose that you have an investment that earns 10% in the first year, 20% in the second year, and 30% in the third year.

35. Suppose that your house went down in value a total of 25% over the last three years but then will go up a total of 25% over the next three years. Will you end up with more than, less than, or the same as the original value?

A-43

36. Suppose that your friend’s house went up in value a total of 25% over three years but then went down a total of 25% over the succeeding three years. Did your friend’s house end up at more than, less than, or the same as the original value?

37. Calculate the doubling times for continuous compounding at 2%, 3%, and 4%, and compare them with those predicted by the rule of 72.

38. Calculate the doubling times for continuous compounding at 6%, 8%, and 9%, and compare them with those predicted by the rule of 72. What do you conclude? Why do you think people prefer the rule of 72 over a rule of 69.3?

21.5 A Model for Saving

39. Suppose that you want to save up $2000 for a semester abroad two years from now. How much do you have to put away each month in a savings account that earns 2% interest compounded monthly?

40. Repeat Exercise 39, except that you have found a better deal: 3% interest compounded monthly.

41. Parents may struggle for the first few years after a child is born but often are finally able to start saving toward the child’s college education when the child starts school at age 6 (because the parents stop paying for daycare). If they save $400 per month in an account paying 2.5% interest compounded monthly, how much will they have for college expenses 12 years later?

42. Suppose that you save for retirement by contributing the same amount each month from your 23rd birthday until your 65th birthday, in a retirement account that pays a steady 4% annual interest compounded monthly.

43. A colleague feels that he will need $1 million in savings to afford to retire at age 65 and still maintain his current standard of living. A younger colleague, age 30, decides to begin saving for retirement based on that advice. How much does the younger colleague need to save per month to have $1 million at retirement if the fund earns a steady 3% annual interest compounded monthly?

44. The younger colleague of Exercise 43 is not satisfied with a 3% return, which he could get with U.S. Treasury bonds or perhaps long-term CDs. Instead, he wants to take the riskier route of investing in the stock market, which has over its history returned an APY of about 10% per year (although between October 2007 and February 2009, it lost almost half its value). Assuming that over the 35 years until his retirement the stock market behaves just that way (a big assumption!), how much would he need to invest each month to achieve his goal of $2 million by age 65?

45. Many young people do not start saving right away for retirement, although by the time that they do, they may be earning more and thus could afford to save more each month. How much will be in your fund at age 65 if you don’t start saving until age 35 (by which time you hope interest rates will have risen) and at that age, start saving $100 per month in an account paying a steady 6% annual interest compounded monthly?

46. Suppose that you have children young, pay for their college expenses, and finally start saving for retirement at age 50. How much do you have to save per month, with a steady return of 6% compounded monthly, to accumulate $250,000 by age 65?

47. Suppose that when you turn 25, you are single and are in a 25% bracket for federal income tax and a 7% bracket for state and local income taxes. (In 2015 this corresponded to an income, beyond exemptions and deductions, of between $37,000 and $90,000.) This means that you pay an income tax rate (“marginal rate”) of 32% on part of your income (but a lower rate on the rest). Suppose that you commit to saving $100 per month toward retirement.

48. We continue the circumstances of Exercise 47. Instead of saving $100 per month—money on which you have already paid taxes, “after-tax” dollars—you have the alternative option offered in the tax code of participating in a tax-deferred retirement account (TDA), either through payroll deduction at work [e.g., as part of a 401(k) plan] or through an independent retirement account (traditional IRA). The money that goes into such a fund consists of “pre-tax” dollars: You do not pay tax on the money until you withdraw it (usually at retirement). Since you don’t pay income tax on the money as you put it in, you can actually put in more than $100 per month while reducing your take- home pay by only $100.

903

49. There is yet another alternative to the two options for saving toward retirement in Exercises 47 and 48. Instead of saving after-tax dollars or contributing to a tax-deferred plan, you can take the money as income, pay income tax on it, and make a deposit into a Roth IRA. For this special kind of retirement account, the interest earned over the years is not taxed. (There are further advantages and disadvantages.)

Suppose that you put $100 per month in after-tax dollars into a Roth IRA account. Assuming the same savings account or safe investment as in Exercises 47 and 48 that pays a steady return of 6% compounded monthly, how much will be in your account, tax-free, at age 65? How does that compare with the answers to Exercises 47a and 48c?

50. We continue the theme of Exercises 47–49 by comparing in algebraic terms three kinds of investments for retirement: an ordinary after-tax investment, a tax-deferred investment (such as a tax-deferred annuity or an IRA), and a Roth IRA. Let an investment earn interest at a steady annual yield and let your income (in whatever year you receive it) be taxed at rate .

51. Apart from CDs, returns on investments are rarely the same from year to year, since they vary with prevailing interest rates. How should you calculate an “average” rate of return over several years? Consider a mutual fund that delivers 100% return one year and loses 50% the next year. Calculate just the ordinary average (the arithmetic mean) of the percentages to get . That sounds good, but check what happens to a $1000 investment: It grows to $2000, then halves back to $1000—for a 0% gain. Because the average of the percentages is not useful, the customary way used in finance to calculate the “average” return is to use the geometric mean. If the initial value of the portfolio was , and its value after years is , then the average annual rate of return is the value of that solves , or .

52. Repeat Exercise 51a, but for consecutive returns over four years of , and 7%.

53. (Adapted from Terence Blows, Northern Arizona University.) Classify the following growth and decay scenarios as linear (arithmetic), exponential (geometric), or neither.

54. (Adapted from Terence Blows, Northern Arizona University.) Classify as in Exercise 53, but for the following circumstances.

55. What is the present value of $10,000, 4 years from now, at an APY of 5%?

56. What is the present value of $150,000, 10 years from now, at an APY of 3%?

57. As you will see in Chapter 22, if you had a 30-year $200,000 mortgage at 8% on a house or apartment, three-quarters of the way through the mortgage—after 22.5 years of payments—you would still owe half the amount, or about $100,000! You also would have paid about $300,000 in interest. (Current interest rates are much lower, but even at 4.75%, you would still owe $80,000 after 22.5 years.) What is the present value of $100,000, 22.5 years from now, at an interest rate of 8%? (If you put this much into a down payment, but made the same-size payments as for the 30-year mortgage on $200,000, you would own the house free and clear after 22.5 years.)

58. If you have a 30-year $200,000 mortgage at 6.48% on a house or apartment, after 10 years of payments you will still owe about $170,000. What is the present value of $170,000, 10 years from now, at an interest rate of 6.48%?

21.6 Inflation

59. Suppose that inflation proceeds at a constant rate of 2% per year from mid-2015 through mid-2018.

60. The Ford Mustang automobile celebrated its 50th anniversary in 2015. The original model cost about $2400 new in mid-1965. How much would that be in mid-2015 dollars? (The sticker price of the 2015 model year Mustang was $23,335.)

61. A first-semester college mathematics book cost $10.75 in 1962. What would the equivalent price be in 2015 dollars? How does that compare with what you paid to buy or rent this book? (That book had black-and-white text and figures and no photographs, color or otherwise.)

62. In 1970, before the oil embargo by the Organization of the Petroleum Exporting Countries (OPEC), gasoline cost about per gallon. In 1974, after the embargo, it cost about per gallon. What would the equivalent prices be in 2015 dollars? How do they compare with the price of gasoline today?

63. Find the average rate of inflation from 2005 to 2015. Is 3% a good approximation?

64. If inflation had been 3% each year from 2005 to 2015, what would the CPI have been in 2015?

65. (Based on a problem in Ed Barbeau’s “More Fallacies, Flaws, & Flimflam”; Washington, D.C., Mathematical Association of America, 2013, p. 2.) Suppose that you get a year-end pay raise of 5%, but over the year, there has been inflation of 10%—so in effect you have suffered a pay decrease in terms of what your salary will buy. What is the percentage decrease?

66. Suppose that you get a year-end pay raise of 5% but over the year there has been inflation of 2%. What is the percentage increase in your purchasing power?

67. According to state law introduced by Governor Scott Walker in 2011, teachers and state employees in Wisconsin can negotiate only salary increases and only up to the level of inflation. A typical elementary school teacher who began teaching in Wisconsin in 2015 at age 22 earned a salary of about $35,000. What would be the purchasing power in today’s dollars of her salary when she retires in 2055 at age 62 if each year her salary increase matches inflation?

68. (Spreadsheet helpful) Can a new assistant professor who starts now expect to make the equivalent of $90,000 in today’s dollars when she retires 35 years from now if inflation holds steady at 1.5%?

69. Repeat Exercise 68 but with inflation steady at 2.5%.

70. (Spreadsheet helpful) Suppose that you are the vice president for academic affairs at the liberal arts college. Suggest a salary policy that would result in the new assistant professor, when she retires in 35 years, making the equivalent of

71. (Spreadsheet helpful) Your roommate (a business major) has already planned her retirement and started funding it in 2015. She plans to retire in 2050 at age 57 on $100,000 per year in 2050 dollars, living on just the interest on her investments. Assume that she realizes a steady 7.2% interest rate and assume a steady 3% annual inflation. (Suggested by Terence Blows, Northern Arizona University.)

72. (Spreadsheet helpful) You think what your roommate means in Exercise 71 is that she wants to retire in 2050 with a steady income of $100,000 a year in 2015 dollars. You also feel that she should plan to receive that same value of income for 43 years in case she lives to 100 (2% of your classmates will). What is the present value in 2015 of the planned stream of 43 years of retirement income?

73. (Spreadsheet helpful) Suppose that you decide to lease a new car. (Leasing is cheaper than buying, because over the lease period you pay only about half the cost of buying the car.) At the end of a 48-month lease period, you either return the car or else make a lump-sum payment of $10,000 if you want to keep the car. You decide to save up, just in case you decide to keep the car; if you don’t keep this car, you will still have a down payment for a new leased or purchased car. You feel comfortable with saving $140/month (over and above your lease payments). How high an annual nominal interest rate on savings do you need to accumulate $10,000 in 48 months, with interest compounded monthly?

74. (Spreadsheet helpful) A 1990 advertisement read, “If you had put $100 per month into this fund starting in 1980, you’d have $37,747 today.” Assume that deposits were made on the last day of the month, starting in January 1980, through December 1989, and that interest was paid monthly on the last day of the month (120 months).

75. (Spreadsheet helpful) Suppose that your parents are willing to lend you $20,000 for part of the cost of your college education and living expenses. They want you to repay them the $20,000, without any interest, in a lump sum 15 years after you graduate, when they plan to retire and move. Meanwhile, you will be busy repaying federally guaranteed loans for the first 10 years after graduation. But you realize that you won’t be able to repay the lump sum without saving up. So you decide that you will put aside money in an interest-bearing account every month for the five years before the payment is due. You feel comfortable with putting aside $275 a month (the amount of the payment on your college loans, which will be paid off after 10 years).

How high an annual nominal interest rate on savings do you need to accumulate the $20,000 in 60 months, if interest is compounded monthly? Enter into a spreadsheet the values (annual rate), and , and the savings formula with replaced by (the monthly interest rate). You will find that the amount accumulated is not enough. Change to 0.09; it’s more than enough. Try other values until you determine to two decimal places.

Chapter Review

76. Tuition, room, and board cost $3400 per year at a certain Minnesota liberal arts college in 1970. What would be the equivalent cost in 2015 dollars?

77. Suppose that a 25-year-old who makes $40,000 a year gets a raise of 1% above the inflation rate each year, through age 65. What would be the purchasing power in today’s dollars of the final year’s salary?

78. What is the APY for an interest rate of 5% compounded daily?

79. Postage for a first-class letter cost $0.34 in 2001. If the cost had just kept up with inflation since then, what would it have been in 2015?

80. We noted that exponential growth is not necessarily fast growth. Confirm this fact for yourself by comparing the growth of $100,000 at 1% interest over 10 years for the two situations of