22.5 22.4 Other Loans
Discounted Loans
A federal student loan is in fact a hybrid loan. It is a combination of a conventional loan, in which the principal is amortized through monthly payments, and a discounted loan, in which in effect some of the interest is paid in advance as a “loan origination fee.”
A similar circumstance exists with mortgage loans for which at the time of purchase the borrower can pay “points” (a percentage of the house price) to obtain a lower interest rate.
Consequently, we explore discounted loans, as well as the related add-on loans.
Discounted Loan DEFINITION
A discounted loan is a loan in which the borrower receives the principal minus the interest but must pay back the entire principal, usually with equal payments.
924
For a discounted loan, the interest is computed as simple interest. Instead of getting the principal , the borrower gets the proceeds, the principal minus the interest: . However, the borrower must pay back the entire principal over the term of the loan; if there are payments, usually the amount of each payment is . (What is discounted is not the cost of the loan but how much the borrower gets!) The interest is based on the entire ; the borrower never has the use of that much, yet pays—up front—interest on the entire amount .
We first consider a simple imaginary situation to show how a discounted loan works. Suppose you need to borrow $7200 to buy a car. So you go to Joe’s Friendly Loan Service at a nearby off-campus location, and Joe offers you a discounted loan, at an interest rate of 5% per year (but he’s really friendly), with all of the interest to be paid up front and repayment of the principal to be in monthly installments over 3 years. The interest up front comes to . How much is your monthly payment? It’s just per month.
There’s just one (big) problem: With a discounted loan, Joe gives you the proceeds of the loan, which is $7200 minus the interest of $1080, or $6120. That leaves you $1080 short of the cost of the car!
Joe suggests that maybe you want to borrow a principal large enough so that the proceeds comes to $7200. How much should the amount of the loan be then? With the 15% interest subtracted, the proceeds are 85% of the principal . You need the proceeds to be , or . Then you get $7200, Joe gets a slightly bigger cut up front , and your payment is .
In general, for a principal , annual interest rate , and a term of years, the proceeds are .
Discounted Loan Formula RULE
For a principal loaned at annual interest rate for years, the monthly payment is
Being a law-abiding (mostly) loan shark, Joe knows that he is supposed to tell you the APR for your loan. To do that presents a problem: The APR is supposed to be the number of compounding periods per year times the rate of interest per compounding period. But Joe isn’t doing any compounding on your loan—except for the interest paid up front, all of your payments go toward paying back the principal.
Joe is confronting an instance of a larger problem: How do you compare different loans if up-front fees are involved?
925
We know how to compare some kinds of different loans for the same principal, if there are no additional fees involved:
- If the loans are over the same term, the loan with a lower interest rate is better for the borrower.
- If the loans are over different terms, as for a 15-year mortgage versus a 30-year mortgage, or a 48-month car loan versus a 72-month car loan, the borrower has to balance higher monthly payments for a shorter loan against paying more interest in the long run on a longer loan.
But for two loans with the same term, which of the following is less expensive?
- A loan with a 5% interest rate but 4% in fees up front
- A loan with a 4% interest rate but 5% in fees up front
The answer depends in part on the time value of money—how much more it is worth to you to pay a dollar at some time in the future compared with paying it today. That in turn may depend on the rate of inflation (see page 914) and on what you could gain by investing the money instead. In other words, it depends on the net present value of the stream of payments, given an investment interest rate and a rate for inflation. We cannot go into the details of such considerations here; that would be taken up in a course in mathematics of finance.
In fact, people tend to compare loans on the basis of the monthly payments rather than the interest rates. What we can do is take a loan with up-front fees and calculate the interest rate for an equivalent no-fee loan.
EXAMPLE 9 Equivalencing to a No-Fee Conventional Loan
In the case of Joe financing your car purchase, we can imagine that you are not borrowing $8470.59 and making an immediate payment of $1270.59, but simply borrowing $7200 and then repaying $7200 as a conventional loan at $235.29 per month over 36 months. The question is, what APR does that amortization correspond to?
We know , , and and need to solve the amortization payment formula for the annual interest rate , which is the APR:
That doesn’t look easy to do! And it isn’t—it cannot be done; it’s algebraically impossible to separate the out by itself. However, you can find the value of by putting the formula into a spreadsheet and trying successive approximations, or else by entering into the spreadsheet. (See Spotlight 21.3, page 888, for details about using a spreadsheet for financial calculations.) You find . Just don’t miss any payments.
Self Check 8
Joe’s competitor Sam has a counteroffer: a discounted loan at 5%, with proceeds of $7200, but repaid with monthly payments over 4 years (instead of 3 years). How much interest do you pay, what is the amount of your monthly payment, and what is the APR of the corresponding conventional loan?
- $1800 interest; $187.50 payment; 11.4% APR
926
EXAMPLE 10 Your Federal Student Loan
We can do a similar analysis for a federal student loan. For such a loan, in effect you pay 1.073% interest in advance as a “loan origination fee” and then have a conventional loan for the principal at a set interest rate of 4.29%. For simplicity, we assume that you make the monthly interest payments until your repayment starts.
So suppose you need $10,000. You need to borrow . To pay off the $10,108.46 loan over 120 months, your payment, either from the amortization payment formula or from , is $103.74.
Although you are paying back as if the loan were for $10,108.46, you got only $10,000. We can use a spreadsheet to calculate the APR for a corresponding no-fee loan as in Example 9 above via ) and arrive at 4.521%.
The effective annual rate (EAR) of the corresponding no-fee loan is
Self Check 9
Does the EAR depend on how much you borrowed?
- No
The situation with mortgages is similar. Banks often offer choices of mortgages with various combinations of interest rates and “points.” A point is 1% of the mortgage amount. You may “pay points” to “buy down” the interest rate for the mortgage to a lower rate. The cost of the points is paid up front to the bank at the closing of the house sale, so it is like interest on a discounted loan.
EXAMPLE 11 Paying Points
Suppose that you are offered a 30-year mortgage at 3.5% if you are willing to pay 2 points. What is the APR of the corresponding conventional loan, and what is its EAR?
The amount of the mortgage is not specified; in fact, to answer the question, we don’t need to know that. But to make the example concrete, suppose that the mortgage is for $100,000.
To have the full $100,000, you need to borrow $100,000/(1 - 0.02) — $102,041. To pay off that amount over 360 months at 3.5% annual interest, the payment can be found to be $458.21 from . We apply this payment to determine what interest rate it would correspond to in repaying a loan of only $100,00° by finding and we arrive at an APR of 3.663%. The corresponding EAR is
Self Check 10
Here is an extreme example: Suppose that you are offered a 30-year mortgage for $360,000 at 0% if you are willing to pay 50 points. You don’t have the 50 points (= $180,000), so you need to borrow a total of $540,000. What is the APR of the corresponding conventional loan, and what is its EAR?
- 2.91% APR; 2.95% EAR
927
In equivalencing a discounted loan to a no-fee loan, we are spreading some up-front fees over the term of the repayment. What is being equated between the two loans is the total amount of interest and fees that would be paid over the entire term of the loan. However, since the point of making payments over time is to make possible a large purchase for which you do not have enough money now, it does not make sense (as in Self Check 10) to pay a large amount of interest up front.
That is especially true if there is some likelihood of your paying the loan off early. For example, it does not pay to “pay points” if you expect to move in a few years and hence pay off the mortgage early.
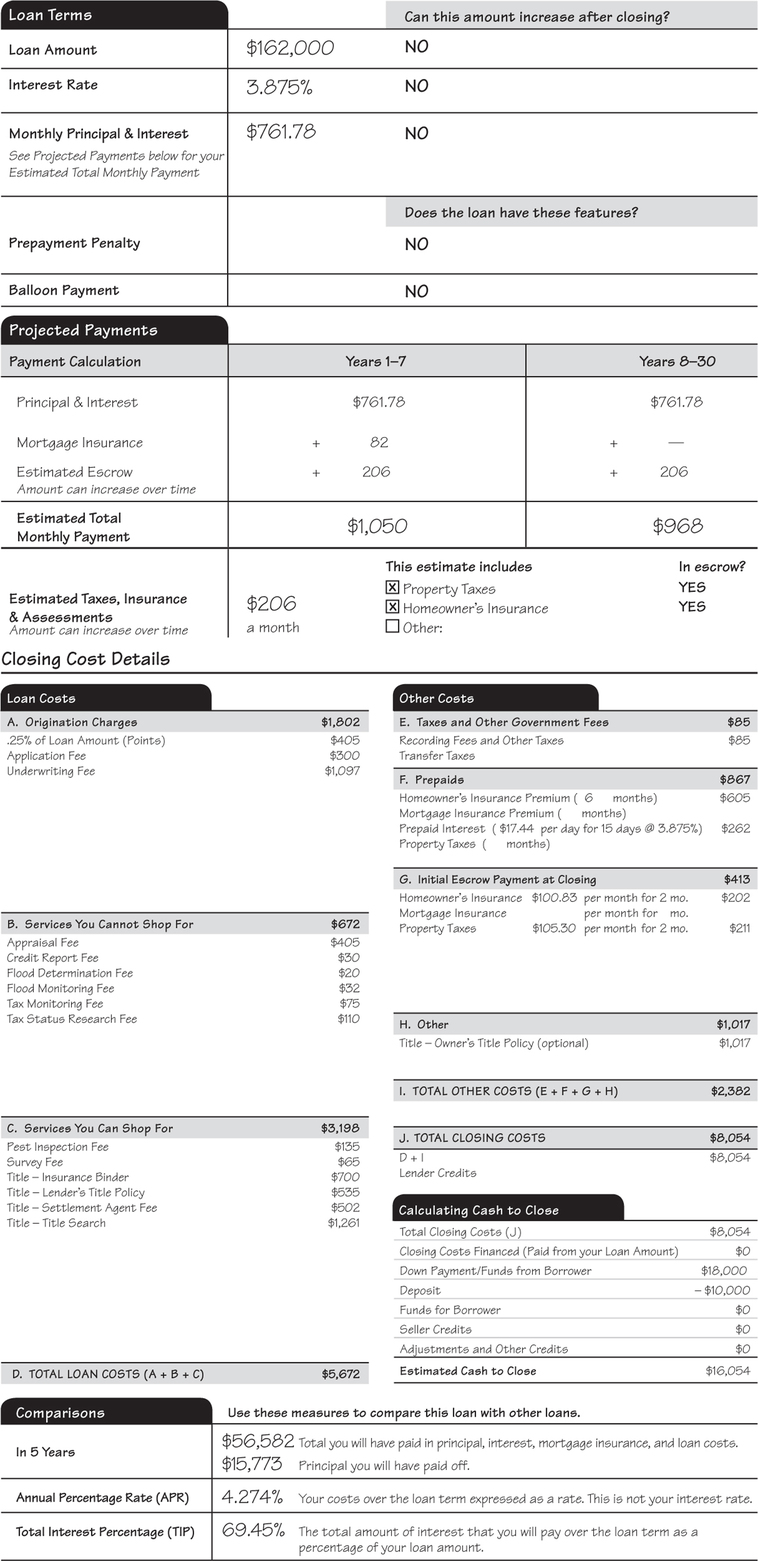
Figure 22.2 on the next page shows parts of a model mortgage loan estimate disclosure form created by the government’s Consumer Financial Protection Bureau, as directed by Congress.
The form includes new disclosure requirements effective from August 2015. Although the Bureau believes that including almost all of the up-front costs that the consumer must pay to get the loan as part of the “finance charge” (and the APR) would be more meaningful and beneficial for the consumer, it did not change policy to do so because of potential cost to the mortgage industry. Such a change would discourage lenders from shifting costs to fees that do not have to be declared as part of the finance charge. So the policy for the foreseeable future is “some fees in, some fees out.”
Add-on Loans
Another type of consumer loan is the add-on loan, which is often used for payday loans and sometimes for other purchases, such as furniture or a car. Such a loan has the great convenience of easy calculation of the interest and payments.
Add-on Loan DEFINITION
An add-on loan is a loan in which the borrower receives the principal, the interest is calculated in advance as simple interest, and the borrower pays back the principal plus interest in equal installments.
You borrow an amount to be repaid in years; the interest is simple interest at an annual rate , for a total of . You must pay in installments; with payments, each payment is
In effect, you pay th of the principal and th of the total interest with each payment. With monthly installments, we have and the formula becomes
Add-on Loan Formula RULE
For a principal loaned at annual interest rate for years, the monthly payment is
928
929
EXAMPLE 12 Add-on Loan
Suppose that you need to borrow $8000 to buy a used car. The dealer offers you a 5% add-on loan to be repaid in monthly installments over 4 years. This sounds like a much better deal than the 8% conventional loan that you can get at the credit union. How much is your payment on the dealer’s add-on loan?
The total amount to be paid over the 4 years (48 months) is
for a monthly payment of an even $200.00.
Self Check 11
What would be the monthly payment on the loan from the credit union at 8% over 48 months?
- $195.30
With an add-on loan, everything sounds simple and straightforward (especially the calculation). The interest is calculated on the entire principal; however, while you are slowly paying back the principal, you do not have the use of the whole amount for the entire loan period. (In fact, you have the use of the full principal for just one period!) You do indeed have the use of the car! But the net value that you have at any point is the value of the car minus the amount of principal already repaid (we neglect depreciation of the car). It is on this net value, and the amount of interest, that a “true” interest rate could be calculated.