Skills Check

Skill Check

Question 5.1

1. Here are the first rows of a dataset used for a student’s research project:

Student ID Gender Scholarship
Award		 High School
Percentile		 Residency First-year GPA
10256 Male 2000 64 Commuter 2.81
10260 Female 4000 72 On campus 3.62
10349 Female 2000 65 On campus 2.35
10388 Male 6000 85 On campus 3.76
10394 Female 6000 87 On campus 3.53
10422 Female 2000 59 On campus 3.01
10597 Male 4000 75 Commuter 3.26
10600 Male 4000 78 On campus 3.42

Describe the individuals in these data.

1.

The students (identified by Student ID)

Question 5.2

2. The number of variables in Skills Check 1 is _______. Classify each as quantitative or qualitative.

Figure 5.7 (page 193) is a histogram of the annual tuition and fee charges for 64 four-year universities/colleges in Massachusetts. Skills Checks 3, 4, and 5 are based on this histogram.

2.

5; gender and residency are qualitative; scholarship award, high school percentile, and first-year GPA are quantitative.

Question 5.3

3. The number of universities/colleges with tuition and fee charges covered by the leftmost bar in the histogram is _______.

3.

8

Question 5.4

4. What is the relative frequency for the interval covered by the leftmost bar?

4.

or 12.5%

Question 5.5

5. What percentage of universities/colleges had tuition and fees of at least $40,000?

5.

37.5%

222

Question 5.6

6. The histogram below represents the number of job applications completed by recent college graduates before securing a job. This distribution is best described as _______-skewed.

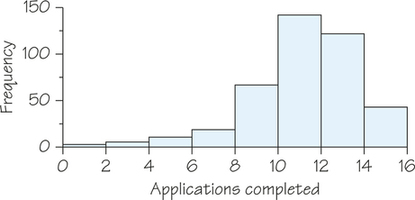
image

6.

left

Question 5.7

7. Brenner looks at real estate ads for houses in Sarasota, Florida. There are many houses ranging from $200,000 to $400,000 in price. A few houses on the coast, however, have prices of up to $15 million. The distribution of house prices will be

  1. skewed to the left.
  2. roughly symmetric.
  3. skewed to the right.

7.

c

Question 5.8

8. Here are foot lengths (in centimeters) of 15 randomly chosen soldiers:

27.2 28.0 26.2 25.7 32.8
25.0 26.3 28.0 27.4 25.8
26.9 26.8 27.3 29.0 28.8

In a stemplot of these measurements, the largest stem is _____.

8.

32

Question 5.9

9. For Figure 5.11 (page 195), interpret the meaning of 10|2.

  1. Ten states have a Hispanic population of 2%.
  2. Two states have a Hispanic population of 10%.
  3. One state has a Hispanic population of 10.2%.

9.

c

Question 5.10

10. The final (rightmost) digit of an observation would be called a ___________ in a stemplot.

10.

leaf

Question 5.11

11. For Figure 5.12 (page 195), what does the stem in 4|2 represent?

  1. 4
  2. 40
  3. 2

11.

b

Question 5.12

12. The mean foot length of the 15 soldiers in Skills Check 8 is ________.

12.

Question 5.13

13. The median foot length of the 15 soldiers in Skills Check 8 is _________.

13.

27.2 cm

Question 5.14

14. The mode of the foot length data in Skills Check 8 is _________.

14.

28.0 cm

Question 5.15

15. If a single-peaked distribution is skewed to the right, the median generally lies to the ___________ of the mean.

15.

left

Question 5.16

16. Between the first quartile and the third quartile lie ____% of the observations in a distribution.

16.

50%

Question 5.17

17. Which of these is greatest?

  1. The first quartile
  2. The third quartile
  3. The median

17.

b

Question 5.18

18. An outlier’s effect on the difference between the third quartile and the first quartile is _____ than its effect on the range.

18.

less

Question 5.19

19. In degrees Fahrenheit, a typical January day in Houston has a low of 46 and a high of 63, while in El Paso a typical January day has a low of 32 and high of 57. Which city has a larger temperature range for January?

  1. Houston, because and
  2. El Paso, because
  3. El Paso, because

19.

b

Question 5.20

20. The first quartile of the dataset {1, 2, 3, 4, 5, 6} is _______.

20.

2

Question 5.21

21. Which of these measures is not in a five-number summary?

  1. Median
  2. Minimum
  3. Mean

21.

c

Question 5.22

22. Determine the five-number summary of the 15 soldiers’ foot lengths in Skills Check 8. (Remember to list the five numbers in increasing order.)

22.

25.0, 26.2, 27.2, 28.0, 32.8

Question 5.23

23. The standard deviation of the 10 blood pressures in Self Check 4 (page 195) is _______. (Support your answer by showing the calculations.)

  1. 13.23
  2. 13.95
  3. 194.6

23.

b

Question 5.24

24. You have data on the weights (measured in grams) of five crackers. The correct units for the standard deviation of these weights are _________________.

24.

grams

223

Question 5.25

25. What are all the values that a standard deviation s can possibly take?

25.

a

Question 5.26

26. To specify the shape of a normal distribution completely, you must give its mean and its ___________________.

26.

standard deviation

Question 5.27

27. If two normal curves have the same mean but different standard deviations, the curve with the larger standard deviation will be ____________ the other curve.

  1. as tall as
  2. taller than
  3. shorter than

27.

c

Question 5.28

28. The steepest part of a normal curve is at the

  1. first and third quartiles.
  2. points at which the curvature changes.
  3. mean.

28.

b

Question 5.29

29. The scale of scores on an IQ test is approximately normal with a mean of 100 and a standard deviation of 15. The organization Mensa, which calls itself "the high-IQ society," requires an IQ score of 130 or higher for membership. What percentage of adults would qualify for membership?

  1. 95%
  2. 5%
  3. 2.5%

29.

c

Question 5.30

30. The length of human pregnancies from conception to birth varies according to a distribution that is approximately normal, with a mean of 266 days and a standard deviation of 16 days. We can expect that about _____% of all completed pregnancies are between 234 and 298 days.

30.

95%