5 Arbitrage and Interest Rates
Traders need to decide how to allocate their liquid cash balances between bank deposits that pay interest in different currencies. Suppose there are bank deposits that pay interest in dollars in the U.S. and in euros in Europe. Which is the best investment? Can traders make profits by shifting funds between them? How do these choices affect exchange rates? The answers are fundamental to the rest of the book.
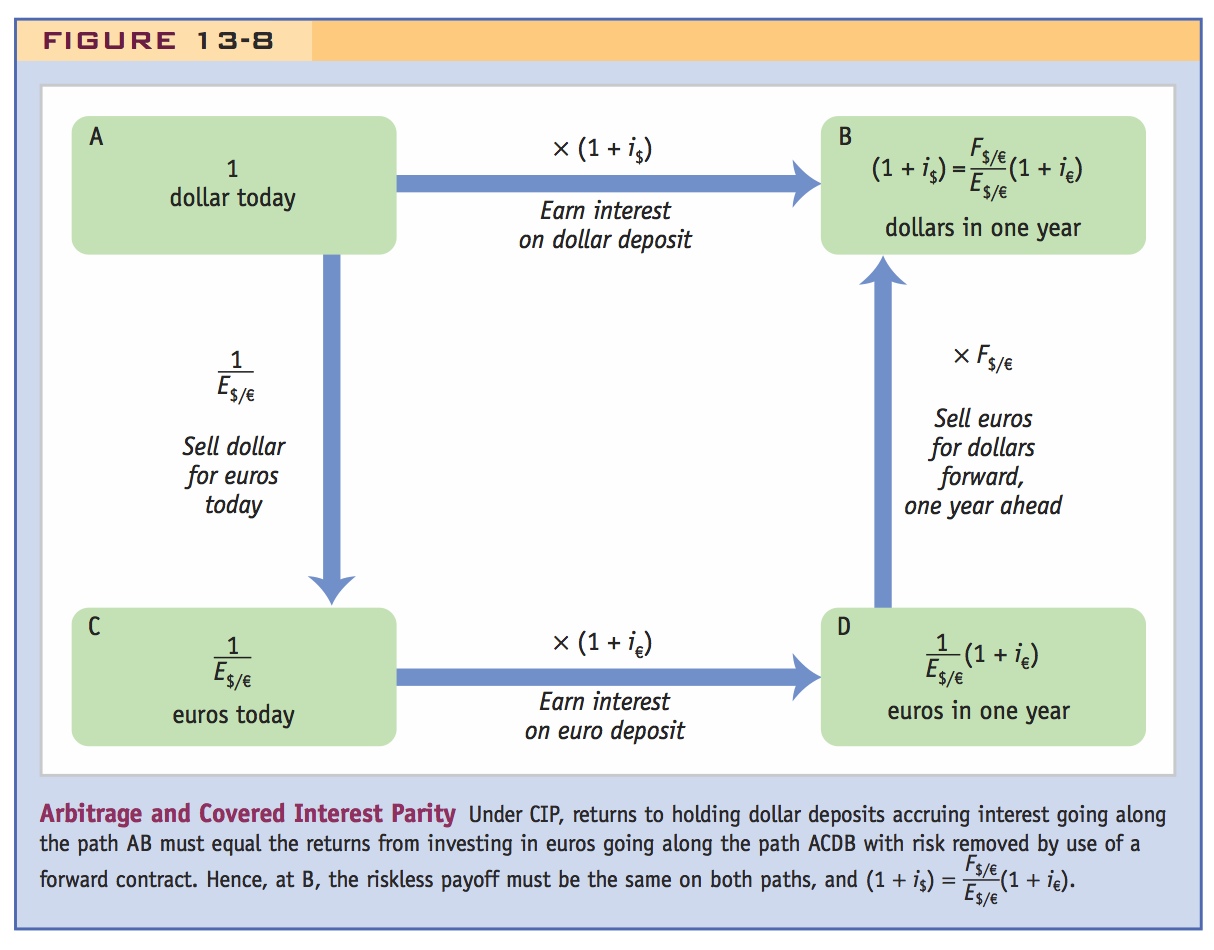
a. The Problem of Risk: If a trader repatriates the interest from a deposit denominated in foreign currency using the spot rate at maturity he or she will face exchange risk; this would constitute risky arbitrage. If he or she locks into a forward rate to bring home the interest at maturity, however, there is no risk; this is riskless arbitrage.
So far, our discussion of arbitrage has shown how actors in the forex market—for example, the banks—exploit profit opportunities if currencies trade at different prices. But this is not the only type of arbitrage activity affecting the forex market.
An important question for investors is in which currency they should hold their liquid cash balances. Their cash can be placed in bank deposit accounts denominated in various currencies where it will earn a modest interest rate. For example, a trader working for a major bank in New York could leave the bank’s cash in a euro deposit for one year earning a 2% euro interest rate or she could put the money in a U.S. dollar deposit for one year earning a 4% dollar interest rate. How can she decide which asset, the euro or the dollar deposit, is the best investment?
This is the final problem that we address in this chapter, and this analysis provides the tools we need to understand the forex market in the rest of this book. The analysis again centers on arbitrage. Would selling euro deposits and buying dollar deposits make a profit for the banker? Decisions like these drive the demand for dollars versus euros and the exchange rate between the two currencies.
The Problem of Risk A key issue for the trader is the exchange rate risk. The trader is in New York, and her bank cares about returns in U.S. dollars. The dollar deposit pays a known return, in dollars. But the euro deposit pays a return in euros, and one year from now we cannot know for sure what the dollar–euro exchange rate will be. Thus, how we analyze arbitrage in the sections that follow depends on how exchange rate risk is handled by the investor.
Reiterate that forward contracts obviate exchange risk.
As we know from our discussion of derivatives, an investor may elect to cover or hedge their exposure to exchange rate risk by using a forward contract, and their decision then simplifies to a case of riskless arbitrage. On the other hand, an investor may choose not to use a forward, and instead wait to use a spot contract when their investment matures, whereupon their decision is a case of risky arbitrage. These two ways of doing arbitrage lead to two important implications, called parity conditions, which describe equilibria in the forward and spot markets. We now examine each one in turn.
Riskless Arbitrage: Covered Interest Parity
1. Riskless Arbitrage: Covered Interest Parity
You have $1 to invest. Consider two investment strategies: (1) Invest the dollar in a U.S. deposit that pays 1 + i$ in dollars at maturity. (2) Trade the dollar for 1/E$/€ Euros; invest them at a deposit that pays 1 + i€ in euros at maturity and repatriate them at the forward rate F$/€. The forward rate provides forward cover from exchange risk. Argue that the no-arbitrage conditions requires the dollar return on dollar deposits to equal the dollar return on the euro deposit. This is covered interest parity (CIP), 1 + i$ = (1 + i€)F$/€/E$/€.
a. What Determines the Forward Rate? Traders use the CIP formula to calculate forward rates, given interest rates and current spot rates.
Suppose that contracts to exchange euros for dollars in one year’s time carry an exchange rate of F$/€ dollars per euro. This is known as the forward exchange rate and it allows investors to be absolutely sure of the price at which they can trade forex in the future.
Assume you are trading for the bank in New York, and you have to decide whether to invest $1 for one year in either a dollar or euro bank deposit that pays interest. The interest rate offered in New York on dollar deposits is i$ and in Europe the interest rate offered on euro deposits is i€. Which investment offers the higher return?
If you invest in a dollar deposit, your $1 placed in a U.S. bank account will be worth (1 + i$) dollars in one year’s time. The dollar value of principal and interest for the U.S. dollar bank deposit is called the dollar return. Note that we explicitly specify in what currency the return is measured, so that we may compare returns.
You may need to go through this reasoning a couple of times before they really understand where the dollar return on the euro deposit comes from.
If you invest in a euro deposit, you first need to convert the dollar to euros. Using the spot exchange rate, $1 buys 1/E$/€ euros today. These 1/E$/€ euros would be placed in a euro account earning i€, so in a year’s time they would be worth (1 + i€)/E$/€ euros. You would then convert the euros back into dollars, but you cannot know for sure what the future spot rate will be. To avoid that risk, you engage in a forward contract today to make the future transaction at a forward rate F$/€. The (1 + i€)/E$/€ euros you will have in one year’s time can then be exchanged for (1 + i€)F$/€/E$/€ dollars, the dollar value of principal and interest, or the dollar return on the euro bank deposit.10
48
Follow the same two-step procedure as with spot arbitrage when you explain this: (1) Imagine CIP doesn't hold, and ask them how they could make money. (2) Now, if everybody responds in the same way as you, what would happen to interest rates and the spot rate?
Three outcomes are possible when you compare the dollar returns from the two deposits. The U.S. deposit has a higher dollar return, the euro deposit has a higher dollar return, or both deposits have the same dollar return. In the first case, you would advise your bank to sell its euro deposits and buy dollar deposits; in the second case, you would advise the bank to sell its dollar deposits and buy euro deposits. Only in the third case is there no expected profit from arbitrage, so the corresponding no-arbitrage condition can be written as follows:
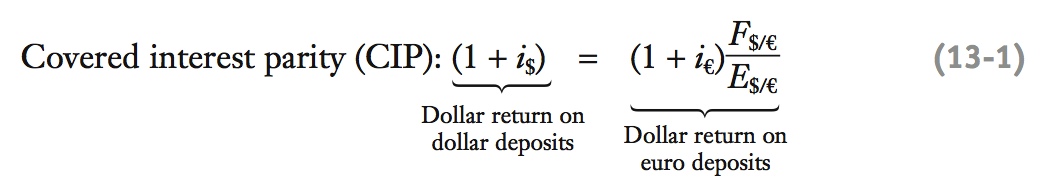
This expression is called covered interest parity (CIP) because all exchange rate risk on the euro side has been “covered” by use of the forward contract. We say that such a trade employs forward cover. The condition is illustrated in Figure 13-8.
What Determines the Forward Rate? Covered interest parity is a no-arbitrage condition that describes an equilibrium in which investors are indifferent between the returns on interest-bearing bank deposits in two currencies and exchange risk has been eliminated by the use of a forward contract. Because one of the returns depends on the forward rate, covered interest parity can be seen as providing us with a theory of what determines the forward exchange rate. We can rearrange the above equation and solve for the forward rate:
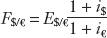
Thus, if covered interest parity holds, we can calculate the forward rate if we know all three right-hand side variables: the spot rate E$/€, the dollar interest rate i$, and the euro interest rate i€. For example, suppose the euro interest rate is 3%, the dollar interest rate is 5%, and the spot rate is $1.30 per euro. Then the preceding equation says the forward rate would be 1.30 × (1.05)/(1.03) = $1.3252 per euro.
This is worth emphasizing. CIP holds almost exactly (allowing for transaction costs and capital controls) and actually use this formula to set forward rates.
In practice, this is exactly how the forex market works and how the price of a forward contract is set. Traders at their computers all around the world can see the interest rates on bank deposits in each currency, and the spot exchange rate. We can now also see why the forward contract is called a “derivative” contract: to establish the price of the forward contract (the forward rate F), we first need to know the price of the spot contract (the spot rate E). That is, the pricing of the forward contract is derived from the pricing of the underlying spot contract, using additional information on interest rates.
This result raises a new question: How are the interest rates and the spot rate determined? We return to that question in a moment, after looking at some evidence to verify that covered interest parity does indeed hold.
49
Example of the pound/marks rate 1970-1994. After financial liberalization CIP holds almost exactly.
Evidence on Covered Interest Parity
Does covered interest parity hold? We expect returns to be equalized only if arbitrage is possible. But if governments impose capital controls, there is no way for traders to exploit profit opportunities and no reason for the returns on different currencies to equalize. Historical examples of such policies can provide illustration.
For example, Figure 13-9 shows that covered interest parity held for the United Kingdom and Germany after the two countries abolished their capital controls in the period from 1979 to 1981. (The German deposits shown here were denominated in marks prior to 1999; after 1999, the euro replaced the mark as the German currency.)
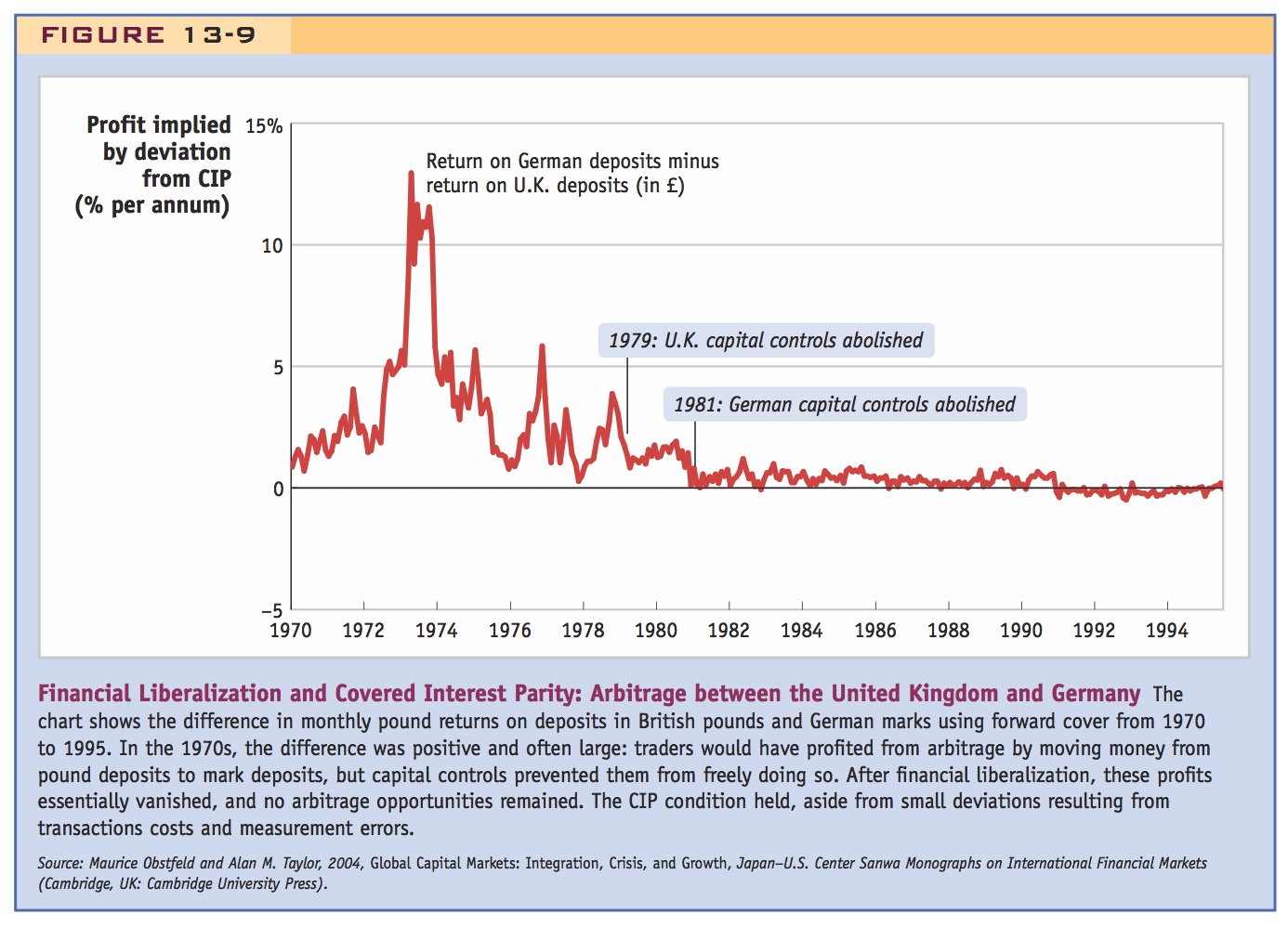
The chart shows the profit that could have been made (measured in percent per annum in British currency, before transaction costs) if the investor had been able to move funds from the United Kingdom to Germany with forward cover (or, when the line is in negative territory, the profit from moving funds from Germany to the United Kingdom). From Equation (2-1), we know that the profit from this riskless arbitrage would be
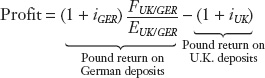
50
This profit would be zero only if covered interest parity held. In the 1960s and 1970s, the hypothetical profits implied by this expression were large—or would have been had arbitrage been allowed. Instead, capital controls in both countries prevented arbitrage. Covered interest parity therefore failed to hold. Following the financial liberalization from 1979 to 1981, arbitrage became possible. From that time until the present, profits have been essentially zero (not exactly zero because of regulations, fees, other transaction costs, and measurement error). Once we allow for these factors, there are no profit opportunities left. Covered interest parity holds when capital markets are open, and, using similar calculations, this can be confirmed for all freely traded currencies today.
This is a great graph to use, since it emphasizes both how well CIP works and the importance of capital mobility in permitting arbitrage.
Risky Arbitrage: Uncovered Interest Parity
1. Risky Arbitrage: Uncovered Interest Parity
Initially, ignore risk. Same argument as above but expect to repatriate the earnings at the expected future spot rate, Ee$/€. Argue that the no-arbitrage condition i 1 + i$ $ (1 + i€)Ee$/€/E$/€. This is uncovered interest parity (UIP).
As we noted above, the second way to engage in arbitrage is to use spot contracts, and accept that the future exchange rate is then subject to risk. We now examine this case, and by doing so, we will arrive at an understanding of how the exchange rate is determined in the spot market.
Say specifically that we are either assuming away risk, or assuming risk neutrality.
To keep things simple, let us suppose, for now, that investors focus exclusively on the expected dollar return of the two bank deposits and not on any other characteristics of the investment. (See Side Bar: Assets and Their Attributes.) Imagine you are once again trading for a bank in New York, and you must decide whether to invest $1 for one year in a dollar or euro bank deposit that pays interest. This time, however, you use spot contracts only and make no use of the forward contract to hedge against the riskiness of the future exchange rate.
51
The $1 invested in a dollar deposit will be worth (1 + i$) in one year’s time; this is the dollar return, as before.
Reassure the students that we are using exactly the same reasoning as for CIP, except that we have replaced the forward rate with the expected future spot rate.
If you invest in a euro deposit, a dollar buys 1/E$/€ euros today. With interest, these will be worth (1 + i€)/E$/€ euros in one year. At that time, you will convert the euros back into dollars using a spot contract at the exchange rate that will prevail in one year’s time. In this case, traders like you face exchange rate risk and must make a forecast of the future spot rate. We refer to the forecast as 
, which we call the expected exchange rate. Based on the forecast, you expect that the (1 + i€)/E$/€ euros you will have in one year’s time will be worth 
when converted into dollars; this is the expected dollar return on euro deposits, that is, the expected dollar value of principal and interest for euro deposits.
Provides a primer on assets, rates of return, risk, and liquidity.
a. What Determines the Spot Rate?Just as CIP could be used to calculate the forward rate, UIP can be used to calculate the current spot rate, given interest rates and expectations. This is the beginning of a theory of exchange rate determination. The next two chapters will fill in the missing pieces by explaining what determines interest rates and the expected future exchange rate.
Assets and Their Attributes
The bank deposits traded in the forex market pay interest and are part of the wider portfolio of assets held by banks and other private actors. As we have argued, the forex market is heavily influenced by the demand for these deposits as assets.
An investor’s entire portfolio of assets may include stocks, bonds, real estate, art, bank deposits in various currencies, and so on. What influences the demand for all these different kinds of assets? Viewed from a financial viewpoint (i.e., setting aside the beauty of a painting or seaside mansion), all assets have three key attributes that influence demand: return, risk, and liquidity.
An asset’s rate of return is the total net increase in wealth (measured in a given currency) resulting from holding the asset for a specified period, typically one year. For example, you start the year by buying one share of DotBomb Inc., a hot Internet stock, for $100. At year’s end, the share is worth $150 and has paid you a dividend of $5. Your total return is $55: a $50 capital gain from the change in the stock price plus a $5 dividend. Your total annual rate of return is 55/100, or 55%. The next year, the stock falls from $150 to $75 and pays no dividend. You lose half of your money in the second year: your rate of return for that year equals −75/150, or −50%. All else equal, investors prefer investments with high returns.
The risk of an asset refers to the volatility of its rate of return. The liquidity of an asset refers to the ease and speed with which it can be liquidated, or sold. A stock may seem to have high risk because its rate of return bounces up and down a lot, but its risk must be considered in relation to the riskiness of other investments. Its degree of risk could be contrasted with the rate of interest your bank offers on a money market deposit, a return that is usually very stable over time. You will lose your bank deposit only if your bank fails, which is unlikely. Your bank deposit is also very liquid. You can go to a cash machine or write a check to instantly access that form of wealth. In contrast, a work of art, say, is much less liquid. To sell the art for the greatest amount, you usually need the services of an auctioneer. Art is also risky. Works by different artists go in and out of fashion. All else equal, investors prefer assets with low risk and high liquidity.
This discussion of an asset’s attributes allows us to make two observations. First, because all else is never equal, investors are willing to trade off among these attributes. You may be willing to hold a relatively risky and illiquid asset if you expect it will pay a relatively high return. Second, what you expect matters. Most investments, like stocks or art, do not have a fixed, predictable, guaranteed rate of return. As a result, all investors have to forecast. We refer to the forecast of the rate of return as the expected rate of return.
52
Again, three outcomes are possible: the U.S. deposit has a higher expected dollar return, the euro deposit has a higher expected dollar return, or both deposits have the same expected dollar return.
Go through exactly the same two-step arbitrage argument as before.
We have assumed that traders like you are indifferent to risk and care only about expected returns. Thus, in the first two cases, you have expected profit opportunities and risky arbitrage is possible: you would sell the deposit with the low expected return and buy the deposit with the higher expected return. Only in the third case is there no expected profit from arbitrage. This no-arbitrage condition can be written as follows:
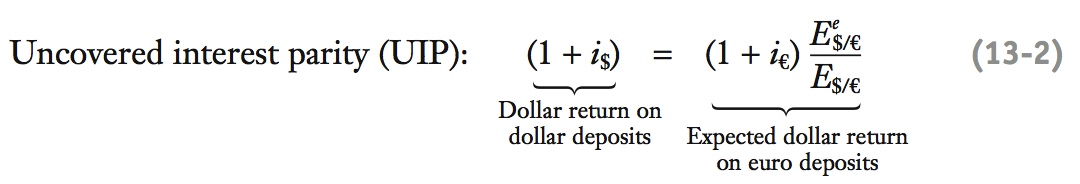
This expression is called uncovered interest parity (UIP) because exchange rate risk has been left “uncovered” by the decision not to hedge against exchange rate risk by using a forward contract and instead simply wait to use a spot contract in a year’s time. The condition is illustrated in Figure 13-10.
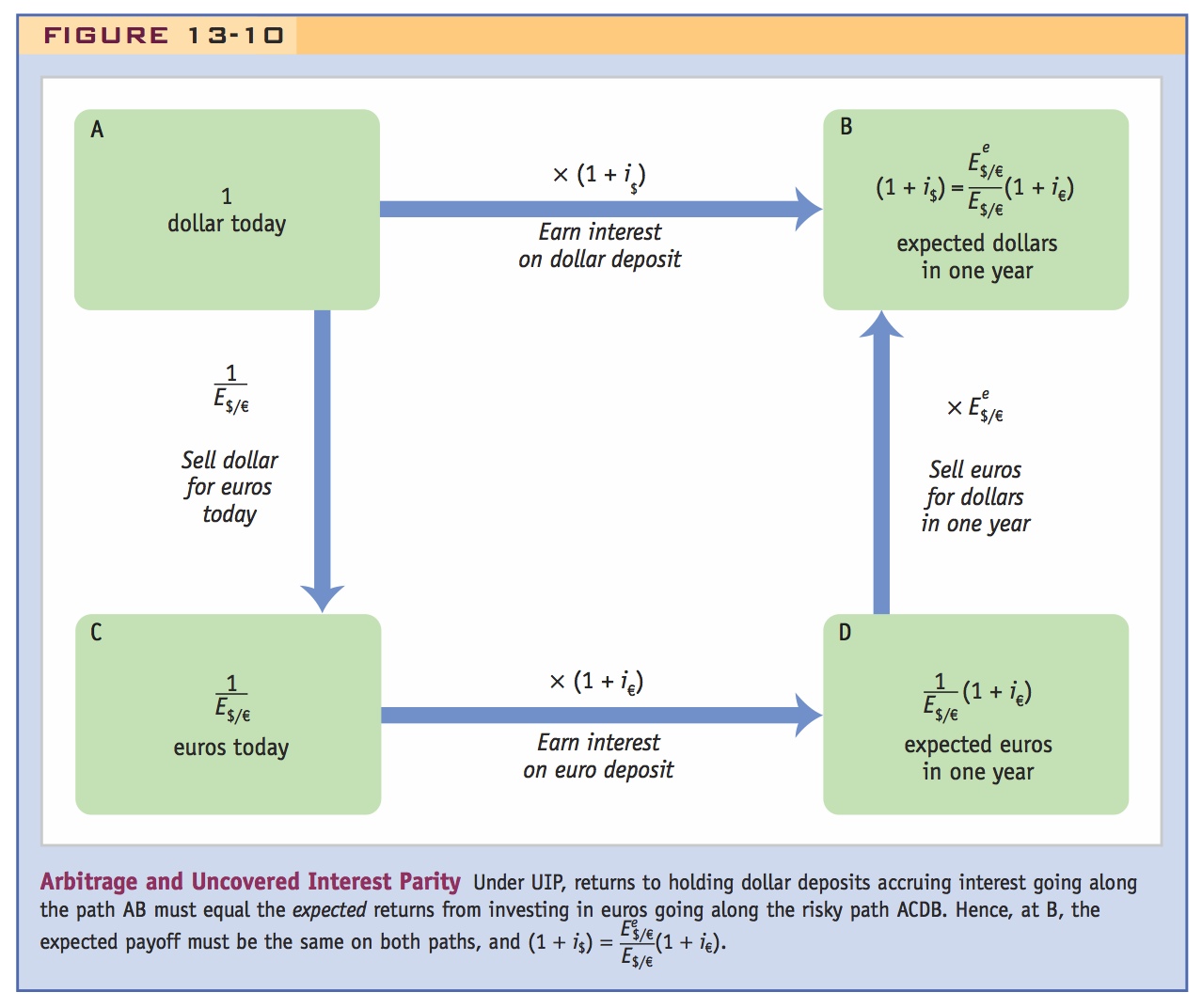
53
This is very important, since it is the beginning of a theory of exchange rate determination. Tell the students that if they see E changing they should work backwards and ask how either expectations or interest rates change. Say that the succeeding chapters will explain what explains expectations and interest rates.
What Determines the Spot Rate? Uncovered interest parity is a no-arbitrage condition that describes an equilibrium in which investors are indifferent between the returns on unhedged interest-bearing bank deposits in two currencies (where forward contracts are not employed). Because one of the returns depends on the spot rate, uncovered interest parity can be seen as providing us with a theory of what determines the spot exchange rate. We can rearrange the above equation and solve for the spot rate:
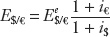
Thus, if uncovered interest parity holds, we can calculate today’s spot rate if we know all three right-hand-side variables: the expected future exchange rate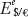 
the dollar interest rate i$; and the euro interest rate i€. For example, suppose the euro interest rate is 2%, the dollar interest rate is 4%, and the expected future spot rate is $1.40 per euro. Then the preceding equation says today’s spot rate would be 1.40 × (1.02)/(1.04) = $1.3731 per euro.
However, this result raises more questions: How can the expected future exchange rate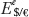 
be forecast? And, as we asked in the case of covered interest parity, how are the two interest rates determined?
In the next two chapters, we address these unanswered questions, as we continue to develop the building blocks needed for a complete theory of exchange rate determination. We start by looking at the determinants of the expected future exchange rate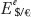 
, and developing a model of exchange rates in the long run, and then by looking at the determinants of the interest rates i$ and i€. We will soon learn that future expectations make the solution of forward-looking economic problems tricky: we have to solve backward from the future to the present, and this motivates the order of the material in this textbook—we must understand exchange rates in the long run before we can understand them in the short run.
This is an important and intuitive insight that bears emphasis.
In every class there will be students who confuse the forward premium with the risk premium. Emphasize that all premia are not created equally. Give the forward premium a name and write down its formula separately before writing this equality.
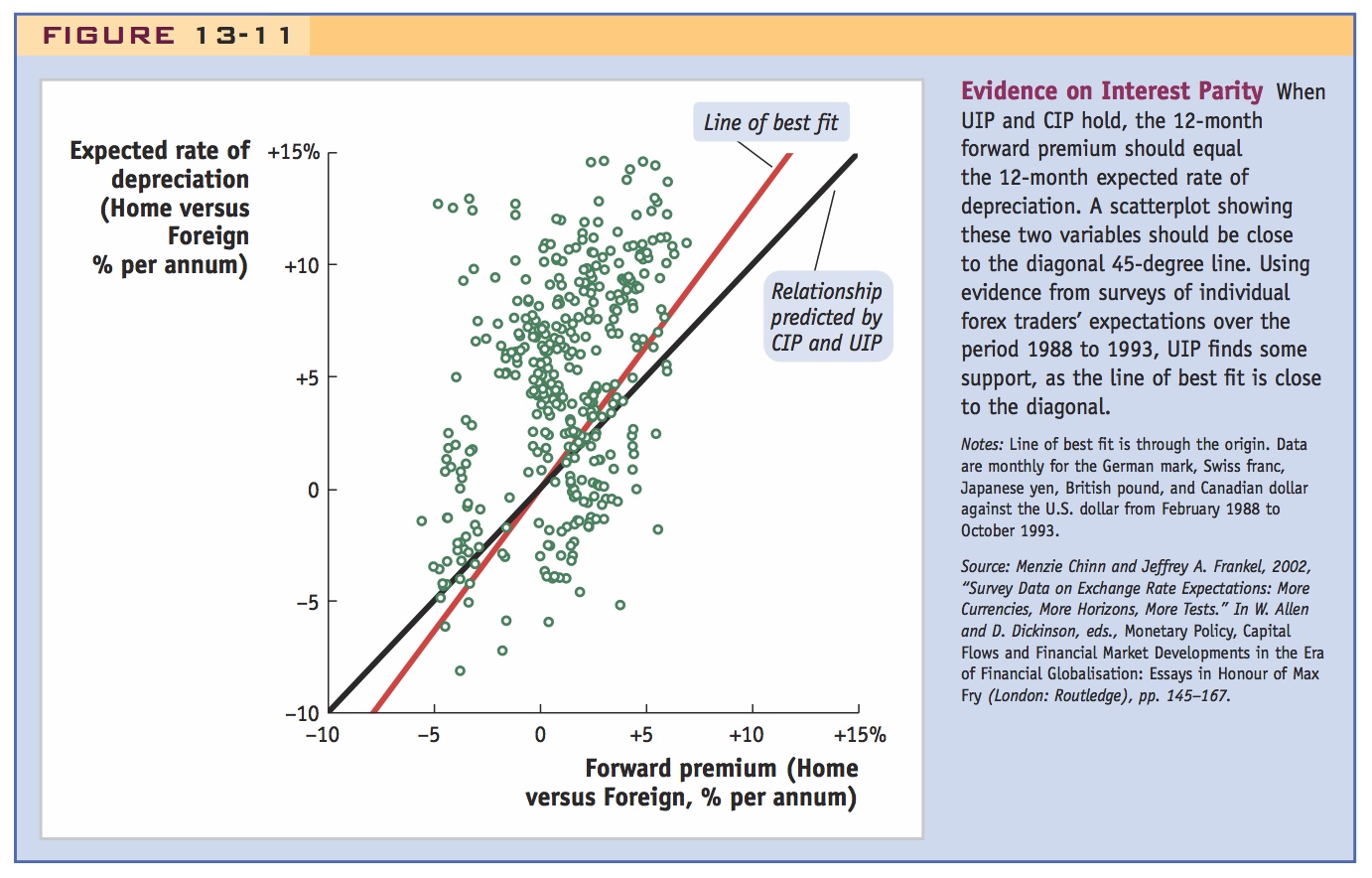
If both CIP and UIP hold then the forward rate should equal the expected future spot rate. Equivalently, the forward premium should equal the expected rate of depreciation. So a simple test of UIP is to ask if this is true. This empirical example provides some support for UIP.
Evidence on Uncovered Interest Parity
Does uncovered interest parity hold? The two interest parity equations seen previously are very similar. Equation (2-1), the CIP equation, uses the forward rate; Equation (2-2), the UIP equation, uses the expected future spot rate:
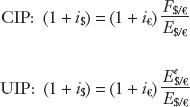
To allow us to see what this implies about the relationship between the expected future spot rate and the forward rate, we divide the second equation by the first, to obtain
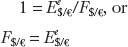
54
The expected future spot rate and the forward rate are distinct concepts. They are also the instruments employed in two different forms of arbitrage—risky and riskless. But, in equilibrium, under the assumptions we have made, we now see that they should not differ at all; they should be exactly the same!
Thus, if both covered interest parity and uncovered interest parity hold, an important relationship emerges: the forward rate F$/€ must equal the expected future spot rate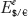 
. The result is intuitive. In equilibrium, and if investors do not care about risk (as we have assumed in our presentation of UIP), then they have no reason to prefer to avoid risk by using the forward rate, or to embrace risk by awaiting the future spot rate; for them to be indifferent, as market equilibrium requires, the two rates must be equal.
With this result we can find an approach to testing UIP that is fairly easy to describe and implement. Because the evidence in favor of CIP is strong, as we have seen, we may assume that it holds. In that case, the previous equation then provides a test for whether UIP holds. But if the forward rate equals the expected spot rate, then we can also express this equivalence relative to today’s spot rate, to show that the expected rate of depreciation (between today and the future period) equals the forward premium (the proportional difference between the forward and spot rates):
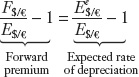
For example, if the spot rate is $1.00 per euro, and the forward rate is $1.05, the forward premium is 5%. But if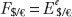 
, the expected future spot rate is also $1.05, and there is a 5% expected rate of depreciation.
We can easily observe the left-hand side of the preceding equation, the forward premium, because both the current spot and forward rates are data we can collect in the market. The difficulty is on the right-hand side: we typically cannot observe expectations. Still, the test can be attempted using surveys in which traders are asked to report their expectations. Using data from one such test, Figure 13-11 shows a strong correlation between expected rates of depreciation and the forward premium, with a slope close to 1. Because expected depreciation does not always equal the interest differential, the points do not lie exactly on the 45-degree line. Does this mean that arbitrage is not working? Not necessarily. The deviations may be caused by sampling errors or noise (differences in opinion of individual traders). In addition, there may be limits to risky arbitrage in the real world because of various factors such as transactions costs (market frictions) and aversion to risk, which we have so far neglected, but which we discuss in more detail in later chapters. That the slope “on average” is close to 1 provides some support for UIP.
Some of us would say this is being far too generous to UIP, but it is the natural benchmark upon which most of the succeeding chapters are built.
Uncovered Interest Parity: A Useful Approximation
3. Uncovered Interest Parity: A Useful Approximation
For small interest rates and rates of depreciation i$ = i€ + ΔEe$/€/E$/€. Meaning: Arbitrage should make returns in different countries equalize, when expressed in the same currency.
Because it provides a theory of how the spot exchange rate is determined, the uncovered interest parity equation (2-2) is one of the most important conditions in international macroeconomics. Yet for most purposes, a simpler and more convenient concept can be used.
55
The intuition behind the approximation is as follows. Holding dollar deposits rewards the investor with dollar interest. Holding euro deposits rewards investors in two ways: they receive euro interest, but they also receive a gain (or loss) on euros equal to the rate of euro appreciation that approximately equals the rate of dollar depreciation. Thus, for UIP to hold, and for an investor to be indifferent between dollar deposits and euro deposits, any interest shortfall (excess) on the euro side must be offset by an expected gain (loss) in the form of euro appreciation or dollar depreciation.
Suggestion: Derive this as in Footnote 11. Let them pick interest rates and a rate of depreciation, plug them into their calculators and be surprised at how well the approximation works.
We can write the approximation formally as follows:
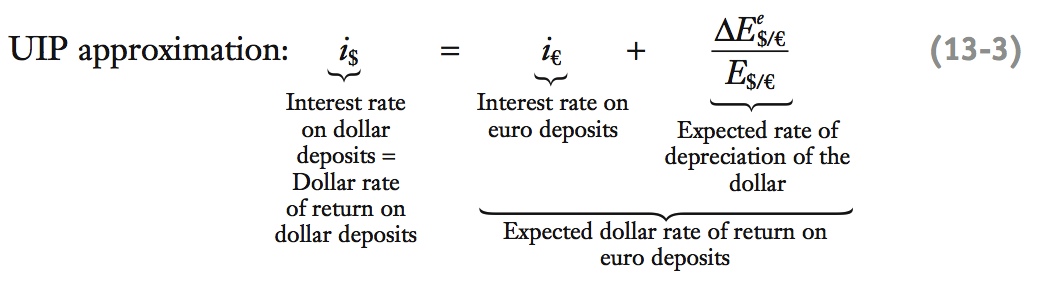
There are three terms in Equation 2-3. The left-hand side is the interest rate on dollar deposits. The first term on the right is the interest rate on euro deposits. The second term on the right can be expanded as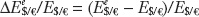 
and is the expected fractional change in the euro’s value, or the expected rate of appreciation of the euro. As we have seen, this expression equals the appreciation of the euro exactly, but for small changes it approximately equals the expected rate of depreciation of the dollar.11
56
The UIP approximation equation, Equation (2-3), says that the home interest rate equals the foreign interest rate plus the expected rate of depreciation of the home currency.
A numerical example illustrates the UIP approximation formula. Suppose the dollar interest rate is 4% per year and the euro interest rate is 3% per year. If UIP is to hold, then the expected rate of dollar depreciation over a year must be 1%. In that case, a dollar investment put into euros for a year will grow by 3% because of euro interest and in dollar terms will grow by an extra 1% because of euro appreciation, so the total dollar return on the euro deposit is approximately equal to the 4% that is offered by dollar deposits.12
To sum up, the uncovered interest parity condition, whether in its exact form (2-2) or its approximate form (2-3), states that there must be parity between expected returns, expressed in a common currency, in the two markets.
Summary
4. Summary
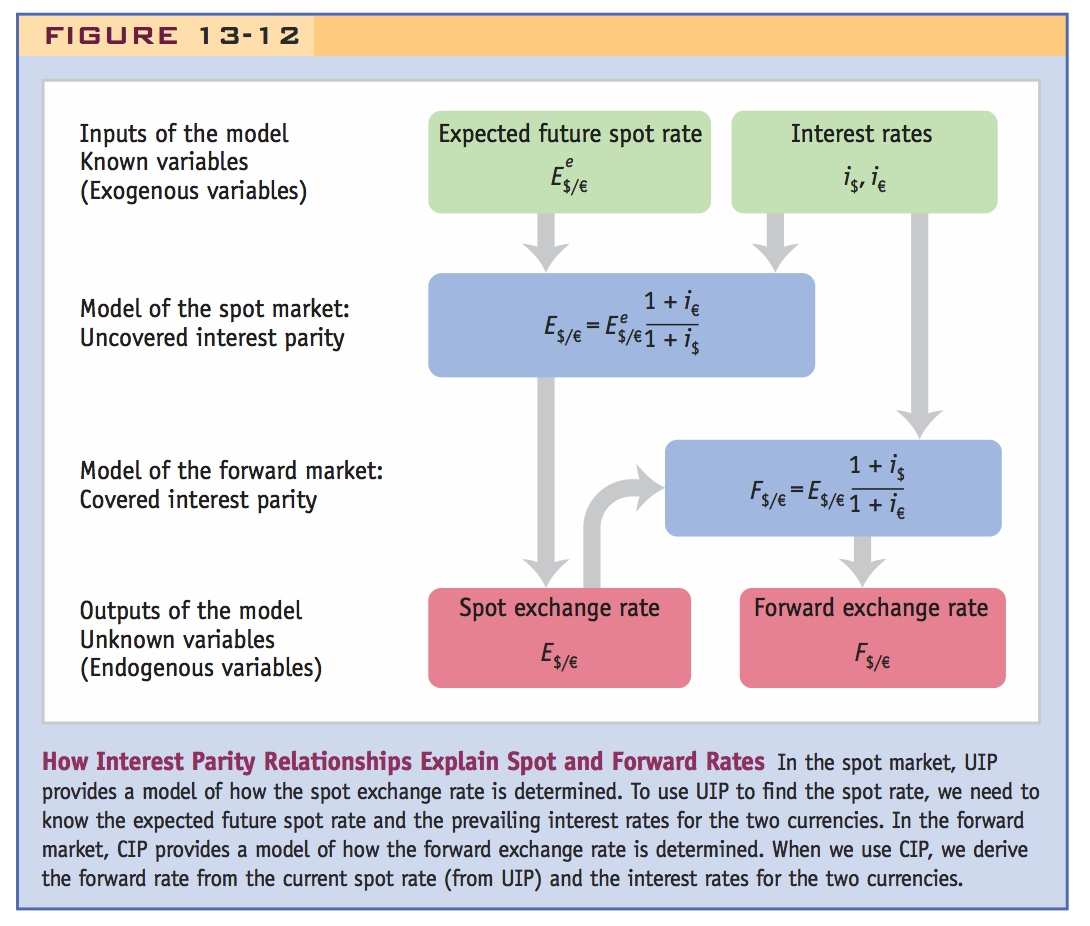
Economic models explain how exogenous variables affect endogenous variables. CIP and UIP show how the two most import variables in the FX market—the spot and forward rates—are determined in equilibrium, when arbitrage opportunities are exhausted.
All economic models produce an output (some unknown or endogenous variable to be explained) and require a set of inputs (some known or exogenous variables that are treated as given). The two interest parity conditions provide us with models that explain how the prices of the two most important forex contracts are determined in market equilibrium with no arbitrage possibilities. Uncovered interest parity applies to the spot market and determines the spot rate, based on interest rates and exchange rate expectations. Covered interest parity applies to the forward market and determines the forward rate based on interest rates and the spot rate. Figure 13-12 sums up what we have learned.
