Screen 1 of 4

Chapter 4Figure It Out 9.3

true

The Power Tires Company has market power and faces the demand curve shown in the figure below. The firm’s marginal cost curve is MC = 30 + 3Q.

Figure A

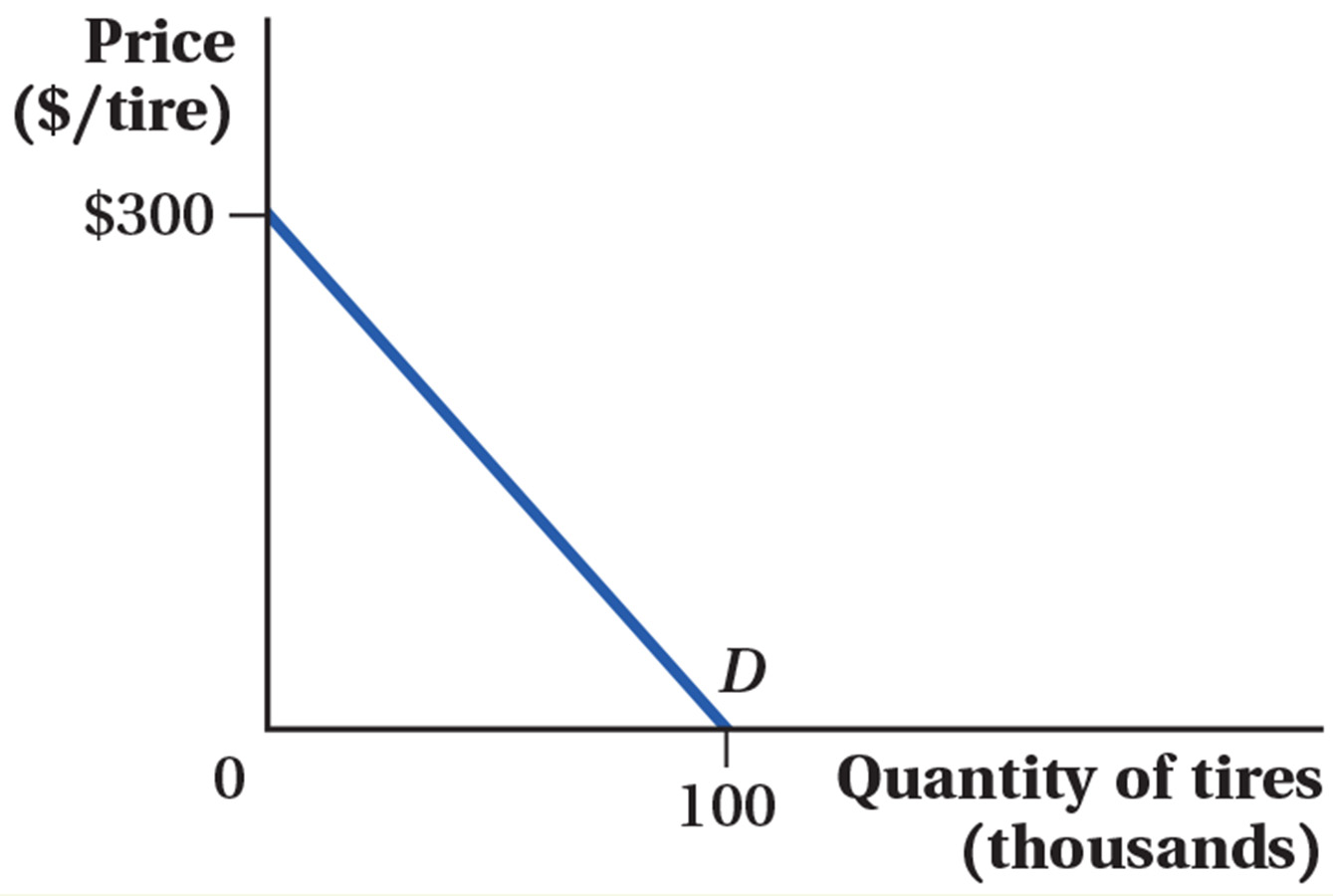
a. Which of the following represents Power Tires’ marginal revenue?

A.
B.
C.
D.
Incorrect. The MR curve starts at the same point as the inverse demand curve, but has twice the slope. The inverse demand curve starts at $300, and has a slope of -300/100, or -3. So, the MR curve must start at 300 and have a slope of -6. Therefore, MR = 300 – 6Q. For further review see section “Market Power and Marginal Revenue”.
Correct! The MR curve starts at the same point as the inverse demand curve (300), but has twice the slope, or -6. So, MR = 300 – 6Q. For further review see section “Market Power and Marginal Revenue”.
GLS 2e FIO 9.3 Part A
Current Time 0:00
Duration 0:00
Loaded: 0%
Stream Type LIVE
Remaining Time 0:00
 
1x
    • Chapters
    • descriptions off, selected

      b. What is the firm’s profit-maximizing output and price?

      The firm’s profit maximizing output is

      The firm’s profit maximizing price is $

      The firm’s profit-maximizing output is found where MR = MC. Power Tire’s marginal revenue is 300 – 6Q; its marginal cost is 30 + 3Q. So, MR = MC when 300 – 6Q = 30 + 3Q, or when Q = 30. Plug that quantity into the inverse demand curve to find the profit-maximizing price of $210. For further review see section “Profit Maximization for a Firm with Market Power”.
      GLS 2e FIO 9.3 Part B
      Current Time 0:00
      Duration 0:00
      Loaded: 0%
      Stream Type LIVE
      Remaining Time 0:00
       
      1x
        • Chapters
        • descriptions off, selected

          c. If the firm’s demand changes to P = 240 – 2Q while its marginal cost remains the same, what is the firm’s profit-maximizing level of output and price? How does this compare to your answer for part (b)?

          The firm’s new profit maximizing output is

          The firm’s new profit maximizing price is $

          The firm’s profit-maximizing output is found where MR = MC. Power Tires’ new marginal revenue is 240 – 4Q (recall that the marginal revenue curve starts at the same point as the inverse demand curve, but has twice the slope). Power Tires’ marginal cost is 30 + 3Q. So, MR = MC when 240 – 4Q = 30 + 3Q, or when Q = 30. Plug that quantity into the new demand curve to find the profit-maximizing price of $180. For further review see section “Profit Maximization for a Firm with Market Power”.
          GLS 2e FIO 9.3 Part C
          Current Time 0:00
          Duration 0:00
          Loaded: 0%
          Stream Type LIVE
          Remaining Time 0:00
           
          1x
            • Chapters
            • descriptions off, selected

              How does the shape of the demand curve affect the firm’s ability to charge a high price?

              		A.
              		B.
              		C.
              		D.
              GLS 2e FIO 9.3 Part D
              Current Time 0:00
              Duration 0:00
              Loaded: 0%
              Stream Type LIVE
              Remaining Time 0:00
               
              1x
                • Chapters
                • descriptions off, selected