Every game, no matter how simple or complex, shares three common elements: players, strategies, and payoffs. We describe what each of these concepts means in the context of game theory to lay the groundwork for our analysis in the rest of the chapter.

The players in a game are the decision makers. They face situations in which outcomes they care about are affected by both their own choices and the choices of others. Players in economic games can be firms (and their managers), consumers, workers, or many other entities. Regardless of their role, however, by definition, all players make choices.

A strategy is a player’s plan of action for a game. Generally, the strategy a player chooses to pursue depends on the anticipated actions of other players. That is, the strategy a player chooses depends on what strategies she thinks her competitors will use.

Strategies can be simple, such as “I’ll redesign my product this year no matter what my competitor does,” or more complex, such as “If the other firm keeps its price high for the next three months, I’ll raise my price. Otherwise, I will keep prices low and try to take some market share from it.” In fact, instead of being dependent on the actions of an opponent, a strategy can even be random: “I’ll flip a coin. If it comes up heads, I’ll charge a low price, and if it comes up tails, I’ll charge a high price.”

467

Payoffs are the outcomes the players receive from playing the game. For consumers, the payoff may be measured in terms of utility or consumer surplus. For firms, payoffs generally represent producer surplus or profits. Most of the time, one player’s payoff depends on the strategies that both the player and her opponents choose. The fact that the players’ actions affect each other’s payoffs and that they know about this effect ahead of time is what makes game theory game theory. When only the player’s own choices affect her payoffs, that is called a single-agent problem. A monopolist choosing an output level given the demand and marginal cost curves it faces or the consumers’ utility-maximization problem are examples of single-agent problems. Once a player’s payoffs are affected by others’ choices, however, it’s a game. The player now has an incentive to understand what motivates her opposing players. The better she can understand her opponent’s motivation, the better the choices she can make in response.

These are the three common elements of games, and you need to understand them to be able to predict the likely outcomes of strategic interactions. But a key to forming those predictions—that is, to pinning down a game’s equilibrium outcome—is to understand how players’ strategies intertwine. The next section deepens our knowledge of strategies before we move on to analyzing various types of games.

Predicting behavior in games is about finding a player’s optimal strategy—the action that has the highest expected payoff. This can be difficult because a particular strategy may be optimal for a player if her opponent chooses one action, but not optimal if her opponent chooses another action (this is another way of saying her optimal strategy must be a best response to the other player’s chosen strategy). Some situations, though, don’t entail that level of complexity; we start by talking about those simpler situations.

If a strategy is always the best thing for a player to do no matter what the other players do, it is called a dominant strategy. Strategies that are never the right thing to do are called dominated strategies.

Let’s think back to our example from the last chapter of the advertising decisions of Warner Brothers and Disney with regard to their animated movies. We made a box that listed each company’s strategy and the subsequent payoffs in millions of dollars of profit to each company. This box, called a payoff matrix, is shown in Table 12.1 (which is a reprint of Table 11.1). The first (left) payoff always belongs to the row player; the second (right) payoff always belongs to the column player. Thus, Warner Brothers’ choices are shown on the left (the rows of the payoff matrix) and its payoffs are listed in red, before the comma. Disney’s choices are listed along the top of the table (the columns of the payoff matrix) and its payoffs are in blue, after the comma.

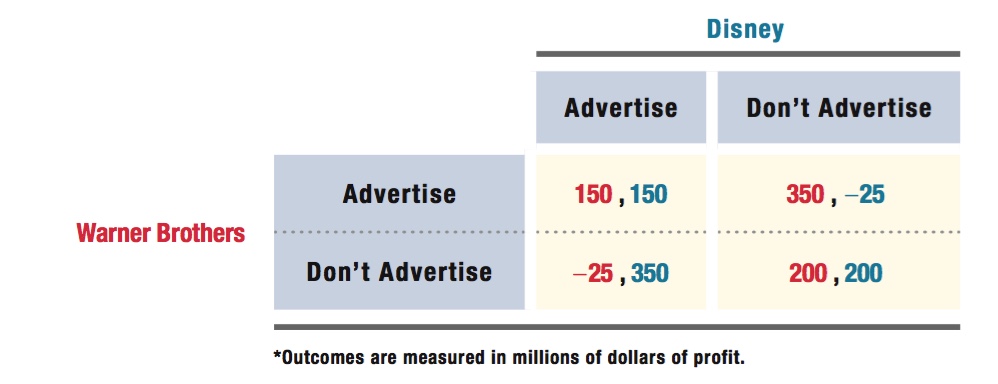
*Outcomes are measured in millions of dollars of profit.

468

In Chapter 11, we saw that this game is a prisoner’s dilemma: Even though Warner Brothers and Disney could both make higher profits if they agreed not to advertise, each had such a strong individual incentive to advertise that they both ended up committing to a full advertising budget.

The expression “prisoner’s dilemma” comes from the classic example often used to introduce people to the concept behind this type of game. In the example, two suspected conspirators are brought in by the police and interviewed in separate rooms. If neither confesses, the district attorney will only have enough evidence to convict them on a lesser charge, and they will quickly go free. If both confess, they will be convicted of a serious crime and severely punished. If one confesses but not the other, the one who confesses will get a lighter sentence for cooperating, and the other will receive an especially harsh sentence. While it is clear the prisoners would be best off if they could coordinate on remaining silent, the payoff structure of the game is set up so that each has the unilateral incentive to confess. This makes the game’s Nash equilibrium a very unappealing one (at least to the suspects): Both confess and receive severe sentences. Here, Warner Brothers and Disney are the prisoners and advertising is akin to confessing.

The reason for this outcome is that in this game, the “Advertise” strategy is a dominant strategy for both companies. That is, Warner Brothers makes more profit by advertising The LEGO Movie 2 whether Disney advertises Frozen 2 or not. If Disney does not advertise, Warner Brothers earns $350 million if it advertises instead of $200 million if it doesn’t. If Disney does advertise, Warner Brothers earns $150 million if it also advertises, but loses $25 million if it doesn’t. If we do a similar analysis for Disney’s response to Warner Brothers’ advertising decisions, we arrive at the same result.

On the other side of the coin, the “Don’t Advertise” strategy is never the best thing to do. In other words, it is a dominated strategy because “Advertise” is a dominant strategy. (When one strategy is dominant, all other strategies must be dominated.) There is never a situation in which Disney’s or Warner Brothers’ payoffs are larger from choosing “Don’t Advertise” than from choosing “Advertise.”

The notion of dominated strategies is useful for finding the equilibrium in a game. Because a dominated strategy is not a player’s best choice under any circumstances, it makes no sense for a player to ever choose such a strategy. So, the first thing to do when analyzing any game is to go through the payoff matrix and eliminate all the dominated strategies as possible equilibrium outcomes for all players. In the game in Table 12.1, this process eliminates the “Don’t Advertise” row for Warner Brothers because Warner Brothers can always achieve a higher payoff from advertising, so it will not select this strategy. Likewise, we want to eliminate the “Don’t Advertise” column for Disney for the same reason. Note that not every game has dominated strategies. If a game has dominated strategies, however, as does this Warner Brothers–Disney game, it is much easier to find the game’s equilibrium outcome once the dominated strategies have been ruled out as possibilities.

After crossing out this row and column, a look at the remaining square shows there’s only one possible strategy for rational players to pursue: Both Warner Brothers and Disney end up advertising and earning $150 million each. Notice that we are sure of this outcome even though both companies must make their decisions simultaneously. That is, they do not know what action the other company will take before making their own choices, but they can anticipate that a rational competitor would never use dominated strategies such as “Don’t Advertise.”