14.1 Present Discounted Value Analysis
Decisions about costs and benefits that occur at different times are more complex to evaluate than those involving costs and benefits that occur at the same time.
When things happen simultaneously, comparing costs and benefits is straightforward; if an action’s benefits outweigh its costs, then do it. But, consider a capital investment decision that involves a $1,000 cost now and pays off $1,200 in five years. Is this investment a good idea? Or, suppose an investment involved costs of $500 now, and again one and two years from now, and paid benefits of $400 one, two, three, and four years from now. How can you evaluate that choice? And what if those future benefits are uncertain and may not be paid because the outcome of the investment is risky?
present discounted value (PDV)
The value of a future payment in terms of equivalent present-
Because the costs and benefits of all the decisions we’ve analyzed up to now have occurred at the same time, we need some new tools to evaluate the types of decisions that have costs and benefits that occur over time. The first tool we introduce in this section is the concept of present discounted value (PDV), a mathematical concept that allows us to compare costs and benefits over time in a way that puts all present and future financial values on equal footing.
Interest Rates
Interest rates and rates of return play a key role in present discounted value analysis. You’ve dealt with these concepts in your personal finances (savings accounts, car loans, student loans, mutual fund or stock holdings, etc.), but it’s worth a quick review to set up our present discounted value analysis.
interest
A periodic payment tied to an amount of assets borrowed or lent.
principal
The amount of assets on which interest payments are made.
interest rate
Interest expressed as a fraction of the principal.
Interest is a periodic payment made by individuals or firms that depends on the value of the assets the interest payments are tied to. The value of the assets is called the principal. The interest rate is the amount of interest paid, expressed as a fraction of the principal. Interest rates are quoted on a per-
compounding or compound interest
A calculation of interest based on the sum of the original principal and the interest paid over past periods.
When interest paid in one period is added to the principal, and the interest rate in the next period is applied to the sum, this is called compounding or compound interest. Suppose our savings account pays a 4% interest rate that is compounded annually. An initial principal deposit of $100 earns $4 interest after one year. If the account holder keeps this interest in the account, the principal becomes $104. The interest in the second period is computed based on this new principal, making it $104 × 0.04 = $4.16. Notice how this interest payment is slightly more than the first-
543
You can see how the compounding process works. Starting with an amount of principal A and an interest rate r, our principal after one time period would be A + (A × r) = A × (1 + r). (In the example above, A = $100 and r = 0.04.) After two periods, the principal would grow to
A × (1 + r) × (1 + r) = A × (1 + r)2
After three periods, the principal would become
A × (1 + r)2 × (1 + r) = A × (1 + r)3
Repeating this type of calculation shows that the value of the account after t periods, Vt, will be
Vt = A × (1 + r)t
Figure 14.1 uses this formula to plot how an initial principal amount of $100 would grow over 30 years. It shows three cases. The bottom line traces the account balance growth when the interest rate is 2%, the middle line shows the case of a 4% interest rate, and the top line assumes a 6% rate. Higher interest rates lead to faster growth—
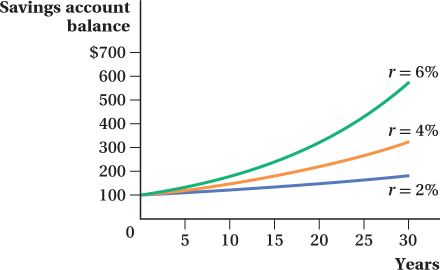
Notice that because of compounding, the same gap in interest rate levels can imply very different balances after extended periods. With a 2% interest rate, for example, the balance after 30 years is $100 × (1.02)30 = $181.14. With a 4% interest rate, it’s $100 × (1.04)30 = $324.34, or $143.20 higher. But, with a 6% interest rate, the 30th-
Also, because of compounding, the number of periods it takes the balance to double is less than you might think. Many people would guess that with a 4% interest rate, it might take 25 years for the $100 initial principal to grow to $200. But, it happens in the 18th year because you earn more than $4 per year in interest as the account compounds in size. For a 6% rate, the balance goes above $200 in the 12th year. And it takes about 35 years with a 2% rate.
544
The “Rule of 72”
There’s a handy rule-
An account compounding at 4% per year should double every 72/4 = 18 years, just as we saw in our example above. It should take about 72/6 = 12 years for a sum to double at a 6% rate, and 72/2 = 36 years for the principal to double at the 2% rate. These numbers are close to what we found in our example.
Present Discounted Value
As we discussed in the chapter’s introduction, a fundamental feature of capital investment decisions is that their costs and benefits are incurred and earned at different times. Typically, investment decisions involve spending money in the present to earn benefits in later periods. Adding up an investment project’s benefits and subtracting its costs while ignoring when they occur would be easy, but doesn’t make sense. A project costing $1,000 today and paying $1,001 in 50 years is a bad idea, even without inflation, as we will explain shortly. We need a way to adjust expenditures and payoffs that happen at different times so that they can be compared on a consistent basis. Present discounted value (PDV) analysis (sometimes called just present value analysis) uses the concepts of interest rates and compounding that we just discussed, but in reverse. Here’s how.
In the previous section, we figured out how large an initial principal value would grow to be if compounded at a given interest rate. Present discounted value takes a future dollar value and asks how large the initial principal would have to be today in order to grow at a given interest rate to that future value. For example, let’s say we wanted to know the present value of a $104.00 payment one year from now at an interest rate of 4%. At 4% interest, the principal today must be $100; at 4%, the $100 will grow to $104 in one year. So, at 4% interest, the PDV of $104 next year—
Discounting can also be used to compare payments that happen more than one period apart. For example, again assuming a 4% interest rate, the PDV of $108.16 in two years (what we saw above would be the account balance after two years) is $100. The PDV of $112.49 in three years at 4% is also $100.
Present discounted values use interest rates and compounding to compare payments happening at different times. The idea is to appropriately discount future values so that they can be put in terms of equivalent present-
Remember in our examples above that the growth of the account balance depended on the interest rate. Just as the interest rate affects the relative sizes of the initial principal and future account balances, it also operates in reverse to affect the PDV of a given future value. Consider the case of a $104 payment that will occur in one year. We know that this payment’s PDV is $100 at a 4% rate, but what if the interest rate were different? Suppose it was 6%. To find the PDV in this case, we have to find what initial principal would grow at a 6% rate to be exactly $104 after one year. This is easy using the formula we derived above. In this case,
545
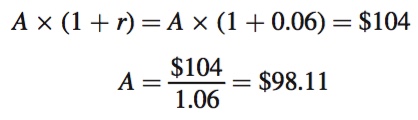
This means that the PDV of $104 is $98.11 when the interest rate is 6%. If we did the same exercise with a 2% interest rate, we would find that the PDV of $104 at this rate is $101.96.
It’s important to notice from these examples that the PDV of a given future value is always inversely related to the interest rate. That is, the higher the interest rate, the smaller the initial principal needs to be to grow to the same future value. Therefore, a particular future-
All we had to do to compute the PDVs above was reverse the equation for the future account balance under compounding. We solved for the initial principal that would be needed to grow to that future value. That’s what a present discounted value is. We saw above that any initial principal A growing at interest rate r for t periods will grow to a value of
Vt = A × (1 + r)t
A PDV reinterprets Vt as the future payment that needs to be expressed in present-
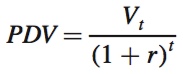
We can use this equation to find the present discounted value of any payment Vt that occurs t periods in the future. This equation plays a central role in much of what we do in this chapter. Knowing how to calculate PDV is practical, too. If you go to buy a car and the salesperson tells you that you can either buy the car for $20,000 or lease it for $2,000 down and $500 per month, you need to figure out the PDV of the payments to determine which option is the better deal.
The present value equation has some important implications. First, PDVs are proportional to the future value being discounted. If Vt were twice as high, its PDV would be, too.
Second, just as we noted above, higher interest rates imply lower PDVs for fixed values of Vt and t. The intuition is that higher interest rates reduce the initial value necessary to grow to future value Vt.3
Finally, the PDV of any particular value Vt is smaller the further into the future that it occurs. The PDV of $104 one year from now is greater than the PDV of $104 in two years, which is itself greater than the PDV of $104 three years from now, and so on.
Present Discounted Value of Payment Streams We just saw how we can find the PDV of a payment that occurs at one particular moment in the future. It’s easy to extend this method to payment streams—collections of payments that happen at different times. To compute the PDV of an entire stream, we just apply the present value discounting equation to each of the stream’s elements and then add them together.
Suppose you earn a scholarship that will pay you $1,000 in each of four installments: The first installment arrives today, and then the next three come one, two, and three years from today. The PDV of the scholarship is the sum of the PDVs of each installment. So, for any generic annual interest rate r, the scholarship’s PDV is
546
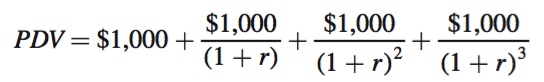
The first $1,000 payment is not discounted because it occurs in the present period. (This is what the PDV formula implies, too. If t = 0—
The interest rate affects the PDV of a stream of payments in the same way that it affects a single payment. For r = 0.04 (a 4% interest rate), for example, the scholarship’s PDV = $1,000 + $961.54 + $924.55 + $889.00 = $3,775.09. This present value is less than the simple $4,000 sum of the payments because of discounting. Payments in the future are not equivalent to the same dollar-
Special Cases of PDVs There are some commonplace payment stream patterns that have general PDV formulas that are worth learning. One is a constant payment that is made for a set number of periods. For example, a car loan might require a payment of $400 per month for 60 months. Fixed-
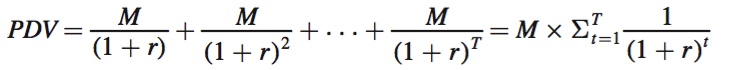
If we simplify the series of terms with 1/(1 + r)t for various t, we end up with
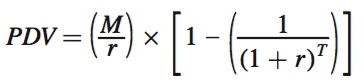
The PDV of this stream of payments is proportional to the regular payment M. That means if you borrow twice as much at the same terms (interest rate and repayment length), your regular payment will be twice as high. The payment stream’s PDV is negatively related to the interest rate, but not proportionately. Specifically, changes in r have the largest effect on the PDV at low interest rates; their influence declines in size as r climbs. Finally, the PDV of the payment stream grows with T. This is no surprise; the longer the payments last, the greater the total value of those payments. This formula also shows the familiar tradeoff facing many borrowers: When borrowing a fixed amount of money (i.e., a given PDV), a borrower can often lower his payment M by agreeing to a longer payback term T.
An interesting special case of this formula occurs when T goes to infinity, meaning a payment of M every period, forever. (This type of arrangement is called a perpetuity.) The PDV of this perpetual stream can be expressed as
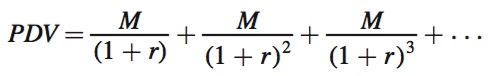
This may look like it’s infinitely large, but it’s not because the (1 + r)t values in the denominator grow fast enough with time that the PDV of payments in the far distant future is basically worth zero. You can see the result of this property by plugging a large number for T into the PDV formula. For instance, if the final payment occurs 500 years from now, this makes the expression (1 + r)500 in the denominator very large. With an interest rate of 4%, you would divide the 500th-
547
When T goes to infinity, the PDV formula above simplifies to
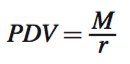
This says that the PDV of any regular payment occurring forever equals the payment divided by the interest rate. If the interest rate is 5%, the PDV of a payment M is 20 times the payment 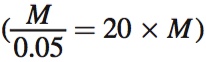 . If the interest rate is 10%, the PDV is 10 × M; for r = 2%, the PDV is 50 × M.
. If the interest rate is 10%, the PDV is 10 × M; for r = 2%, the PDV is 50 × M.
While you may believe that the idea of a payment occurring infinitely seems unrealistic, there are actually a few investment choices or financial instruments that pay off forever. One of the best known is a type of bond, called a consol, issued by the government of the United Kingdom. Consols pay a constant interest payment forever to whoever holds the bond. They don’t sell for infinite prices.
British government debt aside, the perpetuity PDV formula is useful as a shorthand way to approximate PDVs. It’s not easy to compute the PDVs of even steady payment streams in your head, but if you can assume the payments continue forever, you can approximate it. This approximation is an upper bound: Any other type of payment stream would end at some period T < ∞, so the perpetuity PDV approximation is always higher than the true value.
To see an example of this, suppose you are talking to a friend who is thinking about buying a business. He is confident the business can earn a profit of $100,000 per year for the foreseeable future. The business is being sold for $1.2 million. If the interest rate is 10% (and expected to stay at or near that level), would you advise him to buy? Not at that price: Even if the business paid that $100,000 profit forever, the PDV of the business is only
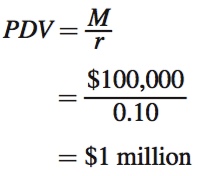
The business’s earnings can’t justify the $1.2 million asking price. On the other hand, if the interest rate is 5%, then the PDV of the business would be, at most, $100,000/0.05 = $2 million, which would make the $1.2 million price worth considering.
figure it out 14.1
Suppose that Emmy is going to turn 21 exactly one year from today, and she wants to throw a spectacular party. Emmy would like to have $1,000 to spend, and wants to set aside enough money today to fund her $1,000 party in a year.
If interest rates are 6%, how much does Emmy have to set aside today?
If interest rates are 9%, how much does Emmy have to set aside today?
What happens to the amount she needs to set aside as interest rates change? Explain.
Solution:
We can use the present discounted value (PDV) formula to determine the amount that Emmy needs to set aside today to have $1,000 one year from now if the interest rate is 6%:
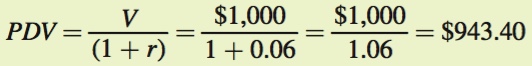
If the interest rate rises to 9%, Emmy will need to set aside
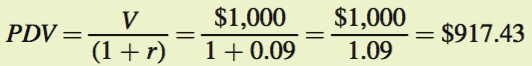
to fund her party.
As the interest rate rises, the present value of $1,000 falls. This is because Emmy’s current funds will grow more quickly at a higher interest rate. Therefore, she will not need to set as much aside to fund her party.
548