Concept 8.1: Genes Are Particulate and Are Inherited According to Mendel’s Laws
Genetics, the field of biology concerned with inheritance, has a long history. There is good evidence that people were deliberately breeding animals (horses) and plants (the date palm tree) for desirable characteristics as long as 5,000 years ago. The general idea was to examine the natural variation among the individuals of a species and “breed the best to the best and hope for the best.” This was a hit-or-miss method—sometimes the resulting offspring had all the good characteristics of the parents, but often they did not.
By the mid-nineteenth century, two hypotheses had emerged to explain the results of breeding experiments:
- The hypothesis of blending inheritance proposed that gametes contained hereditary determinants (what we now call genes) that blended when the gametes fused during fertilization. Like inks of different colors, the two different determinants lost their individuality after blending and could never be separated. For example, if a plant that made smooth, round seeds was mated (crossed) with a plant that made wrinkled seeds, the offspring would be intermediate between the two and the determinants for the two parental characteristics would be lost.
- The hypothesis of particulate inheritance proposed that each determinant had a physically distinct nature; when gametes fused in fertilization, the determinants remained intact. According to this theory, if a plant that made round seeds was crossed with a plant that made wrinkled seeds, the offspring (no matter the shape of their seeds) would still contain the determinants for the two characteristics.
The story of how these competing hypotheses were tested provides a great example of how the scientific method can be used to support one theory and reject another. In the following sections we will look in detail at experiments performed in the 1860s by an Austrian monk and scientist, Gregor Mendel, whose work clearly supported the particulate hypothesis.
Mendel used the scientific method to test his hypotheses
After entering the priesthood at a monastery in Brno, in what is now the Czech Republic, Gregor Mendel was sent to the University of Vienna, where he studied biology, physics, and mathematics. He returned to the monastery in 1853 to teach. The abbot in charge had set up a small plot of land to do experiments with plants and encouraged Mendel to continue with them. Over seven years, Mendel made crosses with many thousands of plants. Analysis of his meticulously gathered data suggested to him that inheritance was due to particulate factors.
Mendel presented his theories in two public lectures in 1865 and a detailed written publication in 1866, but his work was ignored by mainstream scientists until 1900. By that time, the discovery of chromosomes had suggested to biologists that genes might be carried on chromosomes. When they read Mendel’s work on particulate inheritance, the biologists connected the dots between genes and chromosomes.
Mendel chose to study the common garden pea because it is easily cultivated and is amenable to manipulation and controlled crosses. Pea flowers have both male and female sex organs—stamens and pistils, which produce gametes that are contained within the pollen and ovules, respectively:
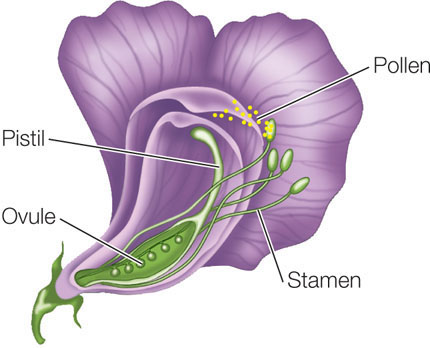
Pea flowers normally self-fertilize. However, the male organs can be removed from a flower so that it can be manually fertilized with pollen from a different flower.
There are many varieties of pea plants with easily recognizable characteristics. A character is an observable physical feature, such as seed shape. A trait is a particular form of a character, such as round or wrinkled seeds. Mendel worked with seven pairs of varieties with contrasting traits for characters such as seed shape, seed color, and flower color. These varieties were true-breeding: that is, when he crossed a plant that produced wrinkled seeds with another of the same variety, all of the offspring plants produced wrinkled seeds.
As we will see, Mendel developed a set of hypotheses to explain the inheritance of particular pea traits, and then designed crossing experiments to test his hypotheses. He performed his crosses in the following manner:
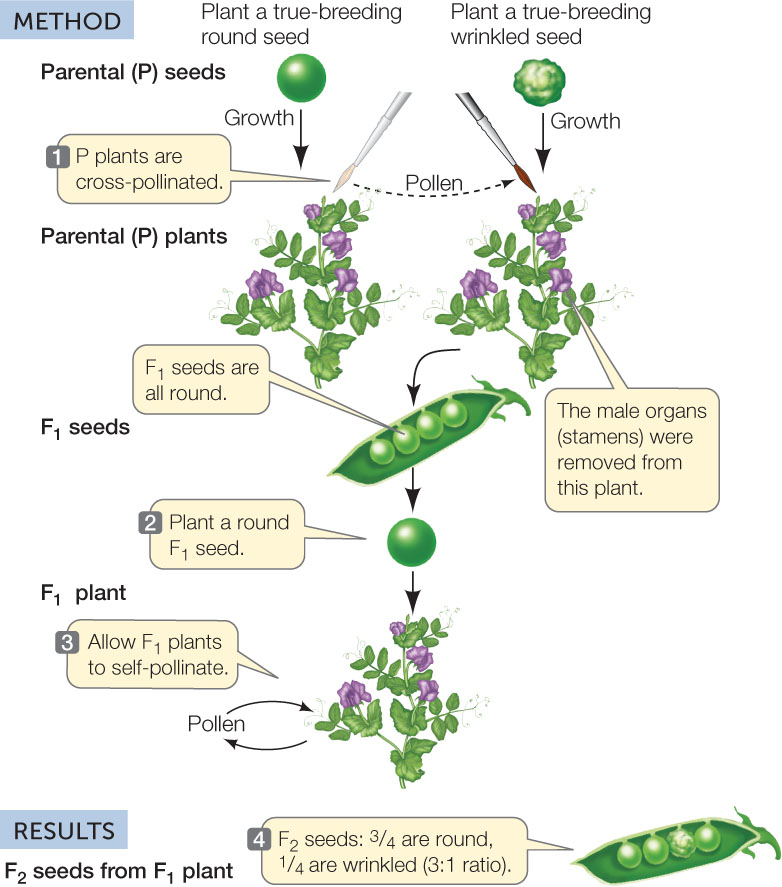
- He removed the stamens to emasculate flowers of one parental variety so that it couldn’t self-fertilize. Then he collected pollen from another parental variety and placed it on the pistils of the emasculated flowers. The plants providing and receiving the pollen were the parental generation, designated P. The pollen provider was the male parent, and the pollen receiver was the female parent.
- In due course, seeds formed and were planted. The seeds and the resulting new plants constituted the first filial generation, or F1 (The word “filial,” from the Latin filius, “son,” refers to the relationship between offspring and parents.) Mendel examined each F1 plant to see which traits it bore and then recorded the number of F1 plants expressing each trait.
- In some experiments the F1 plants were allowed to self-pollinate and produce a second filial generation, the F2. Again, each F2 plant was characterized and counted.

Go to MEDIA CLIP 8.1 Mendel’s Discoveries
PoL2e.com/mc8.1
152
Mendel’s first experiments involved monohybrid crosses
The term “hybrid” refers to the offspring of crosses between organisms differing in one or more characters. In Mendel’s first experiments, he crossed parental (P) varieties with contrasting traits for a single character, producing monohybrids (from the Greek monos, “single”) in the F1 generation. He subsequently planted the F1 seeds and allowed the resulting plants to self-pollinate to produce the F2 generation. This technique is referred to as a monohybrid cross.
Mendel performed the same experiment for seven pea characters. His method is illustrated in FIGURE 8.1, using seed shape as an example. When he crossed a strain that made round seeds with one that made wrinkled seeds, the F1 seeds were round—it was as if the wrinkled seed trait had disappeared completely. However, when F1 plants were allowed to self-pollinate to produce F2 seeds, about one-fourth of the seeds were wrinkled. These observations were key to distinguishing the two theories noted above:
- The F1 offspring were not a blend of the two traits of the parents. Only one of the traits was present (in this case, round seeds).
- Some F2 offspring had wrinkled seeds. The trait did not disappear.
Investigation
HYPOTHESIS
When two strains of peas with contrasting traits are bred, their characteristics are irreversibly blended in succeeding generations.
CONCLUSION
The hypothesis is rejected. There is no irreversible blending of characteristics, and a recessive trait can reappear in succeeding generations.
ANALYZE THE DATA
The table gives Mendel’s data—the number of offspring showing each trait—for the F2 from crosses between P generation plants with contrasting traits:
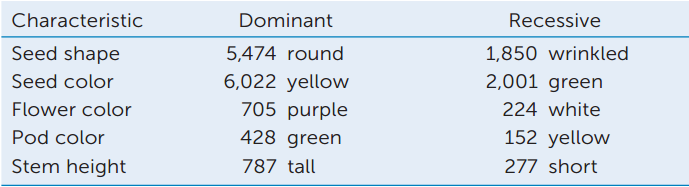
- Calculate the phenotypic ratio of dominant:recessive in the F2 offspring.
- What can you conclude about the behavior of alleles during gamete formation in a plant that is heterozygous for a trait?
- Perform a chi-square test to evaluate the statistical significance of these data (refer to Appendix B).
Go to LaunchPad for discussion and relevant links for all INVESTIGATION figures.
aSee www.mendelweb.org/Mendel.plain.html
These observations led to a rejection of the blending theory of inheritance and provided support for the particulate theory. We now know that hereditary determinants are not actually “particulate,” but they are physically distinct entities: sequences of DNA carried on chromosomes (see Concept 8.3).
All seven crosses between varieties with contrasting traits gave the same kind of data (see Figure 8.1). In the F1 generation only one of the two traits was seen, but the other one reappeared in about one-fourth of the offspring in the F2 generation. Mendel called the trait that appeared in the F1 and was more abundant in the F2 the dominant trait, and the other trait recessive.
Mendel went on to expand the particulate hypothesis. He proposed that hereditary determinants—what we know today as genes, though Mendel did not use that term—occur in pairs and segregate (separate) from one another during the formation of gametes. He concluded that each pea plant has two copies of the gene for each character (such as seed shape), one inherited from each parent. We now use the term diploid to describe the state of having two copies of each gene; haploids have just one copy (see Concept 7.1).
Mendel concluded that while each gamete contains one copy of each gene (i.e., is haploid), the resulting zygote contains two copies (is diploid), because it is produced by the fusion of two gametes. Furthermore, different traits arise because there can be different forms of a gene—now called alleles—for a particular character. For example, Mendel studied two alleles for seed shape: one that caused round seeds and the other causing wrinkled seeds.
153
- An organism that is homozygous for a gene has two alleles that are the same (for example, two copies of the allele for round seeds).
- An organism that is heterozygous for a gene has two different alleles (for example, one allele for round seeds and one allele for wrinkled seeds).
In a heterozygote, one of the two alleles may be dominant (such as round, R) and the other recessive (wrinkled, r). By convention, we designate dominant alleles with uppercase letters and recessive alleles with lowercase letters.
- The physical appearance of an organism is its phenotype.
- The phenotype is the result of the genotype, or genetic constitution, of the organism.
Round seeds and wrinkled seeds are two phenotypes resulting from three possible genotypes: the wrinkled seed phenotype is produced by the genotype rr, whereas the round seed phenotype is produced by either of the genotypes RR or Rr (because the R allele is dominant to the r allele).
Mendel’s first law states that the two copies of a gene segregate
How do Mendel’s theories explain the proportions of traits seen in the F1 and F2 generations of his monohybrid crosses? Mendel’s first law—the law of segregation—states that when any individual produces gametes, the two copies of a gene separate, so each gamete receives only one copy. Thus gametes from a parent with the RR genotype will all be R; gametes from an rr parent will all be r; and the progeny derived from a cross between these parents will all be Rr, producing seeds with a round phenotype (FIGURE 8.2).
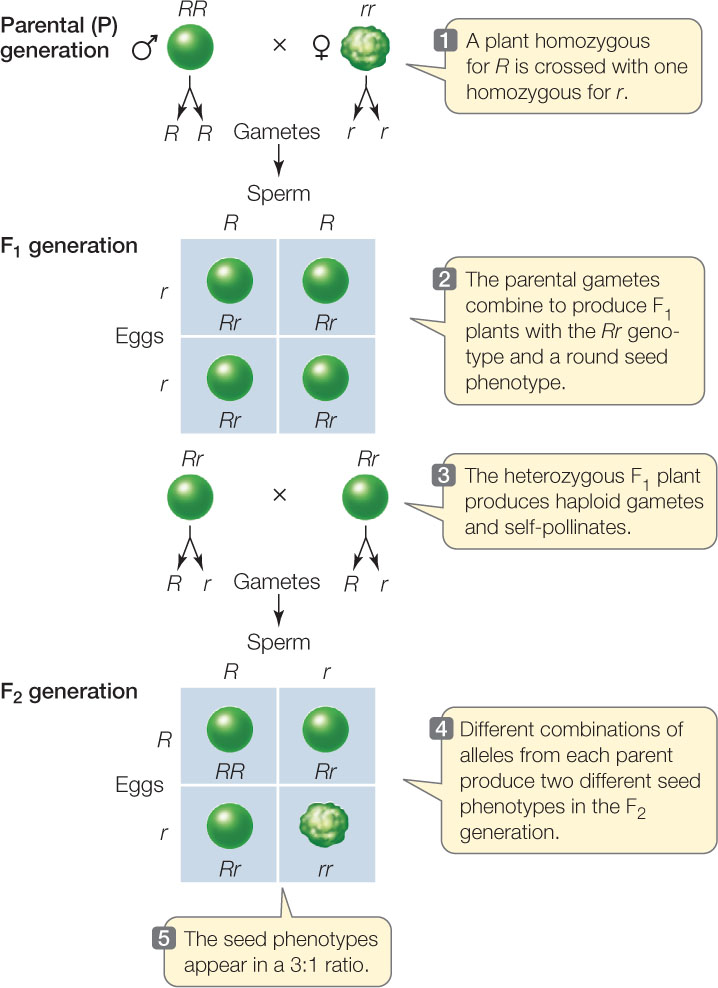
Now let’s consider the composition of the F2 generation. Because the alleles segregate, half of the gametes produced by the F1 generation will have the R allele and the other half will have the r allele. What genotypes are produced when these gametes fuse to form the next (F2) generation? We can predict the allele combinations that will result from a cross using a Punnett square, a method devised in 1905 by the British geneticist Reginald Punnett. This device ensures that we consider all possible random combinations of gametes when calculating expected genotype frequencies. A Punnett square looks like this:
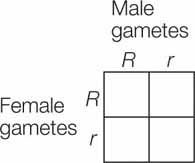
All possible male gamete (haploid sperm) genotypes are shown along the top and all possible female gamete (haploid egg) genotypes along the left side. The grid is completed by filling in each square with the diploid genotype that can be generated from each combination of gametes. In this example, to fill in the top right square, we put in the R from the female gamete (the egg cell) and the r from the male gamete (the sperm cell in the pollen tube), yielding Rr.
Once the Punnett square is filled in, we can readily see that there are four possible combinations of alleles in the F2 generation: RR, Rr, rR, and rr (see Figure 8.2). Since R is dominant, there are three ways to get round-seeded plants in the F2 generation (RR, Rr, or rR), but only one way to get a plant with wrinkled seeds (rr). Therefore we predict a 3:1 ratio of these phenotypes in the F2 generation, remarkably close to the values Mendel found experimentally for all seven of the traits he compared.
Mendel did not live to see his theories placed on a sound physical footing with the discoveries of chromosomes and DNA. Genes are now known to be relatively short sequences of DNA (usually a few thousand base pairs in length) found on the much longer DNA molecules that make up chromosomes (which are often millions of base pairs long). Today we can picture the different alleles of a gene segregating as chromosomes separate during meiosis I (FIGURE 8.3).
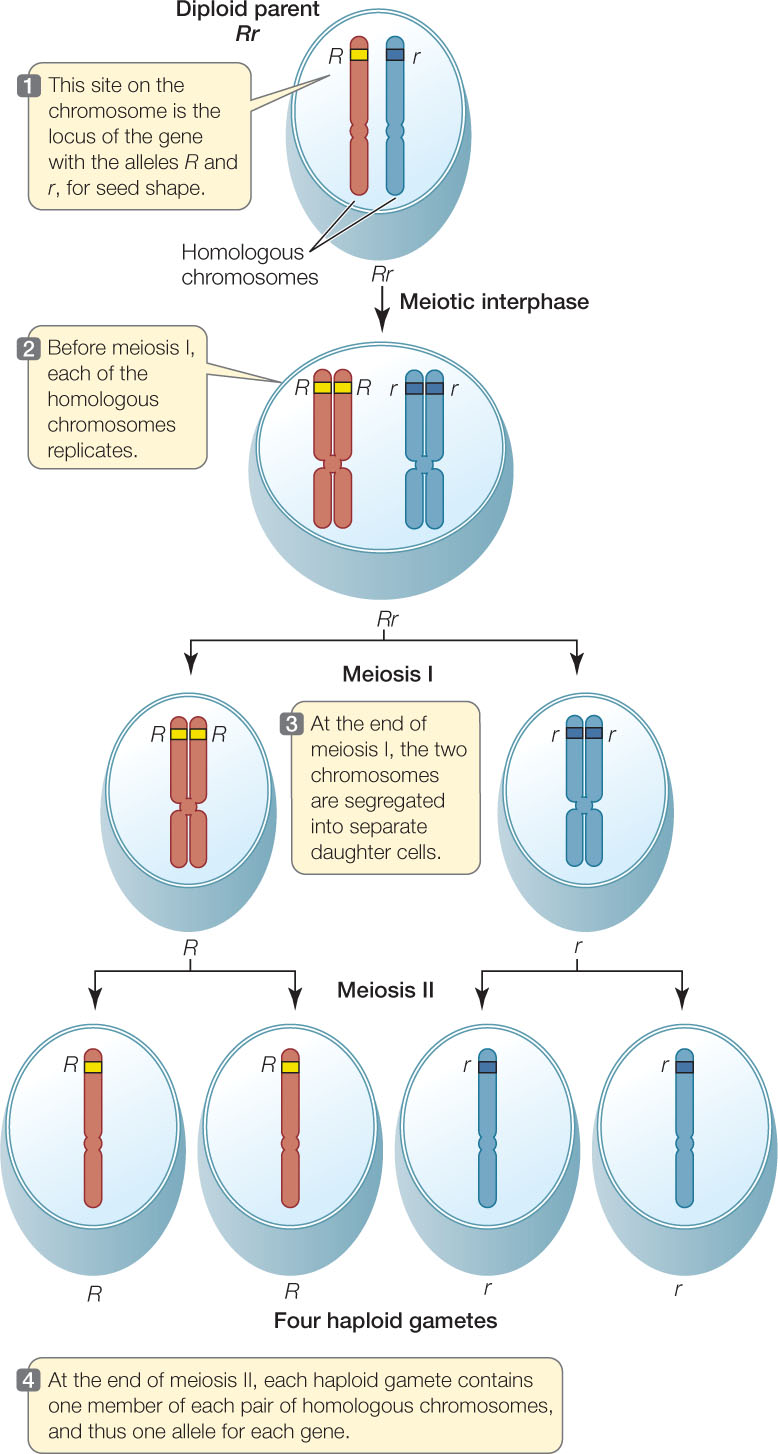
LINK
You can review the process of meiosis and the separation of chromosomes in Concept 7.4
154
Genes determine phenotypes mostly by producing proteins with particular functions, such as enzymes. So in many cases a dominant gene is expressed (transcribed and translated; see Concept 3.1) to produce a functional protein, whereas a recessive gene is mutated so that it is no longer expressed, or it encodes a mutant protein that is nonfunctional. For example, the molecular nature of the wrinkled pea seed phenotype is the absence of an enzyme called starch branching enzyme 1 (SBE1), which is essential for starch synthesis. With less starch, the developing seed has more sucrose, which causes an inflow of water by osmosis. When the seed matures, this water is lost, leaving a shrunken, wrinkled seed.
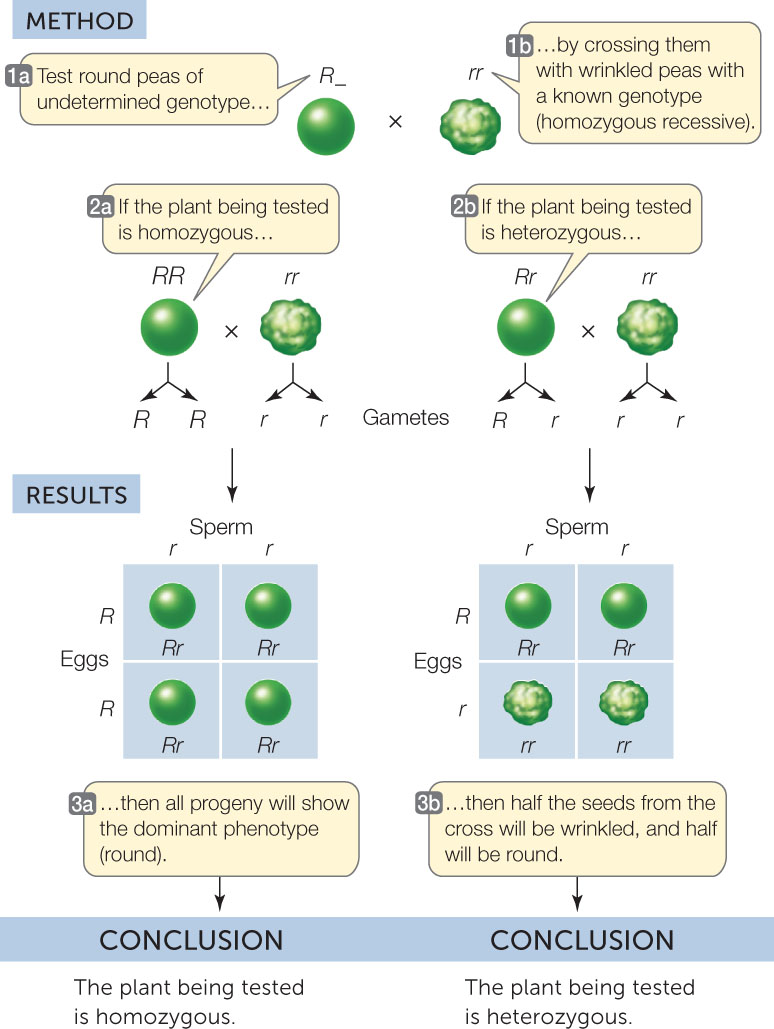
Mendel verified his hypotheses by performing test crosses
As mentioned above, Mendel arrived at his laws of inheritance by developing a series of hypotheses and then designing experiments to test them. One such hypothesis was that there are two possible allele combinations (RR or Rr) for seeds with the round phenotype. Mendel verified this hypothesis by performing test crosses with F1 seeds derived from a variety of other crosses. A test cross is used to determine whether an individual showing a dominant trait is homozygous or heterozygous. The individual in question is crossed with an individual that is homozygous for the recessive trait—an easy individual to identify, because all individuals with the recessive phenotype are homozygous for that trait.
The recessive homozygote for the seed shape gene has wrinkled seeds and the genotype rr. The individual being tested may be described initially as R_ because we do not yet know the identity of the second allele. We can predict two possible results:
- If the individual being tested is homozygous dominant (RR), all offspring of the test cross will be Rr and show the dominant trait (round seeds) (FIGURE 8.4, LEFT).
- If the individual being tested is heterozygous (Rr), then approximately half of the offspring of the test cross will be heterozygous and show the dominant trait (Rr), and the other half will be homozygous for the recessive trait (rr) (FIGURE 8.4, RIGHT).
Investigation
HYPOTHESIS
The progeny of a test cross can reveal whether a parental organism is homozygous or heterozygous.
ANALYZE THE DATA
A tall plant was crossed with a short plant to produce three F1 plants (A, B, and C). Each of the F1 plants was test crossed, with the following results:

- For each test cross, what were the genotypes and phenotypes of both parents?
- Choose and perform a statistical test from Appendix B to evaluate the significance of these data.
Go to LaunchPad for discussion and relevant links for all INVESTIGATION figures.
aSee www.mendelweb.org/Mendel.plain.html
Mendel obtained results consistent with both of these predictions; thus his hypothesis accurately predicted the results of his test crosses.
Go to ACTIVITY 8.1 Homozygous or Heterozygous?
PoL2e.com/ac8.1
Mendel’s second law states that copies of different genes assort independently
Consider an organism that is heterozygous for two genes (RrYy). In this example, the dominant R and Y alleles came from one true-breeding parent, and the recessive r and y alleles came from the other true-breeding parent. When this organism produces gametes, do the R and Y alleles always go together in one gamete, and r and y alleles in another? Or can a single gamete receive one recessive and one dominant allele (R and y or r and Y)?
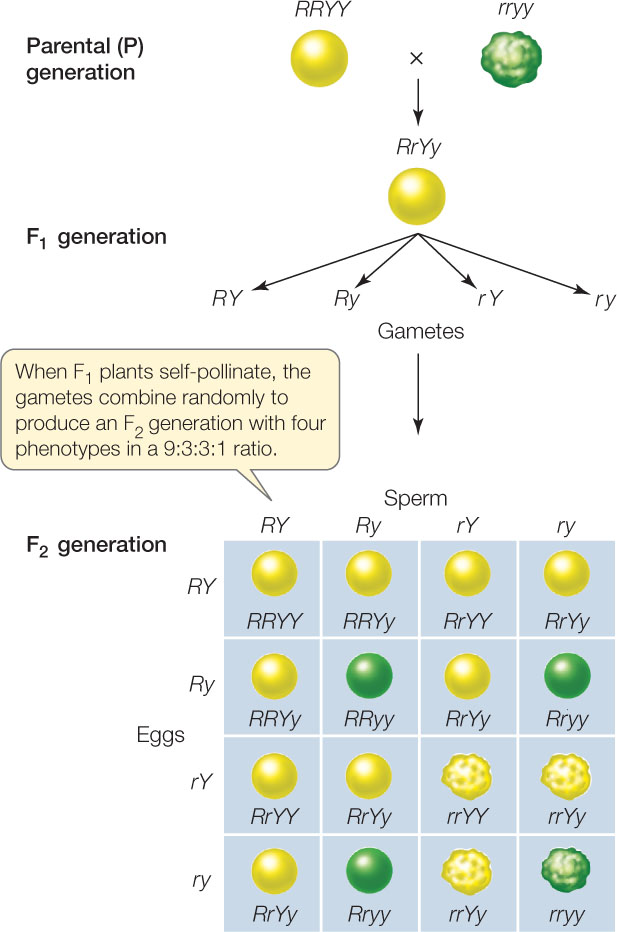
Mendel performed another series of experiments to answer these questions. He began with peas that differed in two characters: seed shape and seed color. One parental variety produced only round, yellow seeds (RRYY), and the other produced only wrinkled, green ones (rryy). A cross between these two varieties produced an F1 generation in which all the plants were RrYy. Because the R and Y alleles were dominant, the F1 seeds were all round and yellow.
155
Mendel continued this experiment into the F2 generation by performing a dihybrid cross, a cross between individuals that are identical double heterozygotes. In this case he simply allowed the F1 plants, which were all double heterozygotes, to self-pollinate (FIGURE 8.5). Depending on whether the alleles of the two genes are inherited together or separately, there are two possible outcomes, as Mendel saw:
- The alleles could maintain the associations they had in the parental generation—they could be near each other (linked) on the same chromosome. If this were the case, the F1 plants would produce two types of gametes (RY and ry). The F2 progeny resulting from self-pollination of these F1 plants would consist of two phenotypes: round yellow and wrinkled green in the ratio of 3:1, just as in the monohybrid cross.
- The segregation of R from r could be independent of the segregation of Y from y—the two genes could be unlinked, on different chromosomes. In this case, four kinds of gametes would be produced in equal numbers: RY, Ry, rY, and ry. When these gametes combine at random, they should produce an F2 having nine different genotypes. The nine genotypes would produce four phenotypes (round yellow, round green, wrinkled yellow, wrinkled green). Putting these possibilities into a Punnett square, we can predict that these four phenotypes just mentioned would occur in a ratio of 9:3:3:1.
156
Mendel’s dihybrid crosses supported the second prediction: four different phenotypes appeared in the F2 generation in a ratio of about 9:3:3:1 (see Figure 8.5). On the basis of such experiments, Mendel proposed his second law, the law of independent assortment: alleles of different genes assort independently of one another during gamete formation. In the example above, the segregation of the R and r alleles is independent of the segregation of the Y and y alleles. As you will see in Concept 8.3, this is not as universal as the law of segregation because it does not apply to genes located near one another on the same chromosome. However, it is correct to say that chromosomes segregate independently during the formation of gametes, and so do any two genes located on separate chromosome pairs (FIGURE 8.6).


Go to ANIMATED TUTORIAL 8.1 Independent Assortment of Alleles
PoL2e.com/at8.1
Probability is used to predict inheritance
One key to Mendel’s success was his use of large sample sizes. By counting many progeny from each cross, he observed clear patterns that allowed him to formulate his theories. After his work became widely recognized, geneticists began using simple probability calculations to predict the ratios of genotypes and phenotypes in the progeny of a given cross or mating. They use statistics to determine whether the actual results match the prediction.
You can think of probabilities by considering a coin toss. The basic conventions of probability are simple:
- If an event is absolutely certain to happen, its probability is 1.
- If it cannot possibly happen, its probability is 0.
- All other events have a probability between 0 and 1.
There are two possible outcomes of a coin toss, and both are equally likely, so the probability of heads is ½—as is the probability of tails.
If two coins (say a penny and a dime) are tossed, each acts independently of the other (FIGURE 8.7). What is the probability of both coins coming up heads? In half of the tosses, the penny comes up heads, and in half of that fraction, the dime comes up heads. The probability of both coins coming up heads is ½ × ½ = ¼. In general, the probability of two independent outcomes occurring together is found by multiplying the two individual probabilities. This can be applied to a monohybrid cross (see Figure 8.2). After the self-pollination of an Rr F1 plant, the probability that an F2 plant will have the genotype RR is ½ × ½ = ¼, because the chance that the sperm will have the genotype R is ½, and the chance that the egg will have the genotype R is also ½. Similarly, the probability of rr offspring is also ¼.
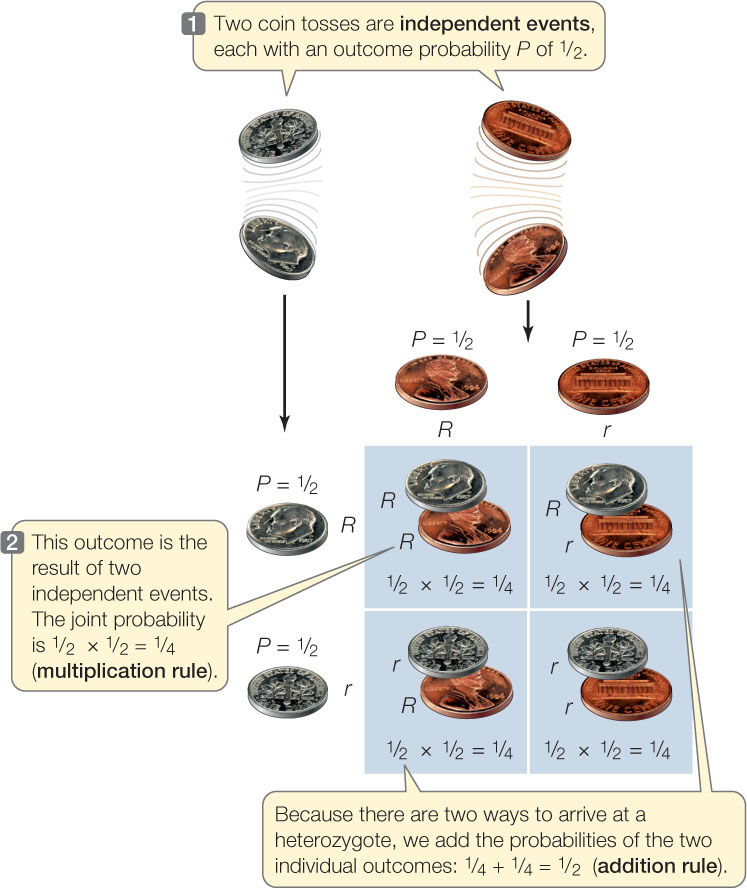
What about the probability of getting a heterozygote? As you can see in Figures 8.2 and 8.7, there are two ways to get an Rr plant or a head and a tail in a coin toss. In the case of the seed shape gene, the R allele can come from a sperm and the r from an egg (probability ¼). Or the R allele could come from the egg and the r from the sperm (probability ¼). The probability of an event that can occur in two or more different ways is the sum of the individual probabilities of those ways. Thus the probability that an F2 plant will be a heterozygote is equal to the sum of the probabilities of the two ways of forming a heterozygote: ¼ + ¼ = ½.
157
Mendel’s laws can be observed in human pedigrees
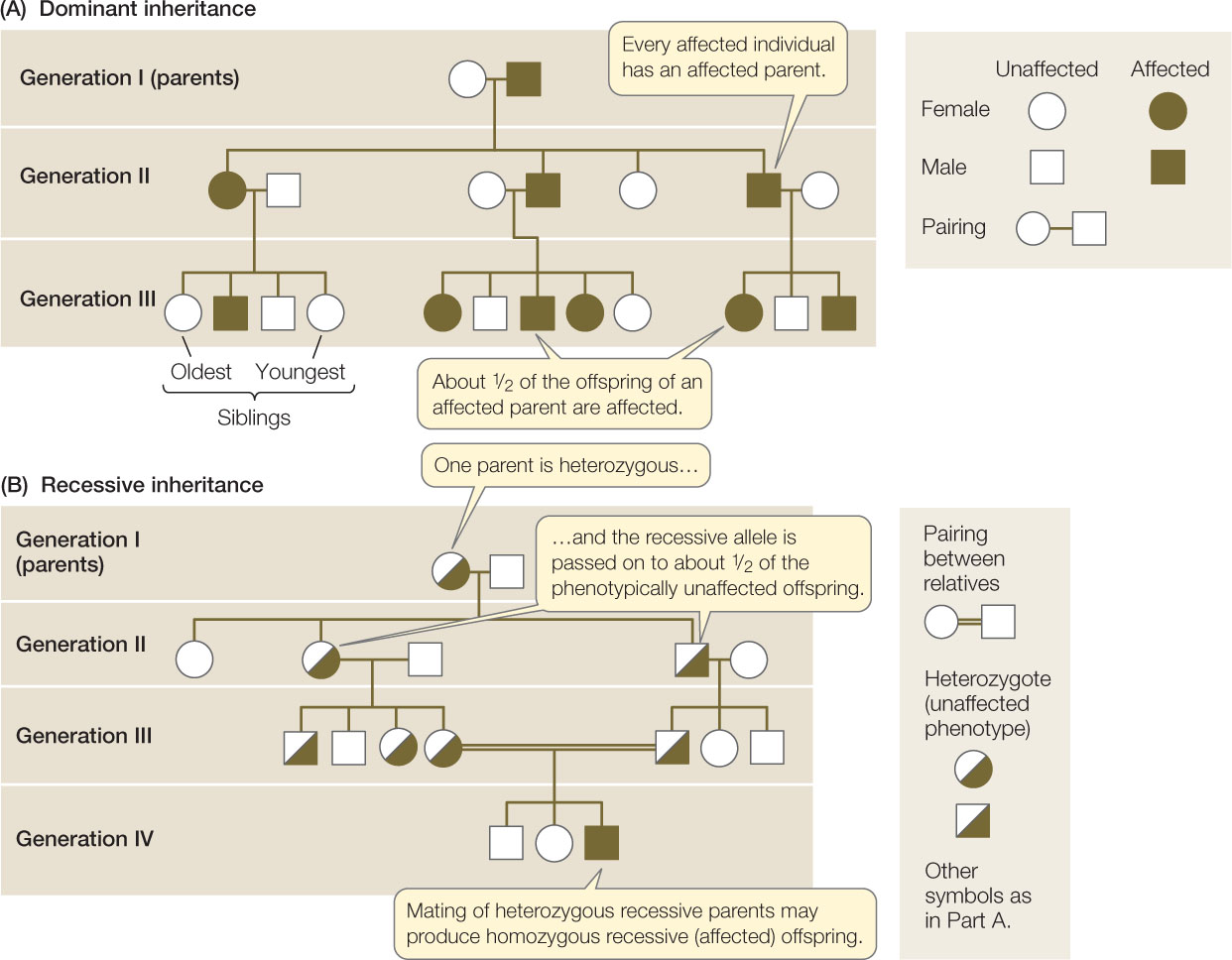
Mendel developed his theories by performing many planned crosses and counting many offspring. This approach is not possible with humans, so human geneticists rely on pedigrees: family trees that show the occurrence of inherited phenotypes in several generations of related individuals (FIGURE 8.8).
Because humans have relatively few offspring, human pedigrees do not show the clear proportions of phenotypes that Mendel saw in his pea plants. For example, when a man and a woman who are both heterozygous for a recessive allele (say, Aa) have children together, each child has a ¼ probability of being a recessive homozygote (aa). But the offspring of a single couple are likely to be too few to reliably show the one-fourth proportion. In a family with only two children, for example, both could easily be aa (or Aa, or AA).
158
Despite this limitation, pedigrees do show inheritance patterns that can provide information about the allele(s) controlling a particular phenotype. For example, it is useful to know whether a particular rare allele that causes an abnormal phenotype is dominant or recessive. Figure 8.8A is a pedigree showing the pattern of inheritance of a rare dominant allele. The following are the key features to look for in such a pedigree:
- Every person with the abnormal phenotype (affected) has an affected parent.
- Either all (in the case of a homozygous parent) or about half (in the case of a heterozygous parent) of the offspring are affected. In Figure 8.8A, each of the affected individuals in generations II and III is heterozygous for the rare dominant allele. This is clear because each person has inherited a recessive allele from his or her unaffected parent.
Compare this pattern with the one shown in Figure 8.8B, which is typical for the inheritance of a rare recessive allele:
- Affected people most often have two unaffected parents.
- Only a small proportion of people are affected: about one-fourth of children whose parents are both heterozygotes. Such parents are heterozygous carriers of the recessive allele. Unfortunately, it is not possible to use the pedigree alone to determine who else in the family may be a heterozygous carrier (that is, unless they parent a homozygous child), because the allele is recessive and shows no phenotype in the heterozygote. However, as we will see in Chapter 12, DNA sequencing or other techniques can sometimes be used to identify carriers of specific recessive alleles. This is especially important in cases where a rare allele causes serious disease.
Other patterns of inheritance can arise in special cases, such as sex-linked characters (see Concept 8.3).

Go to ANIMATED TUTORIAL 8.2 Pedigree Analysis Simulation
PoL2e.com/at8.2
CHECKpoint CONCEPT 8.1
- What are the differences between genes and alleles? Between homozygous and heterozygous conditions? Between genotype and phenotype? Between Mendel’s definition of a gene and the current definition?
- In a monohybrid cross, how do the events of meiosis explain Mendel’s first law? In a dihybrid cross, how does meiosis explain Mendel’s second law?
- Using the cross shown in Figure 8.5, calculate the probability that an F2 seed will be round and yellow.
The laws of inheritance as articulated by Mendel remain valid today, and his discoveries laid the groundwork for all future studies of genetics. However, as we will see next, the relationship of one gene to one phenotype can be complicated by interactions among alleles and among genes.