Chapter 6
13. With the assumption of homozygosity for the normal gene, the mating is A/A · b/b × a/a · B/B. The children would be normal, A/a · B/b.
16.
Red
Purple
9
M1/– ; M2/–
purple
3
m1/m1 ; M2/–
blue
3
M1/– ; m2/m2
red
1
m1/m1 ; m2/m2
white
The mutant alleles do not produce functional enzyme. However, enough functional enzyme must be produced by the single wild-
type allele of each gene to synthesize normal levels of pigment.
20.
The original cross was a dihybrid cross. Both oval and purple must represent an incomplete dominant phenotype.
A long, purple × oval, purple cross is as follows:

23.
|
|
Parents |
Child |
|
a. |
AB × O |
B |
|
b. |
A × O |
A |
|
c. |
A × AB |
AB |
|
d. |
O × O |
O |
27.
The sex ratio is expected to be 1 : 1.
The female parent was heterozygous for an X-
linked recessive lethal allele, which would result in 50% fewer males than females. Half of the female progeny should be heterozygous for the lethal allele, and half should be homozygous for the nonlethal allele. Individually mate the F1 females and determine the sex ratio of their progeny.
30.
The mutations are in two different genes because the heterokaryon is prototrophic (the two mutations complemented each other).
leu1+ ; leu2− and leu1− ; leu2+
With independent assortment, expect
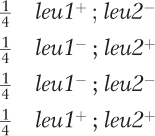
34.
P A/a (frizzle) × A/a (frizzle)
F1 1 A/A (normal) : 2 A/a (frizzle) : 1 a/a (woolly)
If A/A (normal) is crossed with a/a (woolly), all offspring will be A/a (frizzle).
36. The production of black offspring from two pure-
A/A ; b/b × a/a ; B/B
all offspring would be
A/a ; B/b
and they would have a black phenotype because of complementation.
40. The purple parent can be either A/a ; b/b or a/a ; B/b for this answer. Assume that the purple parent is A/a ; b/b. The blue parent must be A/a ; B/b.
43. The cross is gray × yellow, or A/–; R/– × A/–; r/r. The F1 progeny are
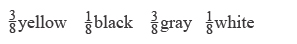
For white progeny, both parents must carry an r and an a allele. Now the cross can be rewritten as A/a ; R/r × A/a ; r/r.
46. The original brown dog is w/w ; b/b and the original white dog is W/W ; B/B. The F1 progeny are W/w ; B/b and the F2 progeny are
|
9 W/– ; B/– |
white |
|
3 w/w ; B/– |
black |
|
3 W/– ; b/b |
white |
|
1 w/w ; b/b |
brown |
50. Pedigrees such as this one are quite common. They indicate lack of penetrance due to epistasis or environmental effects. Individual A must have the dominant autosomal gene.
52.
a. Let WO = oval, WS = sickle, and WR = round. The three crosses are
|
Cross 1: |
WS/WS × WR/Y → WS/WR and WS/Y |
|
Cross 2: |
WS/WS × WS/Y → WS/WR and WR/Y |
|
Cross 3: |
WS/WS × WO/Y → WO/WS and WS/Y |
b. WO/WS × WR/Y

55.
The genotypes are
P
B/B ; i/i × b/b ; I/I
F1
B/b ; I/i
hairless
F2
9 B/– ; I/–
hairless
3 B/– ; i/i
straight
3 b/b ; I/–
hairless
1 b/b ; i/i
bent
Page 837The genotypes are B/b ; I/i × B/b ; i/i.
58. There are a total of 159 progeny that should be distributed in a 9:3:3:1 ratio if the two genes are assorting independently. You can see that
|
Observed |
Expected |
|
88 P/–; Q/– |
90 |
|
32 P/–; q/q |
30 |
|
25 p/p ; Q/– |
30 |
|
14 p/p ; q/q |
10 |
61.
Cross-
feeding is taking place, whereby a product made by one strain diffuses to another strain and allows growth of the second strain. For cross-
feeding to take place, the growing strain must have a block that is present earlier in the metabolic pathway than the block in the strain from which the growing strain is obtaining the product for growth. The data suggest that the metabolic pathway is
trpE → trpD → trpB
Without some tryptophan, there would be no growth at all, and the cells would not have lived long enough to produce a product that could diffuse
63.
The best explanation is that Marfan’s syndrome is inherited as a dominant autosomal trait.
The pedigree shows both pleiotropy (multiple affected traits) and variable expressivity (variable degree of expressed phenotype).
Pleiotropy indicates that the gene product is required in a number of different tissues, organs, or processes. When the gene is mutant, all tissues needing the gene product will be affected. Variable expressivity of a phenotype for a given genotype indicates modification by one or more other genes, random noise, or environmental effects.
66.
This type of gene interaction is called epistasis. The phenotype of e/e is epistatic to the phenotypes of B/– or b/b.
The inferred genotypes are as follows:
I
1 (B/b E/e)
2 (B/b E/e)
II
1 (b/b E/e)
2 (B/b E/e)
3 (–/– e/e)
4 (b/b E/–)
5 (B/b E/e)
6 (b/b E/e)
III
1 (B/b E/–)
2 (–/b e/e)
3 (b/b, E/–)
4 (B/b E/–)
5 (b/b E/–)
6 (B/b E/–)
7 (–/b e/e)
69.
A multiple-
allelic series has been detected: superdouble > single > double. Although the explanation for part a does rationalize all the crosses, it does not take into account either the female sterility or the origin of the superdouble plant from a double-
flowered variety.
71.
A trihybrid cross would give a 63 : 1 ratio. Therefore, there are three R loci segregating in this cross.
P
R1/R1 ; R2/R2 ; R3/R3 × r1/r1 ; r2/r2 ; r3/r3
F1
R1/r1 ; R2/r2 ; R3/r3
F2
27
R1/–; R2/–; R3/–
red
9
R1/–; R2/–; r3/r3
red
9
R1/–; r2/r2 ; R3/–
red
9
r1/r1 ; R2/–; R3/–
red
3
R1/–; r2/r2 ; r3/r3
red
3
r1/r1 ; R2/–; r3/r3
red
3
r1/r1 ; r2/r2 ; R3/–
red
1
r1/r1 ; r2/r2 ; r3/r3
white
(1) To obtain a 1 : 1 ratio, only one of the genes can be heterozygous. A representative cross is R1/r1 ; r2/r2 ; r3/r3 × r1/r1 ; r2/r2 ; r3/r3.
(2) To obtain a 3 red : 1 white ratio, two alleles must be segregating and they cannot be within the same gene. A representative cross is R1/r1 ; R2/r2 ; r3/r3 × r1/r1 ; r2/r2 ; r3/r3.
(3) To obtain a 7 red : 1 white ratio, three alleles must be segregating, and they cannot be within the same gene. The cross is R1/r1 ; R2/r2 ; R3/r3 × r1/r1 ; r2/r2 ; r3/r3.
The formula is 1 − (
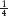 )n, where n = the number of loci that are segregating in the representative crosses in part c.
)n, where n = the number of loci that are segregating in the representative crosses in part c.
75. a. and b. Epistasis is implicated, and the homozygous recessive white genotype seems to block the production of color by a second gene.
Assume the following dominance relations: red > orange > yellow. Let the alleles be designated as follows:
|
red |
AR |
|
orange |
AO |
|
yellow |
AY |
Crosses 1 through 3 now become
|
P |
AO/AO × AY/AY |
AR/AR × AO/AO |
AR/AR × AY/AY |
|
F1 |
AO/AY |
AR/AO |
AR/AY |
|
F2 |
3 AO/– : 1 AY/AY |
3 AR/– : 1 AO/AO |
3 AR/– : 1 AY/AY |
Cross 4: To do this cross, you must add a second gene. You must also rewrite crosses 1 through 3 to include the second gene. Let B allow color expression and b block its expression, producing white. The first three crosses become
|
P |
AO/AO ; B/B × AY/AY ; B/B |
|
|
AR/AR ; B/B × AO/AO ; B/B |
|
|
AR/AR ; B/B × AY/AY ; B/B |
|
F1 |
AO/AY ; B/B |
|
|
AR/AO ; B/B |
|
|
AR/AY ; B/B |
|
F2 |
3 AO/–; B/B : 1 AY/AY ; B/B |
|
|
3 AR/–; B/B : 1 AO/AO ; B/B |
|
|
3 AR/–; B/B : 1 AY/AY ; B/B |
The fourth cross is
|
P |
AR/AR ; B/B × AR/AR ; b/b |
|
F1 |
AR/AR ; B/b |
|
F2 |
3 AR/AR ; B/– : 1AR/AR ; b/b |
Cross 5: To do this cross, note that there is no orange. Therefore, the two parents must carry the alleles for red and yellow, and the expression of red must be blocked.
|
P |
AY/AY ; B/B× AR/AR ; b/b |
|
|
F1 |
AR/AY ; B/b |
|
|
F2 |
9 AR/–; B/– |
red |
|
|
3 AR/–; b/b |
white |
|
|
3 AY/AY ; B/– |
yellow |
|
|
1 AY/AY ; b/b |
white |
Cross 6: This cross is identical with cross 5 except that orange replaces yellow.
|
P |
AO/AO ; B/B× AR/AR ; b/b |
|
|
F1 |
AR/AO ; B/b |
|
|
F2 |
9 AR/–; B/– |
red |
|
|
3 AR/–; b/b |
white |
|
|
3 AO/AO ; B/– |
orange |
|
|
1 AO/AO ; b/b |
white |
Cross 7: In this cross, yellow is suppressed by b/b.
|
P |
AR/AR ; B/B× AY/AY ; b/b |
|
|
F1 |
AR/AY ; B/b |
|
|
F2 |
9 AR/−; B/− |
red |
|
|
3 AR/−; b/b |
white |
|
|
3 AY/AY ; B/− |
yellow |
|
|
1 AY/AY ; b/b |
white |
77.
Intercrossing mutant strains that all have a common recessive phenotype is the basis of the complementation test. This test is designed to identify the number of different genes that can mutate to a particular phenotype. In this problem, if the progeny of a given cross still express the wiggle phenotype, the mutations fail to complement and are considered alleles of the same gene; if the progeny are wild type, the mutations complement and the two strains carry mutant alleles of separate genes.
These data identify five complementation groups (genes).
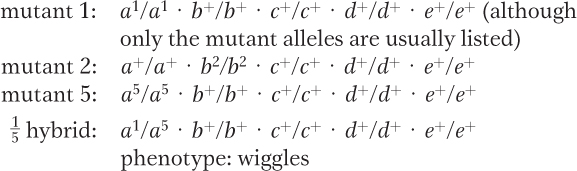
Conclusion: 1 and 5 are both mutant for gene A.
(The relevant cross a+/a+ · b2/b2 × a2/a2 · b5/b5 gives the following hybrid.)
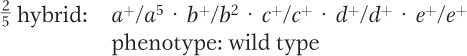
Conclusion: 2 and 5 are mutant for different genes.