Determining the Money Supply
Without banks, there would be no checkable deposits, and so the quantity of currency in circulation would equal the money supply. In that case, the money supply would be determined solely by whoever controls government minting and printing presses. But banks do exist, and through their creation of checkable bank deposits, they affect the money supply in two ways. First, banks remove some currency from circulation: dollar bills that are sitting in bank vaults, as opposed to sitting in people’s wallets, aren’t part of the money supply. Second, and much more importantly, banks create money by accepting deposits and making loans—
AP® Exam Tip
The money that banks create is recorded in accounts. Banks do not print money—
How Banks Create Money

To see how banks create money, let’s examine what happens when someone decides to deposit currency in a bank. Consider the example of Silas, a miser, who keeps a shoebox full of cash under his bed. Suppose Silas realizes that it would be safer, as well as more convenient, to deposit that cash in the bank and to use his debit card when shopping. Assume that he deposits $1,000 into a checkable account at First Street Bank. What effect will Silas’s actions have on the money supply?
Panel (a) of Figure 25.3 shows the initial effect of his deposit. First Street Bank credits Silas with $1,000 in his account, so the economy’s checkable bank deposits rise by $1,000. Meanwhile, Silas’s cash goes into the vault, raising First Street’s reserves by $1,000 as well.
| Figure 25.3 | Effect on the Money Supply of Turning Cash into a Checkable Deposit at First Street Bank |
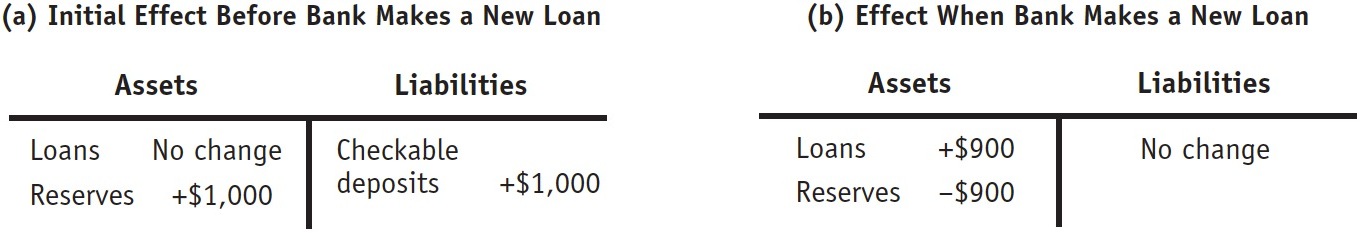
This initial transaction has no effect on the money supply. Currency in circulation, part of the money supply, falls by $1,000; checkable bank deposits, also part of the money supply, rise by the same amount.
But this is not the end of the story because First Street Bank can now lend out part of Silas’s deposit. Assume that it holds 10% of Silas’s deposit—
This may not be the end of the story either. Suppose that Mary uses her cash to buy a television and a Blu-
Assume that Second Street Bank, like First Street Bank, keeps 10% of any bank deposit in reserves and lends out the rest. Then it will keep $90 in reserves and lend out $810 of Anne’s deposit to another borrower, further increasing the money supply.
Table 25.1 shows the process of money creation we have described so far. At first the money supply consists only of Silas’s $1,000. After he deposits the cash into a checkable bank deposit and the bank makes a loan, the money supply rises to $1,900. After the second deposit and the second loan, the money supply rises to $2,710. And the process will, of course, continue from there. (Although we have considered the case in which Silas places his cash in a checkable bank deposit, the results would be the same if he put it into any type of near-
Table 25.1How Banks Create Money
| Currency in circulation | Checkable bank deposits | Money supply | |
| First stage: Silas keeps his cash under his bed. |
$1,000 | $0 | $1,000 |
| Second stage: Silas deposits cash in First Street Bank, which lends out $900 to Mary, who then pays it to Anne Acme. |
900 | 1,000 | 1,900 |
| Third stage: Anne Acme deposits $900 in Second Street Bank, which lends out $810 to another borrower. |
810 | 1,900 | 2,710 |
This process of money creation may sound familiar. Recall the spending multiplier process that we described in Module 16: an initial increase in spending leads to a rise in real GDP, which leads to a further rise in spending, which leads to a further rise in real GDP, and so on. What we have here is another kind of multiplier—
Reserves, Bank Deposits, and the Money Multiplier
Excess reserves are a bank’s reserves over and above its required reserves.
In tracing out the effect of Silas’s deposit in Table 25.1, we assumed that the funds a bank lends out always end up being deposited either in the same bank or in another bank—
AP® Exam Tip
Excess reserves are important because these reserves are what banks can loan out to borrowers.
Now suppose that for some reason a bank suddenly finds itself with $1,000 in excess reserves. What happens? The answer is that the bank will lend out that $1,000, which will end up as a checkable bank deposit somewhere in the banking system, launching a money multiplier process very similar to the process shown in Table 25.1. In the first stage, the bank lends out its excess reserves of $1,000, which becomes a checkable bank deposit somewhere. The bank that receives the $1,000 deposit keeps 10%, or $100, as reserves and lends out the remaining 90%, or $900, which again becomes a checkable bank deposit somewhere. The bank receiving this $900 deposit again keeps 10%, which is $90, as reserves and lends out the remaining $810. The bank receiving this $810 keeps $81 in reserves and lends out the remaining $729, and so on. As a result of this process, the total increase in checkable bank deposits is equal to a sum that looks like:
$1,000 + $900 + $810 + $729 + . . .
We’ll use the symbol rr for the reserve ratio. More generally, the total increase in checkable bank deposits that is generated when a bank lends out $1,000 in excess reserves is:
(25-
As we have seen, an infinite series of this form can be simplified to $1,000/rr. We must introduce another term before formally defining the money multiplier, but we can now see its usefulness: it is the factor by which we multiply an initial increase in excess reserves to find the total resulting increase in checkable bank deposits:
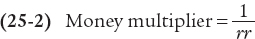
AP® Exam Tip
The money multiplier is 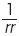 where rr is the reserve ratio. Be sure to know this frequently tested formula.
where rr is the reserve ratio. Be sure to know this frequently tested formula.
Given a reserve ratio of 10%, or 0.1, a $1,000 increase in excess reserves will increase the total value of checkable bank deposits by $1,000 × 1/rr = $1,000/0.1 = $10,000. In fact, in a checkable-
The Money Multiplier in Reality
In reality, the determination of the money supply is more complicated than our simple model suggests because it depends not only on the ratio of reserves to bank deposits but also on the fraction of the money supply that individuals choose to hold in the form of currency. In fact, we already saw this in our example of Silas depositing the cash instead of holding it under his bed: when he chose to hold a checkable bank deposit instead of currency, he set in motion an increase in the money supply.
The monetary base is the sum of currency in circulation and bank reserves.

To define the money multiplier in practice, we need to understand that the Federal Reserve controls the monetary base, the sum of currency in circulation and the reserves held by banks. The Federal Reserve does not determine how that sum is allocated between bank reserves and currency in circulation. Consider Silas and his deposit one more time: by taking the cash from under his bed and depositing it in a bank, he reduced the quantity of currency in circulation but increased bank reserves by an equal amount. So while the allocation of the monetary base changes—
The monetary base is different from the money supply in two ways. First, bank reserves, which are part of the monetary base, aren’t considered part of the money supply. A $1 bill in someone’s wallet is considered money because it’s available for an individual to spend, but a $1 bill held as bank reserves in a bank vault or deposited at the Federal Reserve isn’t considered part of the money supply because it’s not available for spending. Second, checkable bank deposits, which are part of the money supply because they are available for spending, aren’t part of the monetary base.
Figure 25.4 shows the two concepts schematically. The circle on the left represents the monetary base, consisting of bank reserves plus currency in circulation. The circle on the right represents the money supply, consisting mainly of currency in circulation plus checkable or near-
| Figure 25.4 | The Monetary Base and the Money Supply |
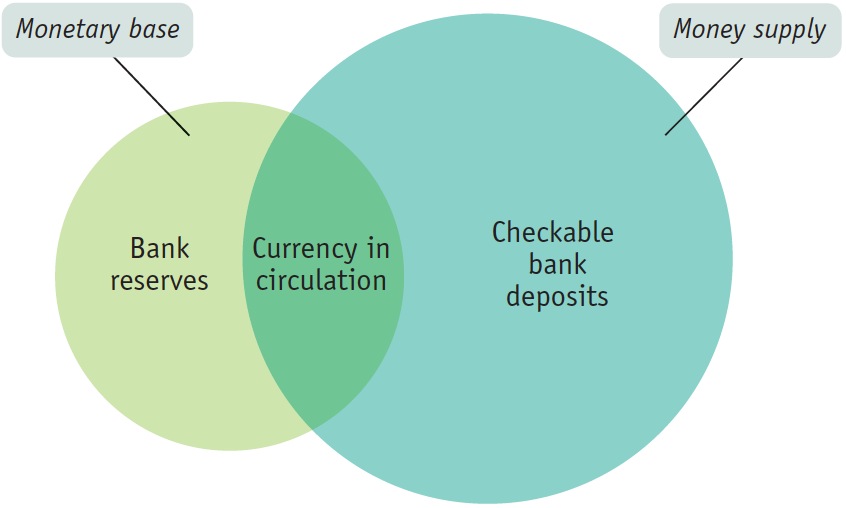
The money multiplier is the ratio of the money supply to the monetary base. It indicates the total number of dollars created in the banking system by each $1 addition to the monetary base.
Now we can formally define the money multiplier: it’s the ratio of the money supply to the monetary base. Most importantly, this tells us the total number of dollars created in the banking system by each $1 addition to the monetary base. We have seen that in a simple situation in which banks hold no excess reserves and all cash is deposited in banks, the money multiplier is 1/rr. So if the reserve requirement is 0.1 (the minimum required ratio for most checkable deposits in the United States), the money multiplier is 1/0.1 = 10; if the Federal Reserve adds $100 to the monetary base, the money supply will increase by 10 × $100 = $1,000. During normal times, the actual money multiplier in the United States, using M1 as our measure of money, is about 1.9. That’s a lot smaller than 10. Normally, the reason the actual money multiplier is so small arises from the fact that people hold significant amounts of cash, and a dollar of currency in circulation, unlike a dollar in reserves, doesn’t support multiple dollars of the money supply. In fact, currency in circulation normally accounts for more than 90% of the monetary base.