Tackle the Test: Free-Response Questions

1. Question

   Refer to the graph provided. Assume the government has imposed an excise tax of $60 on producers in this market.

   

   
   

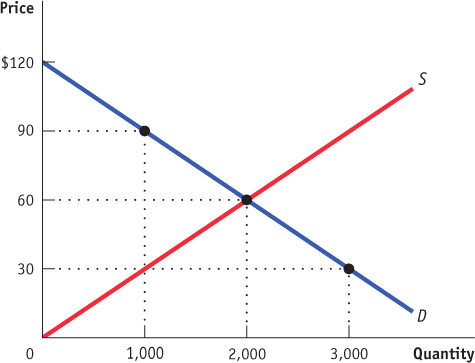
   1. What quantity will be sold in the market?

      
      
   
   

   2. What price will consumers pay in the market?

      
      
   
   

   3. By how much will consumer surplus change as a result of the tax?

      
      
   
   

   4. By how much will producer surplus change as a result of the tax?

      
      
   
   

   5. How much revenue will the government collect from this excise tax?

      
      
   
   

   6. Calculate the deadweight loss created by the tax.

      
      
   
   

   Rubric for FRQ 1 (6 points)

   1 point: 1,000

   1 point: $90

   1 point: Consumer surplus will decrease by $45,000, from ½ ($2,000 × 60) = $60,000 before the tax to ½ ($1,000 × 30) = $15,000 after the tax.

   1 point: Producer surplus will decrease by $45,000, from ½ ($2,000 × 60) = $60,000 before the tax to ½ ($1,000 × 30) = $15,000 after the tax.

   1 point: $60 × 1,000 = $60,000

   1 point: ½ × $60 × 1,000 = $30,000

2. Question

   Draw a correctly labeled graph of a competitive market in equilibrium. Use your graph to illustrate the effect of an excise tax imposed on consumers. Indicate each of the following on your graph:

   
   

   
   

   1. the equilibrium price and quantity without the tax, labeled P_E and Q_E

      
      
   
   

   2. the quantity sold in the market post-tax, labeled Q_T

      
      
   
   

   3. the price paid by consumers post-tax, labeled P_C

      
      
   
   

   4. the price received by producers post-tax, labeled P_P

      
      
   
   

   5. the tax revenue generated by the tax, labeled “Tax revenue”

      
      
   
   

   6. the deadweight loss resulting from the tax, labeled “DWL” (7 points)

      
      
   
   

   Rubric for FRQ 2 (7 points)

   

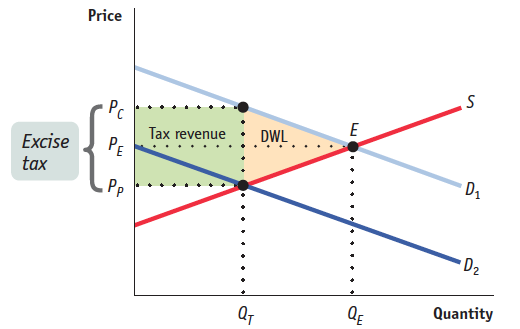
   1 point: A graph with “Price” (or P ) on the vertical axis, “Quantity” (or Q ) on the horizontal axis, a downward-sloping demand curve, and an upward-sloping supply curve

   1 point: P_E and Q_E labeled along the appropriate axes at the equilibrium levels

   1 point: Post-tax quantity labeled Q_T

   1 point: Post-tax consumer price labeled P_c

   1 point: Post-tax producer price labeled P_p

   1 point: Tax revenue area labeled “Tax revenue”

   1 point: Deadweight loss area labeled “DWL”