Problems
1.Hiro owns and operates a small business that provides economic consulting services. During the year he spends $57,000 on travel to clients and other expenses. In addition, he owns a computer that he uses for business. If he didn’t use the computer, he could sell it and earn yearly interest of $100 on the money created through this sale. Hiro’s total revenue for the year is $100,000. Instead of working as a consultant for the year, he could teach economics at a small local college and make a salary of $50,000.
- a. What is Hiro’s accounting profit?
- b. What is Hiro’s economic profit?
- c. Should Hiro continue working as a consultant, or should he teach economics instead?
2.Jackie owns and operates a web-
- a. How much total revenue does Jackie need to make in order to break even in the eyes of her accountant? That is, how much total revenue would give Jackie an accounting profit of just zero?
- b. How much total revenue does Jackie need to make in order for her to want to remain self-
employed? That is, how much total revenue would give Jackie an economic profit of just zero?
3.You own and operate a bike store. Each year, you receive revenue of $200,000 from your bike sales, and it costs you $100,000 to obtain the bikes. In addition, you pay $20,000 for electricity, taxes, and other expenses per year. Instead of running the bike store, you could become an accountant and receive a yearly salary of $40,000. A large clothing retail chain wants to expand and offers to rent the store from you for $50,000 per year. How do you explain to your friends that despite making a profit, it is too costly for you to continue running your store?
4.Suppose you have just paid a nonrefundable fee of $1,000 for your meal plan for this academic term. This allows you to eat dinner in the cafeteria every evening.
- a. You are offered a part-
time job in a restaurant where you can eat for free each evening. Your parents say that you should eat dinner in the cafeteria anyway, since you have already paid for those meals. Are your parents right? Explain why or why not. - b. You are offered a part-
time job in a different restaurant where, rather than being able to eat for free, you receive only a large discount on your meals. Each meal there will cost you $2; if you eat there each evening this semester, it will add up to $200. Your roommate says that you should eat in the restaurant since it costs less than the $1,000 that you paid for the meal plan. Is your roommate right? Explain why or why not.
5.You have bought a $10 ticket in advance for the college soccer game, a ticket that cannot be resold. You know that going to the soccer game will give you a benefit equal to $20. After you have bought the ticket, you hear that there will be a professional baseball post-
6.Amy, Bill, and Carla all mow lawns for money. Each of them operates a different lawn mower. The accompanying table shows the total cost to Amy, Bill, and Carla of mowing lawns.
| Quantity of lawns mowed | Amy’s total cost | Bill’s total cost | Carla’s total cost |
|---|---|---|---|
| 0 | $0 | $0 | $0 |
| 1 | 20 | 10 | 2 |
| 2 | 35 | 20 | 7 |
| 3 | 45 | 30 | 17 |
| 4 | 50 | 40 | 32 |
| 5 | 52 | 50 | 52 |
| 6 | 53 | 60 | 82 |
- a. Calculate Amy’s, Bill’s, and Carla’s marginal costs, and draw each of their marginal cost curves.
- b. Who has increasing marginal cost, who has decreasing marginal cost, and who has constant marginal cost?
7.You are the manager of a gym, and you have to decide how many customers to admit each hour. Assume that each customer stays exactly one hour. Customers are costly to admit because they inflict wear and tear on the exercise equipment. Moreover, each additional customer generates more wear and tear than the customer before. As a result, the gym faces increasing marginal cost. The accompanying table shows the marginal costs associated with each number of customers per hour.

- a. Suppose that each customer pays $15.25 for a one-
hour workout. Use the profit- maximizing principle of marginal analysis to find the optimal number of customers that you should admit per hour. - b. You increase the price of a one-
hour workout to $16.25. What is the optimal number of customers per hour that you should admit now?
8.Georgia and Lauren are economics students who go to a karate class together. Both have to choose how many classes to go to per week. Each class costs $20. The accompanying table shows Georgia’s and Lauren’s estimates of the marginal benefit that each of them gets from each class per week.
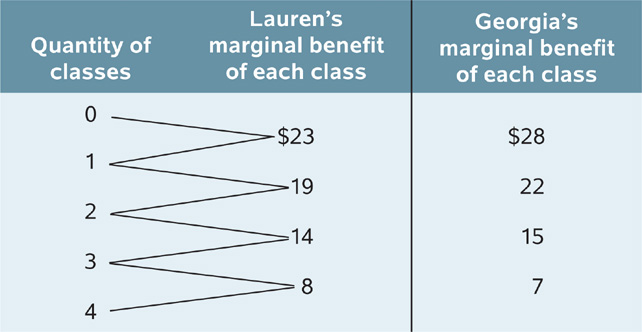
- a. Use marginal analysis to find Lauren’s optimal number of karate classes per week. Explain your answer.
- b. Use marginal analysis to find Georgia’s optimal number of karate classes per week. Explain your answer.
9.The Centers for Disease Control and Prevention (CDC) recommended against vaccinating the whole population against the smallpox virus because the vaccination has undesirable, and sometimes fatal, side effects. Suppose the accompanying table gives the data that are available about the effects of a smallpox vaccination program.
| Percent of population vaccinated | Deaths due to smallpox | Deaths due to vaccination side effects |
|---|---|---|
| 0% | 200 | 0 |
| 10 | 180 | 4 |
| 20 | 160 | 10 |
| 30 | 140 | 18 |
| 40 | 120 | 33 |
| 50 | 100 | 50 |
| 60 | 80 | 74 |
- a. Calculate the marginal benefit (in terms of lives saved) and the marginal cost (in terms of lives lost) of each 10% increment of smallpox vaccination. Calculate the net increase in human lives for each 10% increment in population vaccinated.
- b. Using marginal analysis, determine the optimal percentage of the population that should be vaccinated.
10.Patty delivers pizza using her own car, and she is paid according to the number of pizzas she delivers. The accompanying table shows Patty’s total benefit and total cost when she works a specific number of hours.
| Quantity of hours worked | Total benefit | Total cost |
|---|---|---|
| 0 | $0 | $0 |
| 1 | 30 | 10 |
| 2 | 55 | 21 |
| 3 | 75 | 34 |
| 4 | 90 | 50 |
| 5 | 100 | 70 |
- a. Use marginal analysis to determine Patty’s optimal number of hours worked.
- b. Calculate the total profit to Patty from working 0 hours, 1 hour, 2 hours, and so on. Now suppose Patty chooses to work for 1 hour. Compare her total profit from working for 1 hour with her total profit from working the optimal number of hours. How much would she lose by working for only 1 hour?
11.Assume De Beers is the sole producer of diamonds. When it wants to sell more diamonds, it must lower its price in order to induce shoppers to buy more. Furthermore, each additional diamond that is produced costs more than the previous one due to the difficulty of mining for diamonds. De Beers’s total benefit schedule is given in the accompanying table, along with its total cost schedule.
| Quantity of diamonds | Total benefit | Total cost |
|---|---|---|
| 0 | $0 | $0 |
| 1 | 1,000 | 50 |
| 2 | 1,900 | 100 |
| 3 | 2,700 | 200 |
| 4 | 3,400 | 400 |
| 5 | 4,000 | 800 |
| 6 | 4,500 | 1,500 |
| 7 | 4,900 | 2,500 |
| 8 | 5,200 | 3,800 |
- a. Draw the marginal cost curve and the marginal benefit curve and, from your diagram, graphically derive the optimal quantity of diamonds to produce.
- b. Calculate the total profit to De Beers from producing each quantity of diamonds. Which quantity gives De Beers the highest total profit?
12.In each of the following examples, explain whether the decision is rational or irrational. Describe the type of behavior exhibited.
- a. Rick has just gotten his teaching degree and has two job offers. One job, replacing a teacher who has gone on leave, will last only two years. It is at a prestigious high school, and he will be paid $35,000 per year. He thinks he will probably be able to find another good job in the area after the two years are up but isn’t sure. The other job, also at a high school, pays $25,000 per year and is virtually guaranteed for five years; after those five years, he will be evaluated for a permanent teaching position at the school. About 75% of the teachers who start at the school are hired for permanent positions. Rick takes the five-
year position at $25,000 per year. - b. Kimora has planned a trip to Florida during spring break in March. She has several school projects due after her return. Rather than do them in February, she figures she can take her books with her to Florida and complete her projects there.
- c. Sahir overpaid when buying a used car that has turned out to be a lemon. He could sell it for parts, but instead he lets it sit in his garage and deteriorate.
- d. Barry considers himself an excellent investor in stocks. He selects new stocks by finding ones with characteristics similar to those of his previous winning stocks. He chocks up losing trades to ups and downs in the macroeconomy.
13.You have been hired as a consultant by a company to develop the company’s retirement plan, taking into account different types of predictably irrational behavior commonly displayed by employees. State at least two types of irrational behavior employees might display with regard to the retirement plan and the steps you would take to forestall such behavior.
14.For each of the following situations, decide whether Al has increasing, constant, or diminishing marginal utility.
- a. The more economics classes Al takes, the more he enjoys the subject. And the more classes he takes, the easier each one gets, making him enjoy each additional class even more than the one before.
- b. Al likes loud music. In fact, according to him, “the louder, the better.” Each time he turns the volume up a notch, he adds 5 utils to his total utility.
- c. Al enjoys watching reruns of the old sitcom Friends. He claims that these episodes are always funny, but he does admit that the more times he sees an episode, the less funny it gets.
- d. Al loves toasted marshmallows. The more he eats, however, the fuller he gets and the less he enjoys each additional marshmallow. And there is a point at which he becomes satiated: beyond that point, more marshmallows actually make him feel worse rather than better.
15.Use the concept of marginal utility to explain the following: Newspaper vending machines are designed so that once you have paid for one paper, you could take more than one paper at a time. But soda vending machines, once you have paid for one soda, dispense only one soda at a time.
16.Brenda likes to have bagels and coffee for breakfast. The accompanying table shows Brenda’s total utility from various consumption bundles of bagels and coffee.
| Consumption bundle | ||
|---|---|---|
| Quantity of bagels | Quantity of coffee (cups) | Total utility (utils) |
| 0 | 0 | 0 |
| 0 | 2 | 28 |
| 0 | 4 | 40 |
| 1 | 2 | 48 |
| 1 | 3 | 54 |
| 2 | 0 | 28 |
| 2 | 2 | 56 |
| 3 | 1 | 54 |
| 3 | 2 | 62 |
| 4 | 0 | 40 |
| 4 | 2 | 66 |
Suppose Brenda knows she will consume 2 cups of coffee for sure. However, she can choose to consume different quantities of bagels: she can choose either 0, 1, 2, 3, or 4 bagels.
- a. Calculate Brenda’s marginal utility from bagels as she goes from consuming 0 bagel to 1 bagel, from 1 bagel to 2 bagels, from 2 bagels to 3 bagels, and from 3 bagels to 4 bagels.
- b. Draw Brenda’s marginal utility curve of bagels. Does Brenda have increasing, diminishing, or constant marginal utility of bagels?
17.Bernie loves notebooks and Beyoncé CDs. The accompanying table shows the utility Bernie receives from each product.
| Quantity of notebooks | Utility from notebooks (utils) | Quantity of CDs | Utility from CDs (utils) |
|---|---|---|---|
| 0 | 0 | 0 | 0 |
| 2 | 70 | 1 | 80 |
| 4 | 130 | 2 | 150 |
| 6 | 180 | 3 | 210 |
| 8 | 220 | 4 | 260 |
| 10 | 250 | 5 | 300 |
The price of a notebook is $5, the price of a CD is $10, and Bernie has $50 of income to spend.
- a. Which consumption bundles of notebooks and CDs can Bernie consume if he spends all his income? Illustrate Bernie’s budget line with a diagram, putting notebooks on the horizontal axis and CDs on the vertical axis.
- b. Calculate the marginal utility of each notebook and the marginal utility of each CD. Then calculate the marginal utility per dollar spent on notebooks and the marginal utility per dollar spent on CDs.
- c. Draw a diagram like Figure 20-4 in which both the marginal utility per dollar spent on notebooks and the marginal utility per dollar spent on CDs are illustrated. Using this diagram and the optimal consumption rule, predict which bundle—
from all the bundles on his budget line— Bernie will choose.
18.For each of the following situations, decide whether the bundle Lakshani is considering is optimal or not. If it is not optimal, how could Lakshani improve her overall level of utility? That is, determine which good she should spend more on and which good she should spend less on.
- a. Lakshani has $200 to spend on sneakers and sweaters. Sneakers cost $50 per pair, and sweaters cost $20 each. She is thinking about buying 2 pairs of sneakers and 5 sweaters. She tells her friend that the additional utility she would get from the second pair of sneakers is the same as the additional utility she would get from the fifth sweater.
- b. Lakshani has $5 to spend on pens and pencils. Each pen costs $0.50 and each pencil costs $0.10. She is thinking about buying 6 pens and 20 pencils. The last pen would add five times as much to her total utility as the last pencil.
- c. Lakshani has $50 per season to spend on tickets to football games and tickets to soccer games. Each football ticket costs $10, and each soccer ticket costs $5. She is thinking about buying 3 football tickets and 2 soccer tickets. Her marginal utility from the third football ticket is twice as much as her marginal utility from the second soccer ticket.
