The quantity theory is based on a simple money demand function: it assumes that the demand for real money balances is proportional to income. The quantity theory is a good place to start when analyzing the effects of money on the economy, but it is not the whole story. Here we add another determinant of the quantity of money demanded—the nominal interest rate.

The money you hold in your wallet does not earn interest. If, instead of holding that money, you used it to buy government bonds or deposited it in a savings account, you would earn the nominal interest rate. Therefore, the nominal interest rate is the opportunity cost of holding money: it is what you give up by holding money rather than bonds.

Another way to see that the cost of holding money equals the nominal interest rate is by comparing the real returns on alternative assets. Assets other than money, such as government bonds, earn the real return r. Money earns an expected real return of −Eπ, because its real value declines at the rate of inflation. When you hold money, you give up the difference between these two returns. Thus, the cost of holding money is r − (−Eπ), which the Fisher equation tells us is the nominal interest rate i.

Just as the quantity of bread demanded depends on the price of bread, the quantity of money demanded depends on the price of holding money. Hence, the demand for real money balances depends both on the level of income and on the nominal interest rate. We write the general money demand function as

(M/P)^d = L(i, Y).

The letter L is used to denote money demand because money is the economy’s most liquid asset (the asset most easily used to make transactions). This equation states that the demand for the liquidity of real money balances is a function of income and the nominal interest rate. The higher the level of income Y, the greater the demand for real money balances. The higher the nominal interest rate i, the lower the demand for real money balances.

119

Money, prices, and interest rates are now related in several ways. Figure 5-5 illustrates the linkages we have discussed. As the quantity theory of money explains, money supply and money demand together determine the equilibrium price level. Changes in the price level are, by definition, the rate of inflation. Inflation, in turn, affects the nominal interest rate through the Fisher effect. But now, because the nominal interest rate is the cost of holding money, the nominal interest rate feeds back to affect the demand for money.

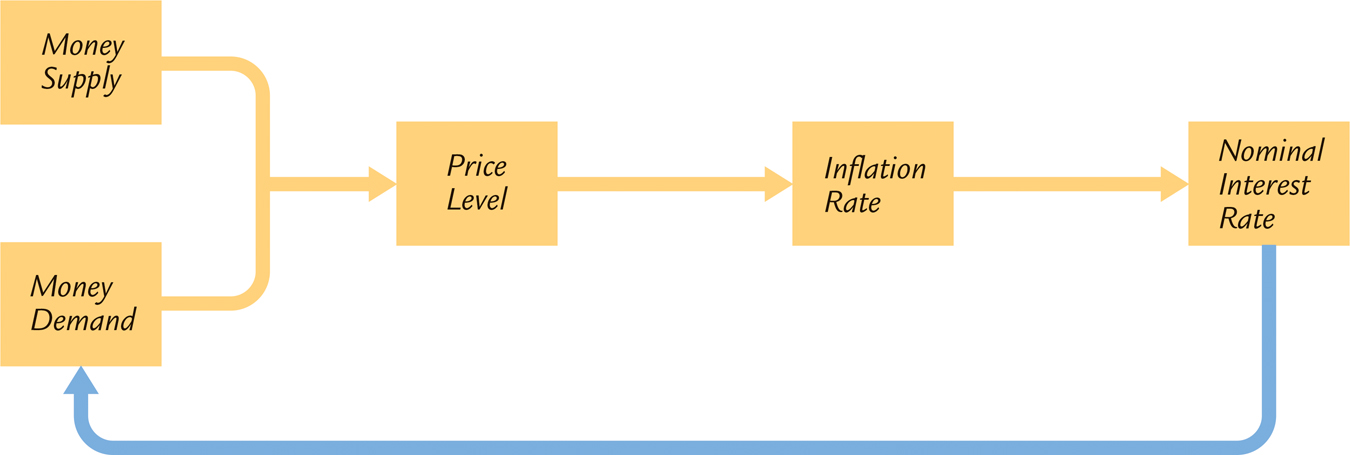
Consider how the introduction of this last link affects our theory of the price level. First, equate the supply of real money balances M/P to the demand L(i, Y):

M/P = L(i, Y).

Next, use the Fisher equation to write the nominal interest rate as the sum of the real interest rate and expected inflation:

M/P = L(r + Eπ, Y).

This equation states that the level of real money balances depends on the expected rate of inflation.

The last equation tells a more sophisticated story about the determination of the price level than does the quantity theory. The quantity theory of money says that today’s money supply determines today’s price level. This conclusion remains partly true: if the nominal interest rate and the level of output are held constant, the price level moves proportionately with the money supply. Yet the nominal interest rate is not constant; it depends on expected inflation, which in turn depends on growth in the money supply. The presence of the nominal interest rate in the money demand function yields an additional channel through which money supply affects the price level.

120

This general money demand equation implies that the price level depends not only on today’s money supply but also on the money supply expected in the future. To see why, suppose the Fed announces that it will increase the money supply in the future, but it does not change the money supply today. This announcement causes people to expect higher money growth and higher inflation. Through the Fisher effect, this increase in expected inflation raises the nominal interest rate. The higher nominal interest rate increases the cost of holding money and therefore reduces the demand for real money balances. Because the Fed has not changed the quantity of money available today, the reduced demand for real money balances leads to a higher price level. Hence, expectations of higher money growth in the future lead to a higher price level today.

The effect of money on prices is complex. The appendix to this chapter presents the Cagan model, which shows how the price level is related to current and expected future monetary policy. In particular, the analysis concludes that the price level depends on a weighted average of the current money supply and the money supply expected to prevail in the future.