A.4 Proportions: Fractions, Decimals, and Percentages
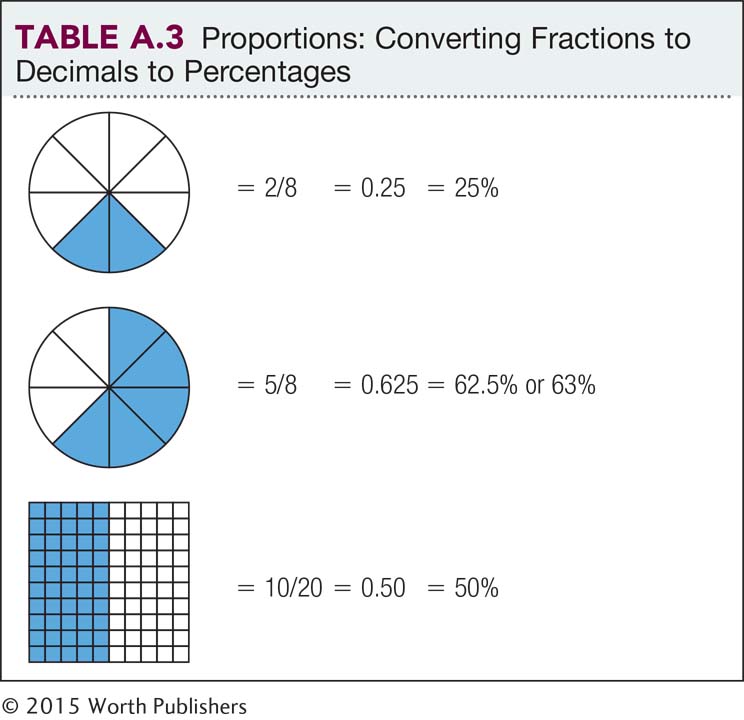
A proportion is a part in relation to a whole. When we look at fractions, we understand the denominator (the bottom number) to be the number of equal parts that comprise the whole. The numerator represents the proportion of parts of that whole that are present. Fractions can be converted into decimals by dividing the numerator by the denominator. Decimals can then be converted into percentages by multiplying by 100 (Table A.3). It is important to use the percentage symbol (%) when differentiating decimals from percentages. Additionally, decimals are often rounded to the nearest hundredth before they are converted into a percentage.
FRACTIONS
Equivalent Fractions
The same proportion can be expressed in a number of equivalent fractions. Equivalent fractions are found by multiplying both the numerator and the denominator by the same number.
1/2 = 2/4 = 6/12 = 30/60
In this case, we multiply each side of 1/2 by 2 to reach the equivalent 2/4, then by 3 to reach the equivalent 6/12, then by 5 to reach the equivalent 30/60. Or we could have multiplied the numerator and denominator of the original 1/2 by 30 to reach our concluding 30/60.
Fractions can also be reduced to a simpler form by dividing the numerator and denominator by the same number. Be sure to divide each by a number that will result in a whole number for both the numerator and the denominator.
25/75 = 5/15 = 1/3
By dividing each side by 5, the fraction was reduced from 25/75 to 5/15. By further dividing by 5, we reduce the fraction to its simplest form, 1/3. Or we could have divided the numerator and denominator of the original 25/75 by 25, resulting in the simplest expression of this fraction, 1/3.
Adding and Subtracting Fractions (with the same denominator)
Finding equivalent fractions is essential to adding and subtracting two or more fractions. In order to add or subtract, each fraction must have the same denominator. If the two fractions already have the same denominator, add or subtract the numbers in the numerators only.
2/7 + 1/7 = 3/7 4/5 − 3/5 = 1/5
In each of these instances, we are adding or subtracting from the same whole (or same pie, as in lines 1 and 2 of Table A.3). In the first equation, we are increasing our proportion of 2 by 1 to equal 3 pieces of the whole. In the second equation, we are reducing the number of proportions from 4 by 3 to equal just 1 piece of the whole.
Adding and Subtracting Fractions (with different denominators)
When adding or subtracting two proportions with different denominators, it is necessary to find a common denominator before performing the operation. It is often easiest to multiply each side (numerator and denominator) by the number equal to the denominator of the other fraction. This provides an easy route to finding a common denominator.
2/5 + 1/6 =
Multiply the numerator and denominator of 2/5 by 6, equaling 12/30.
Multiply the numerator and denominator of 1/6 by 5, equaling 5/30.
12/30 + 5/30 = 17/30
Multiplying Fractions
When multiplying fractions, it is not necessary to find common denominators. Just multiply the two numerators in each fraction and the two denominators in each fraction.
4/7 × 5/8 = (4 × 5) / (7 × 8) = 20/56
(Note: This fraction can be reduced to a simpler equivalent by dividing both the numerator and denominator by 4. The result is 5/14.)
Dividing Fractions
When dividing a fraction by another fraction, invert the second fraction and multiply as above.
1/3 ÷ 2/3 = 1/3 × 3/2 = (1 × 3) / (3 × 2) = 3/6
(Note: This can be reduced to a simpler equivalent by dividing both the numerator and denominator by 3. The result is one-
SELF-QUIZ #3: Fractions
(Answers to this quiz can be found on page A-
2/5 + 1/5 =
2/7 × 4/5 =
11/15 − 2/5 =
3/5 ÷ 6/8 =
3/8 + 1/4 =
1/8 ÷ 4/5 =
8/9 − 5/9 + 2/9 =
2/7 + 1/3 =
4/15 × 3/5 =
6/7 − 3/4 =
DECIMALS
Converting Decimals to Fractions
Decimals represent proportions of a whole, similar to fractions. Each decimal place represents a factor of 10. So the first decimal place represents a number over 10, the second decimal place represents a number over 100, the third decimal place represents a number over 1000, the fourth decimal place represents a number over 10,000, and so on.
To convert a decimal to a fraction, take the number as the numerator and place it over 10, 100, 1000, and so on based on how many numbers are to the right of the decimal point. For example:
0.6 = 6/10 0.58 = 58/100
0.926 = 926/1000 0.7841 = 7841/10,000
Adding and Subtracting Decimals
When adding or subtracting decimal points, it is necessary to keep the decimal points in a vertical line. Then add or subtract each vertical row as you normally would.
| 3.83 | 4.4992 |
| + 1.358 | − 1.738 |
| ________ | ________ |
| 5.188 | 2.7612 |
Multiplying Decimals
Multiplying decimals requires two basic steps. First, multiply the two decimals just as you would any numbers, paying no concern to where the decimal point is located. Once you have completed that operation, add the number of places to the right of the decimal in each number and count off that many decimal points in the solution line. That is your answer, which you may round up to three decimal places (two for the final answer).

Dividing Decimals
When dividing decimals, it is easiest to multiply each decimal by the factor of 10 associated with the number of places to the right of the decimal point. So, if one of the numbers has two numbers to the right of the decimal point and the other number has one, each number should be multiplied by 100. For example:
0.7 ÷ 1.32 = 0.7/1.32
Then multiply each side by the factor of 10 associated with the most spaces to the right of the decimal point in either number. In this case, that is 2, so we multiply each side by 100.
0.7 × 100 = 70
1.32 × 100 = 132
The new fraction is 70/132, which we can solve: 70 ÷ 132 = 0.530
SELF-QUIZ #4: Decimals
(Answers to this quiz can be found on page A-
1.83 × 0.68 =
2.637 + 4.2 =
1.894 − 0.62 =
0.35 ÷ 0.7 =
3.419 × 0.12 =
0.82/1.74 =
0.125 ÷ 0.625 =
0.44 × 0.163 =
0.8 + 1.239 =
13.288 − 4.46 =
PERCENTAGES
Converting Percentages to Fractions or Decimals
Convert a percentage into a fraction by removing the percentage symbol and placing the number over a denominator of 100.
82% = 82/100 or 41/50 or 0.82
20% = 20/100 or 1/5 or 0.2
Multiplying with Percentages
In statistics, it is often necessary to determine the percentage of a whole number when analyzing data. To multiply with a percentage, convert the percentage to a decimal (see Table A.3) and solve the equation. To convert a percentage to a decimal, remove the percentage symbol and move the decimal point two places to the left.
80% of 45 = 80% × 45 = 0.80 × 45 = 36
25% of 94 = 25% × 94 = 0.25 × 94 = 23.5
SELF-QUIZ #5: Percentages
(Answers to this quiz can be found on page A-
45% × 100 =
22% of 80 =
35% of 90 =
80% × 23 =
58% × 60 =
32 × 16% =
125 × 73% =
24 × 75% =
69% of 224 =
51% × 37 =