9.1 CONDUCTING A SINGLE-SAMPLE t TEST

In How It Works 7.2, we conducted a z test for data from the Consideration of Future Consequences (CFC) scale (Adams, 2012). How can we conduct all six steps of hypothesis testing for a single-sample t test for the same data using a p level of 0.05 and a two-tailed test? To start, we use the population mean CFC score of 3.20, but pretend that we no longer know the population standard deviation. As before, we wonder whether behavioral sciences students who joined a career discussion group might have improved CFC scores, on average, compared with the population. Forty-five students attended these discussion groups, and had a mean CFC score of 3.45 with a standard deviation of 0.52.

Step 1: Population 1: All students in career discussion groups. Population 2: All students who did not participate in career discussion groups.

The comparison distribution will be a distribution of means. The hypothesis test will be a single-sample t test because we have only one sample and we know the population mean, but we do not know the population standard deviation. This study meets two of the three assumptions and may meet the third. The dependent variable is scale. In addition, there are more than 30 participants in the sample, indicating that the comparison distribution will be normal. The data were not randomly selected, however, so we must be cautious when generalizing.

Step 2: Null hypothesis: Students who participated in career discussion groups had the same CFC scores, on average, as students who did not participate—H₀: µ₁₌ µ₂. Research hypothesis: Students who participated in career discussion groups had different CFC scores, on average, than students who did not participate—H₁: µ₁ ≠ µ₂.

Step 3: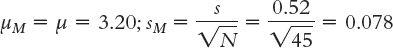

Step 4: df = N − 1 = 45 − 1 = 44

The critical values, based on 44 degrees of freedom (because 44 is not in the table, we look up the more conservative degrees of freedom of 40), a p level of 0.05, and a two-tailed test, are −2.021 and 2.021.

Step 5: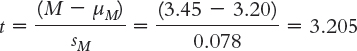

Step 6: Reject the null hypothesis. It appears that students who participate in career discussion groups have higher CFC scores, on average, than do students who do not participate.

The statistics, as presented in a journal article, would read:

t(44) = 3.21, p < 0.05

(Note: If we had used software, we would report the actual p value instead of just whether the p value is larger or smaller than the critical p value.)

9.2 CONDUCTING A PAIRED-SAMPLES t TEST

Salary Wizard is an online tool that allows you to look up incomes for specific jobs for cities in the United States. We looked up the 25th percentile for income for six jobs in two cities: Boise, Idaho, and Los Angeles, California. The data are below.

238

How can we conduct a paired-samples t test to determine whether income in one of these cities differs, on average, from income in the other? We’ll use a two-tailed test and a p level of 0.05.

Step 1: Population 1: Job types in Boise, Idaho. Population 2: Job types in Los Angeles, California.

The comparison distribution will be a distribution of mean differences. The hypothesis test will be a paired-samples t test because we have two samples, and all participants—the job types, in this case—are in both samples.

This study meets the first of the three assumptions and may meet the third. The dependent variable, income, is scale. We do not know whether the population is normally distributed, there are not at least 30 participants, and there is not much variability in the data in the samples, so we should proceed with caution. The data were not randomly selected, so we should be cautious when generalizing beyond this sample of job types.

Step 2: Null hypothesis: Jobs in Boise pay the same, on average, as jobs in Los Angeles—H₀: µ₁ = µ₂. Research hypothesis: Jobs in Boise pay different incomes, on average, than do jobs in Los Angeles—H₁: µ₁ ≠ µ₂.

Step 3: µ_M = µ = 0; s_M = 438.919

M_difference = 7914.333

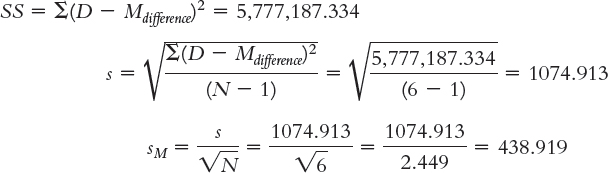
Step 4: df = N − 1 = 6 − 1 = 5

The critical values, based on 5 degrees of freedom, a p level of 0.05, and a two-tailed test, are −2.571 and 2.571.

Step 5: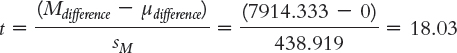

Step 6: Reject the null hypothesis. It appears that jobs in Los Angeles pay more, on average, than do jobs in Boise.

The statistics, as they would be presented in a journal article, are:

t(5) = 18.03, p < 0.05