Chapter 1. Impact 15.1
Impact…ON BIOCHEMISTRY: I15.1 The helix–coil transition in polypeptides
The hydrogen bonds between amino acids of a polypeptide give rise to stable helical or sheet structures, which may collapse into a random coil when certain conditions are changed. The unwinding of a helix into a random coil is a cooperative transition, in which the polymer becomes increasingly more susceptible to structural changes once the process has begun. We examine here a model based on the principles of statistical thermodynamics that accounts for the cooperativity of the helix–coil transition in polypeptides.
To calculate the fraction of polypeptide molecules present as helix or coil we need to set up the partition function for the various states of the molecule. To illustrate the approach, consider a short polypeptide with four amino acid residues, each labelled h if it contributes to a helical region and c if it contributes to a random coil region. We suppose that conformations hhhh and cccc contribute terms q0and q4, respectively,to the partition function q. Then we assume that each of the four conformations with one c amino acid (such as hchh) contributes q1. Similarly, each of the six states with two c amino acids contributes a term q2, and each of the four states with three c amino acids contributes a term q3. The partition function is then
\(q = q_{0} + 4q_{1} + 6q_{2} + 4q_{3} + q_{4} = q_{0} (1 + \frac{4q_{1}}{q_{0}} + \frac{6q_{2}}{q_{0}} + \frac{4q_{3}}{q_{0}} + \frac{q_{4}}{q_{0}})\)
We shall now suppose that each partition function differs from q0 only by the energy of each conformation relative to hhhh, and write
\( \frac{q_{1}}{q_{0}} = \mathtt{e}^ {-( \varepsilon_{i}-\varepsilon_{0})/kT}\)
Next, we suppose that the conformational transformations are non-cooperative, in the sense that the energy associated with changing one h amino acid into one c amino acid has the same value regardless of how many h or c amino acid residues are in the reactant or product state and regardless of where in the chain the conversion occurs. That is, we suppose that the difference in energy between cih4–i and ci+1h3–i has the same value \(\gamma\) for all i. This assumption implies that \( \varepsilon_{i} - \varepsilon_{0} = i \gamma \) and therefore that
\( \frac{q}{q_{0}} = 1 + 4s + 6s^{2} + 4s^{3} + s^{4} = (1 + s)^{4}\)
\( s = \mathtt{e}^{- \gamma /kT}\)
where s is called the stability parameter. The extension of this treatment to take into account a longer chain of residues is now straightforward: we simply replace the 4 in the sum by N:
\(\frac{q}{q_{0}} = (1 + s)^{N}\)
A cooperative transformation is more difficult to accommodate, and depends on building a model of how neighbours facilitate each other’s conformational change. In the simple zipper model, conversion from h to c is allowed only if a residue adjacent to the one undergoing the conversion is already a c residue. Thus, the zipper model allows a transition of the type ...hhhch... \(\rightarrow\) ...hhhcc..., but not a transition of the type ...hhhch... \(\rightarrow\) ...hchch.... The only exception to this rule is, of course, the very first conversion from h to c in a fully helical chain. Cooperativity is included in the zipper model by assuming that the first conversion from h to c, called the nucleation step, is less favourable than the remaining conversions and replacing s for that step by \(\sigma s\), where \(\sigma\) << 1. Each subsequent step is called a propagation step and has a stability parameter s.
A more sophisticated model for the helix–coil transition must allow for helical segments to form in different regions of a long polypeptide chain, with the nascent helices being separated by shrinking coil segments. Calculations based on this more complete Zimm–Bragg model give
\(\theta = \frac {1}{2} \{1 + \frac{(s - 1) + 2\sigma}{[(s - 1)^{2} + 4s\sigma ]^{1/2}}\}\)
where \(\theta\) = (mean number of coil units)/(total units) is the degree of conversion of a polypeptide to a random coil. Figure I15.1 shows plots of \(\theta\) against s for several values of \(\sigma\). The curves show the sigmoidal shape characteristic of cooperative behaviour. There is a sudden surge of transition to a random coil as s passes through 1, and the smaller the parameter \(\sigma\), the greater the sharpness and hence the greater the cooperativity of the transition. That is, the harder it is to get coil formation started, the sharper the transition from helix to coil.
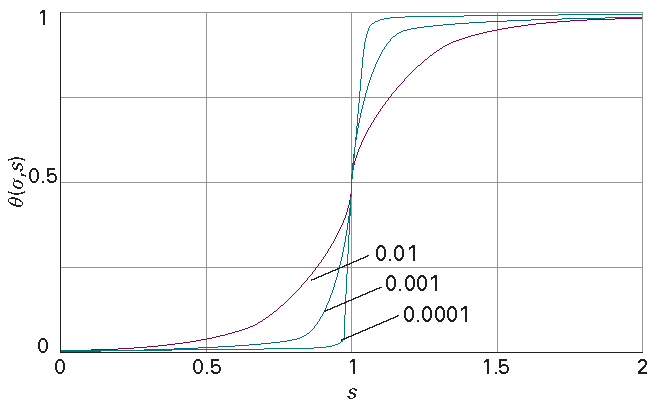
Figure I15.1 Plots of the degree of conversion θ, against s for several values of σ. The curves show the sigmoidal shape characteristics of cooperative behaviour.