8.10: A trait does not decrease in frequency simply because it is recessive.

Do recessive traits gradually become less common in a population? In the early 1900s, there was much discussion among biologists about this question. They wondered: “If the allele for brown eyes is dominant over the allele for blue eyes, why doesn’t a population eventually become all brown-eyed?”

R. C. Punnett (originator of the Punnett square, described in Chapter 7) posed this question to G. H. Hardy, a mathematician with whom Punnett played cricket. Hardy replied the next day, and he published his answer in Science in 1908. It turns out that Wilhelm Weinberg answered the question independently six months earlier. But because he published his findings in an obscure journal, scientists didn’t appreciate his result for another 35 years.

Their result underlies all evolutionary genetics and is referred to as the Hardy-Weinberg Law. It reveals several things, including that the answer to the question of whether recessive traits become rarer in a population is no. A trait does not decrease in frequency simply because it is recessive. Here’s how Hardy and Weinberg demonstrated it.

First, we refer to the frequency of the dominant allele in the population, A, as “p” and the frequency of the recessive allele, a, as “q.” Since every allele in the population has to be either A or a, we can say that p + q = 1. The percentage of the two alleles must total 100%. If we know the frequency of either allele in the population, we can subtract it from 1 to calculate the frequency of the other allele.

Next, we can predict how common each genotype in the population will be. The frequency of AA is just the probability that an individual gets two copies of allele A, which is p × p, or p². Applying the same math, the frequency of aa individuals in the population is simply q × q, or q². Predicting the frequency of heterozygous individuals, Aa, is slightly more complicated. Because the dominant allele may come from either the mother or the father, the frequency of the Aa genotype is 2 × p × q, or 2pq. In other words, it’s actually the frequency of the Aa genotype (i.e., getting the dominant allele from the mother) and the aA genotype (i.e., getting the dominant allele from the father), which is p × q plus q × p, which simplified to 2pq.

Consider an example (FIGURE 8-21). Suppose a population of 1,000 kangaroo rats has the following phenotype and genotype frequencies: 16 are dark brown (BB), 222 are spotted (Bb), and 762 are light brown (bb). The trait shows incomplete dominance, with the allele for dark brown color, B, dominant over the allele for light brown color, b, and the heterozygote having a spotted phenotype. Because every individual in the population has two alleles for the coat-color gene (one from each parent), there are twice as many alleles as members of the population. So a population of 1,000 kangaroo rats has 2,000 alleles of the gene for coat color.

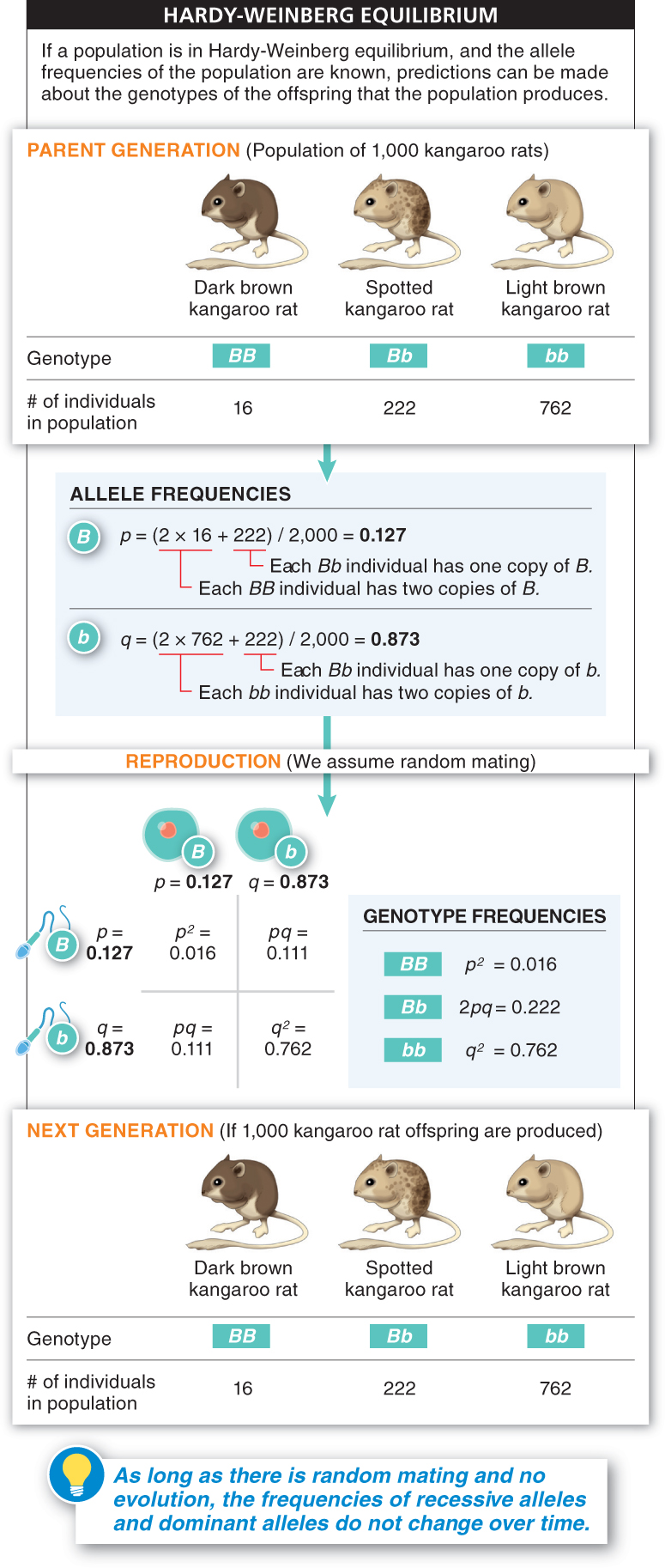
Now let’s look at the allele frequencies. Each BB individual has two copies of B, and each Bb individual has one copy:

Frequency of B = [(2 × 16) + 222]/2,000 = 0.127 = p

Frequency of b = [(2 × 762) + 222]/2,000 = 0.873 = q

Since we know the allele frequencies, p (0.127) and q (0.873), we can determine the genotype frequencies of the offspring produced in this population. According to the equations above, they should be:

Frequency of BB = p² = (0.127)² = 0.016

Frequency of Bb = 2pq = 2(0.127)(0.873) = 0.222

Frequency of bb = q² = (0.873)² = 0.762.

If 1,000 kangaroo rats are produced, we expect to see genotype frequencies that are the same as in the parent generation: 16 BB, 222 Bb, and 762 bb. And from these genotype frequencies, we expect the following allele frequencies among the offspring they produce:

Frequency of B = (2 × 16 + 222)/2,000 = 0.127 = p

Frequency of b = (2 × 762 + 222)/2,000 = 0.873 = q

Notice that the frequencies are unchanged. The allele frequencies 0.127 and 0.873 will always produce the same genotype frequencies—0.16, 0.222, and 0.762—which, in turn, will always have the same allele frequencies. Put another way: the recessive allele doesn’t decrease over time! It doesn’t change at all.

This is true as long as individuals are not dying off specifically because they carry the recessive allele (or because they carry the dominant), in which case the allele frequencies would be changing due to natural selection. It also holds true as long as any of the other mechanisms of evolution that we discussed earlier are not acting on the population—that is, only if mutations, migration, or genetic drift aren’t altering the allele frequencies. In each of these exceptions, the allele frequencies would be changing through evolution. The Hardy-Weinberg conclusion also assumes random mating, in which the alleles are randomly coming together in all possible genotypes.

As long as these assumptions hold true—that is, random mating and no evolution—allele frequencies will not change over time, and the Hardy-Weinberg equations allow us to predict the genotype frequencies we should see.

What if we examine a population and find that the genotype frequencies we observe are not those predicted by the Hardy-Weinberg equations? There may be, for example, fewer heterozygotes than predicted. If this occurs, we say that the population is not in Hardy-Weinberg equilibrium, and we know that either evolution or non-random mating is occurring, or both. If there are fewer heterozygotes than we expected, for example, it may be that more heterozygotes than homozygotes are dying, for some reason—perhaps predation. Or maybe individuals are preferentially mating with individuals having a similar phenotype. In either case, our calculations help us better understand the forces influencing the population and suggest further lines of investigation.