How do the conditions of the Hardy–Weinberg law lead to genotypic proportions of p², 2pq, and q²? Mendel’s principle of segregation says that each individual organism possesses two alleles at a locus and that each of those two alleles has an equal probability of passing into a gamete. Thus, the frequencies of alleles in gametes will be the same as the frequencies of alleles in the parents. Suppose that we have a Mendelian population in which the frequencies of alleles A and a are p and q, respectively. These frequencies will also be those in the gametes. If mating is random (one of the assumptions of the Hardy–Weinberg law), the gametes will come together in random combinations, which can be represented by a Punnett square (­Figure 18.2).

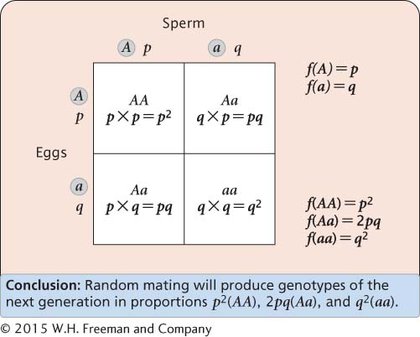
The multiplication rule (see Chapter 3) can be used to determine the probability of various gametes pairing. For example, the probability of a sperm containing allele A is p and the probability of an egg containing allele A is p. Applying the multiplication rule, we find that the probability that these two gametes will combine to produce an AA homozygote is p × p = p². Similarly, the probability of a sperm containing allele a combining with an egg containing allele a to produce an aa homozygote is q × q = q². An Aa heterozygote can be produced in one of two ways: (1) a sperm containing allele A may combine with an egg containing allele a (p × q) or (2) an egg containing allele A may combine with a sperm containing allele a (p × q). Thus, the probability of alleles A and a combining to produce an Aa heterozygote is 2pq. In summary, whenever the frequencies of alleles in a randomly mating population are p and q, the frequencies of the genotypes in the next generation will be p², 2pq, and q². Figure 18.2 demonstrates that only a single generation of random mating is required to produce the Hardy–Weinberg genotypic proportions.

474