EXAMPLE 4.22 How Many Go to MLB?

For baseball, 6.8% of high school players go on to play at the college level. Of these, 9.4% will play in Major League Baseball (MLB).18 Borrowing the notation of Example 4.21, the probability of a high school player ultimately playing professionally is . To find , consider the tree diagram shown in Figure 4.8.

Each segment in the tree is one stage of the problem. Each complete branch shows a path that a player can take. The probability written on each segment is the conditional probability that a player follows that segment given that he has reached the point from which it branches. Starting at the left, high school baseball players either do or do not compete in college. We know that the probability of competing in college is , so the probability of not competing is . These probabilities mark the leftmost branches in the tree.

Conditional on competing in college, the probability of playing in MLB is . So the conditional probability of not playing in MLB is

These conditional probabilities mark the paths branching out from in Figure 4.8.

The lower half of the tree diagram describes players who do not compete in college . For baseball, in years past, the majority of destined professional players did not take the route through college. However, nowadays it is relatively unusual for players to go straight from high school to MLB. Studies have shown that the conditional probability that a high school athlete reaches MLB, given that he does not compete in college, is .19 We can now mark the two paths branching from in Figure 4.8.

202

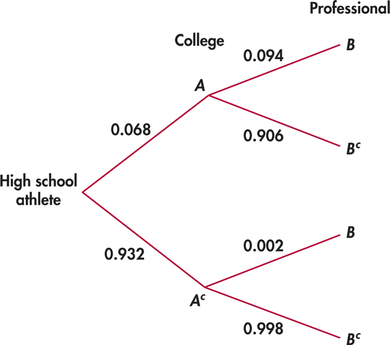
image
Figure 4.8: FIGURE 4.8 Tree diagram and probabilities, Example 4.22.

There are two disjoint paths to (MLB play). By the addition rule, is the sum of their probabilities. The probability of reaching through college (top half of the tree) is

The probability of reaching without college is

The final result is

About eight high school baseball players out of 1000 will play professionally. Even though this probability is quite small, it is comparatively much greater than the chances of making it to the professional ranks in basketball and football.