Example 6.6

EXAMPLE 6.6 The Central Limit Theorem in Action

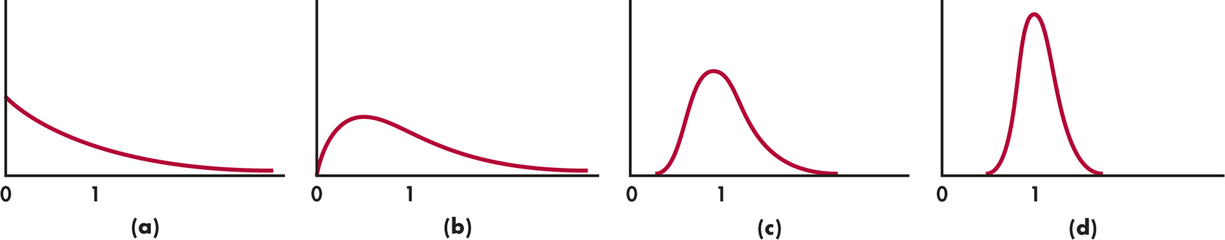
Figure 6.3 shows the central limit theorem in action for another very non-Normal population. Figure 6.3(a) displays the density curve of a single observation from the population. The distribution is strongly right-skewed, and the most probable outcomes are near 0. The mean of this distribution is 1, and its standard deviation is also 1. This particular continuous distribution is called an exponential distribution. Exponential distributions are used as models for how long an iOS device, for example, will last and for the time between text messages sent on your cell phone.

exponential distribution

Figure 6.3: FIGURE 6.3 The central limit theorem in action: the distribution of sample means from a strongly non-Normal population becomes more Normal as the sample size increases. (a) The distribution of one observation. (b) The distribution of for two observations. (c) The distribution of for 10 observations. (d) The distribution of for 25 observations.

296

Figures 6.3(b), (c), and (d) are the density curves of the sample means of 2, 10, and 25 observations from this population. As increases, the shape becomes more Normal. The mean remains at , but the standard deviation decreases, taking the value The density curve for 10 observations is still somewhat skewed to the right but already resembles a Normal curve having and . The density curve for is yet more Normal. The contrast between the shape of the population distribution and of the distribution of the mean of 10 or 25 observations is striking.