Chapter 1. Impact 13.1

Impact …ON ENGINEERING: I13.1 Refrigeration

The argument used to discuss the efficiency of a heat engine can be used to discuss the efficiency of a refrigerator, a device for transferring energy as heat from a cold object (the contents of the refrigerator) to a warm sink (typically, the room in which the refrigerator stands). The less work we have to do to bring this transfer about, the more efficient is the refrigerator.

When an energy |q_c| migrates from a cool source at a temperature T_c into a warmer sink at a temperature T_h, the change in entropy is

\(\Delta S=- \frac{| q_{c}|}{T_{c}}+\frac{| q_{c}|}{T_{h}} < 0\)

The process is not spontaneous because not enough entropy is generated in the warm sink to overcome the entropy loss from the cold source (Fig. 1). To generate more entropy, energy must be added to the stream that enters the warm sink. Our task is to find the minimum energy that needs to be supplied. The outcome is expressed as the coefficient of performance, c:

\(c= \frac{\mathrm {energy}\;\mathrm {transferred}\;\mathrm {as}\;\mathrm {heat}}{\mathrm {energy}\;\mathrm {supplied}\;\mathrm {as}\;\mathrm {work}} = \frac{ |q_{c}| }{|w|} \)

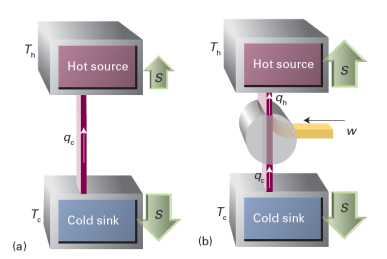
Fig. 1 (a) The flow of energy as heat from a cold source to a hot sink is not spontaneous. As shown here, the entropy increase of the hot sink is smaller than the entropy increase of the cold source, so there is a net decrease in entropy. (b) The process becomes feasible if work is provided to add to the energy stream. Then the increase in entropy of the hot sink can be made to cancel the entropy decrease of the hot source.

The less the work that is required to achieve a given transfer, the greater the coefficient of performance and the more efficient is the refrigerator. For some of this development it will prove best to work with 1/c.

Because |q_c| is removed from the cold source, and the work |w| is added to the energy stream, the energy deposited as heat in the hot sink is |q_h| = |q_c| + |w|. Therefore,

\( \frac{1}{c}= \frac{|w|}{ |q_{c}|}=\frac{|q_{h}|- |q_{c}| }{ |q_{c}|}=\frac{| q_{h} |}{ |q_{c}|}-1\)

We can now use eqn 61.4 (q_h/q_c = –T_h/T_c) to express this result in terms of the temperatures alone, which is possible if the transfer is performed reversibly. This substitution leads to

\( \frac{1}{c}= \frac{ T_{h} }{T_{c}}-1= \frac{ T_{h}- T_{c}}{ T_{c}}\)

and therefore

\(c= \frac{ T_{c} }{ T_{h} - T_{c} }\)

for the thermodynamically optimum coefficient of performance. For instance, for a refrigerator withdrawing heat from ice-cold water (T_c = 273 K) in a typical environment (T_h = 293 K), c = 14, so, to remove 10 kJ (enough to freeze 30 g of water), requires transfer of at least 0.71 kJ as work. Practical refrigerators, of course, have a lower coefficient of performance.