UP CLOSE CALCULATING THE HARDY-WEINBERG EQUILIBRIUM

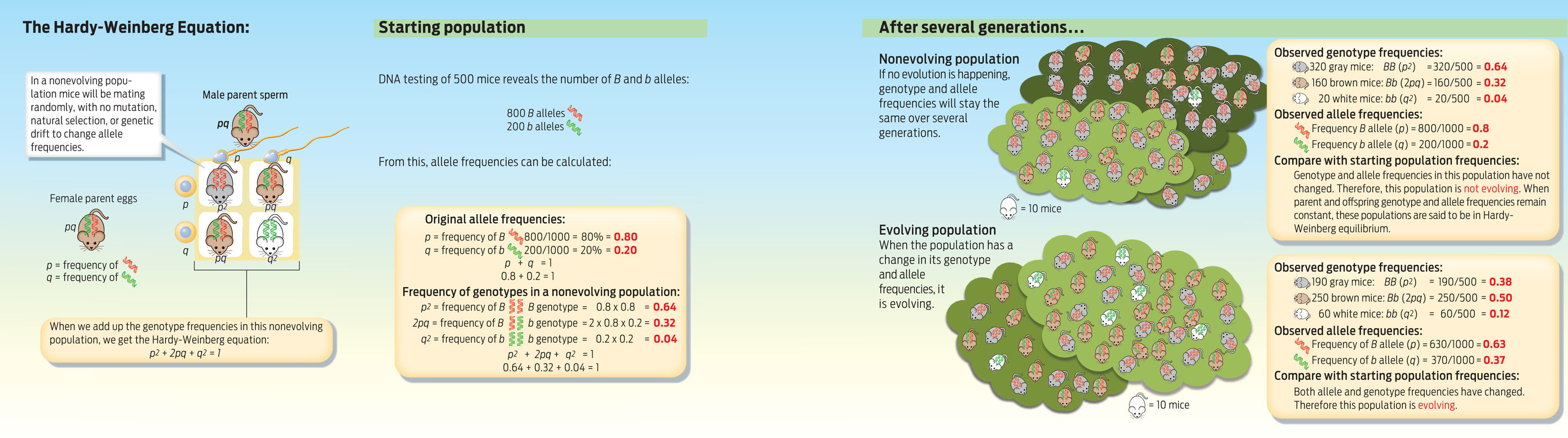
How do we know if a population is evolving? To find out, we use a mathematical formula called the Hardy-Weinberg equation, which calculates the frequency of genotypes you would expect to find in a nonevolving population from simple rules of probability. For a gene with two alleles, B and b, with allele frequencies p and q, this formula can be written as:

By definition, a population is not evolving (and is therefore in Hardy-Weinberg equilibrium) when it has stable allele frequencies and stable genotype frequencies from generation to generation. This can be achieved only when all five of the following conditions are met:

1. No mutation introducing new alleles into the population
2. No natural selection favoring some alleles over others
3. An infinitely large population size (and therefore no genetic drift)
4. No gene flow between populations
5. Random mating of individuals

In nature, no population can ever be in strict Hardy-Weinberg equilibrium, since it will never meet all five conditions. For example, because no real population is infinitely large, genetic drift will always occur. In other words, all natural populations are evolving. Nevertheless, by describing the pattern of genotypes in a nonevolving population, Hardy-Weinberg equilibrium provides a baseline from which to measure evolution.

To see how the Hardy-Weinberg equation can be used to detect evolutionary change, consider the following example. Say you have a population of mice with two alleles (B and b) and three possible phenotypes for fur color, gray (BB), brown (Bb), and white (bb). As every individual in the population has two alleles for the fur-color gene (one maternal and one paternal), there are twice as many alleles as there are members of the population. So a population of 500 mice has 1,000 alleles of the gene for fur color.

Now let’s say we sample the DNA of our mice population and determine that there are 800 B alleles in the population and 200 b alleles. We would then say that the frequency of the B allele is 0.8 (800/1,000) and the frequency of the b allele is 0.2 (200/1,000). Since there are only two alleles in the population, their combined frequencies will add up to 1. If we use p to denote the frequency of B and q to denote the frequency of b, then we can say that p + q = 1.

Suppose we want to use those allele frequencies to calculate the expected frequency of white-furred (bb) individuals in the population if the population is indeed in Hardy-Weinberg equilibrium. If the frequency of b in the population is q, then we know from the Hardy-Weinberg equation that the expected frequency of bb is q² = (.2)(.2) = .04, and that this frequency will remain constant over generations. Thus, in our population of mice, 4%, or 20 mice, would be expected to have white fur, if the population is in Hardy-Weinberg equilibrium. If we find out that the actual percentage of white mice in the population is more or less than this number, then we know that our population is evolving, and we can begin to investigate why.

The Hardy-Weinberg equation also has important applications in public health. It can be used, for example, to estimate the frequency of carriers (heterozygotes) of rare recessive diseases in a population (see Question 17 in Test Your Knowledge for an example).