The 68–95–99.7 rule
There are many Normal curves, each described by its mean and standard deviation. All Normal curves share many properties. In particular, the standard deviation is the natural unit of measurement for Normal distributions. This fact is reflected in the following rule.
The 68–95–99.7 rule
In any Normal distribution, approximately
• 68% of the observations fall within one standard deviation of the mean.
• 95% of the observations fall within two standard deviations of the mean.
• 99.7% of the observations fall within three standard deviations of the mean.
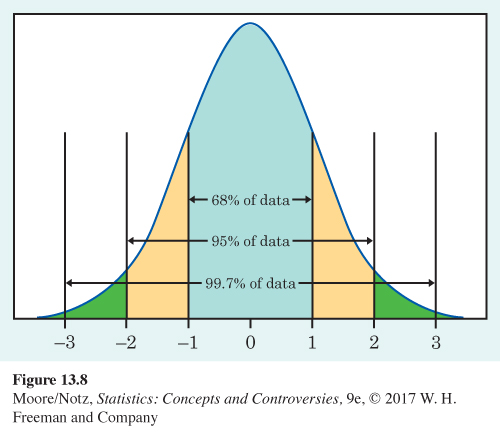
Figure 13.8 illustrates the 68–95–99.7 rule. By remembering these three numbers, you can think about Normal distributions without constantly making detailed calculations. Remember also, though, that no set of data is exactly described by a Normal curve. The 68–95–99.7 rule will be only approximately true for SAT scores or the lengths of crickets because these distributions are approximately Normal.

EXAMPLE 2 Heights of young women
The distribution of heights of women aged 18 to 24 is approximately Normal with mean 65 inches and standard deviation 2.5 inches. To use the 68–95–99.7 rule, always start by drawing a picture of the Normal curve. Figure 13.9 shows what the rule says about women’s heights.
Half of the observations in any Normal distribution lie above the mean, so half of all young women are taller than 65 inches.
The central 68% of any Normal distribution lies within one standard deviation of the mean. Half of this central 68%, or 34%, lies above the mean. So 34% of young women are between 65 inches and 67.5 inches tall. Adding the 50% who are shorter than 65 inches, we see that 84% of young women have heights less than 67.5 inches. That leaves 16% who are taller than 67.5 inches.
The central 95% of any Normal distribution lies within two standard deviations of the mean. Two standard deviations is 5 inches here, so the middle 95% of young women’s heights are between 60 inches (that’s 65 − 5) and 70 inches (that’s 65 + 5).
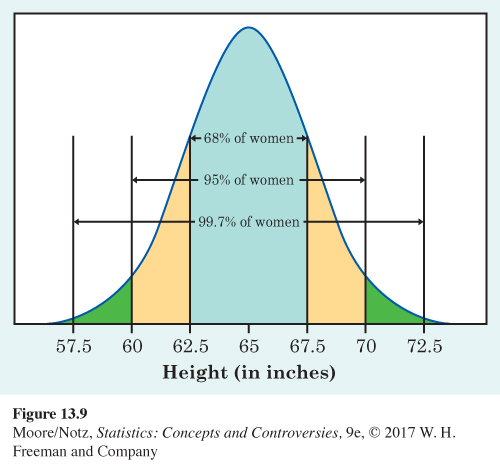
The other 5% of young women have heights outside the range from 60 to 70 inches. Because the Normal distributions are symmetric, half of these women are on the short side. The shortest 2.5% of young women are less than 60 inches (5 feet) tall.
Almost all (99.7%) of the observations in any Normal distribution lie within three standard deviations of the mean. Almost all young women are between 57.5 and 72.5 inches tall.
NOW IT’S YOUR TURN
Question 13.1
13.1 Heights of young men. The distribution of heights of young men is approximately Normal with mean 70 inches and standard deviation 2.5 inches. Between which heights do the middle 95% of men fall?
13.1 The central 95% of any Normal distribution lies within two standard deviations of the mean. Two standard deviations is 5 inches here, so the middle 95% of the young men’s heights is between 65 inches (that’s 70 − 5) and 75 inches (that’s 70 + 5).