CHAPTER 12 EXERCISES
Question 12.9
12.9 Median income. You read that the median income of U.S. households in 2013 was $51,939. Explain in plain language what “the median income’’ is.
12.9 Half of all households make more than the median ($51,939), and the other half make less.
Question 12.10
12.10 What’s the average? The Census Bureau website gives several choices for ‘‘average income’’ in its historical income data. In 2013, the median income of American households was $51,939. The mean household income was $72,641. The median income of families was $63,815, and the mean family income was $84,687. The Census Bureau says, “Households consist of all people who occupy a housing unit. The term ‘family’ refers to a group of two or more people related by birth, marriage, or adoption who reside together.’’ Explain carefully why mean incomes are higher than median incomes and why family incomes are higher than household incomes.
Question 12.11
12.11 Rich magazine readers. Echo Media reports that the average income for readers of the magazine WatchTime (a magazine for people interested in fine watches) is $298,400. Is the median wealth of these readers greater or less than $298,400? Why?
12.11 The median wealth of these readers should be less than $298,400 (the mean) because income distributions tend to be right skewed.
ex12-12
Question 12.12
12.12 College tuition. Figure 11.7 (page 255) is a stemplot of the tuition charged by 116 colleges in Illinois. The stems are thousands of dollars and the leaves are hundreds of dollars. For example, the highest tuition is $38,600 and appears as leaf 6 on stem 38.
(a) Find the five-number summary of Illinois college tuitions. You see that the stemplot already arranges the data in order.
(b) Would the mean tuition be clearly smaller than the median, about the same as the median, or clearly larger than the median? Why?
ex12-13
Question 12.13
12.13 Where are the young more likely to live? Figure 11.11 (page 259) is a stemplot of the percentage of residents aged 18 to 34 in each of the 50 states. The stems are whole percents and the leaves are tenths of a percent.
(a) The shape of the distribution suggests that the mean will be larger than the median. Why?
(b) Find the mean and median of these data and verify that the mean is larger than the median.
12.13 (a) The distribution is somewhat right skewed, which means the mean will be larger than the median. (b) The mean is 23.1%, and the median is 23.0%.
ex12-14
Question 12.14
12.14 Gas mileage. Table 11.2 (page 261) gives the highway gas mileages for some model year 2015 mid-sized cars.
(a) Make a stemplot of these data if you did not do so in Exercise 11.13.
(b) Find the five-number summary of gas mileages. Which cars are in the bottom quarter of gas mileages?
(c) The stemplot shows a fact about the overall shape of the distribution that the five-number summary cannot describe. What is it?
ex12-15
Question 12.15
12.15 Yankee money. Table 11.4 (page 262) gives the salaries of the New York Yankees baseball team. What shape do you expect the distribution to have? Do you expect the mean salary to be close to the median, clearly higher, or clearly lower? Verify your choices by making a graph and calculating the mean and median.
12.15 We expect the distribution to be right skewed, so the mean ($8.96 million) is greater than the median ($5.00 million).
Question 12.16
12.16 The richest 5%. The distribution of individual incomes in the United States is strongly skewed to the right. In 2013, the mean and median incomes of the top 5% of Americans were $196,723 and $322,343. Which of these numbers is the mean and which is the median? Explain your reasoning.
ex12-17
Question 12.17
12.17 How many calories does a hot dog have? Consumer Reports magazine presented the following data on the number of calories in a hot dog for each of 17 brands of meat hot dogs:
| 173 | 191 | 182 | 190 | 172 | 147 |
| 146 | 139 | 175 | 136 | 179 | 153 |
| 107 | 195 | 135 | 140 | 138 |
Make a stemplot [if you did not already do so in Exercise 11.19 (page 263)], and find the five-number summary. The stemplot shows important facts about the distribution that the numerical summary does not tell us. What are these facts?
12.17 The distribution is irregular in shape. The hot dog brands fall into two groups with a gap between them, plus a low outlier. The five-number summary, in units of calories, is Min = 107, Q1 = 138.5, M = 153, Q3 =179, Max = 180.5. Any numerical summary would not reveal the “gaps” in the distribution.
Question 12.18
12.18 Returns on common stocks. Example 5 informs us that financial theory uses the mean and standard deviation to describe the returns on investments. Figure 11.13 (page 260) is a histogram of the returns of all New York Stock Exchange common stocks in one year. Are the mean and standard deviation suitable as a brief description of this distribution? Why?
ex12-19
Question 12.19
12.19 Minority students in engineering. Figure 11.12 (page 259) is a histogram of the number of minority students (black, Hispanic, Native American) who earned doctoral degrees in engineering from each of 152 universities in the years 2000 through 2002. The classes for Figure 11.12 are 1–5, 6–10, and so on.
(a) What is the position of each number in the five-number summary in a list of 152 observations arranged from smallest to largest?
(b) Even without the actual data, you can use your answer to (a) and the histogram to give the five-number summary approximately. Do this. About how many minority engineering PhDs must a university graduate to be in the top quarter?
12.19 (a) The minimum is in position number 1, and the maximum is in position number 152. The median is in position number 76.5 (halfway between the 76th and 77th numbers), the first quartile is in position number 38.5 (halfway between the 38th and 39th numbers), and the third quartile is in position number 114.5 (halfway between the 114th and 115th numbers). (b) The values in the five-number summary fall in the ranges 1 to 5, 1 to 5, 1 to 5, 6 to 10, and 46 to 50. The top quarter includes universities that produce at least six minority engineering doctorates per year. The actual lower bound is somewhere between 6 and 10.
ex12-20
Question 12.20
12.20 The statistics of writing style. Here are data on the percentages of words of 1 to 15 letters used in articles in Popular Science magazine. Exercise 11.16 (page 263) asked you to make a histogram of these data.
| Length: | 1 | 2 | 3 | 4 | 5 |
| Percent: | 3.6 | 14.8 | 18.7 | 16.0 | 12.5 |
| Length: | 6 | 7 | 8 | 9 | 10 |
| Percent: | 8.2 | 8.1 | 5.9 | 4.4 | 3.6 |
| Length: | 11 | 12 | 13 | 14 | 15 |
| Percent: | 2.1 | 0.9 | 0.6 | 0.4 | 0.2 |
Find the five-number summary of the distribution of word lengths from this table.
ex12-21
Question 12.21
12.21 Immigrants in the eastern states. Here are the number of legal immigrants (in thousands) who settled in each state east of the Mississippi River in 2013:
| Alabama | 3.8 | Connecticut | 10.9 | Delaware | 2.3 |
| Florida | 102.9 | Georgia | 24.4 | Illinois | 36.0 |
| Indiana | 7.7 | Kentucky | 5.2 | Maine | 1.2 |
| Maryland | 25.4 | Massachusetts | 29.5 | Michigan | 17.0 |
| Mississippi | 1.7 | New Hampshire | 2.2 | New Jersey | 53.1 |
| New York | 133.6 | North Carolina | 16.8 | Ohio | 13.8 |
| Pennsylvania | 24.7 | Rhode Island | 3.3 | South Carolina | 4.3 |
| Tennessee | 8.4 | Vermont | 0.8 | Virginia | 27.9 |
| West Virginia | 0.8 | Wisconsin | 5.9 |
Make a graph of the distribution. Describe its overall shape and any outliers. Then choose and calculate a suitable numerical summary.
12.21 The shape is skewed to the right. There are several mild outliers (Illinois and New Jersey) and several extreme outliers (Florida and New York). Because the data are skewed to the right with outliers, the five-number summary would be a suitable numerical summary (in thousands)—Min: 0.8, Q1: 3.3, Med: 9.65, Q3: 25.4, Max: 133.6.
Question 12.22
12.22 Immigrants in the eastern states. New York and Florida are high outliers in the distribution of the previous exercise. Find the mean and the median for these data with and without New York and Florida. Which measure changes more when we omit the outliers?
Question 12.23
12.23 State SAT scores. Figure 12.9 is a histogram of the average scores on the SAT Mathematics exam for college-bound senior students in the 50 states and the District of Columbia in 2014. The distinctive overall shape of this distribution implies that a single measure of center such as the mean or the median is of little value in describing the distribution. Explain why this is true.
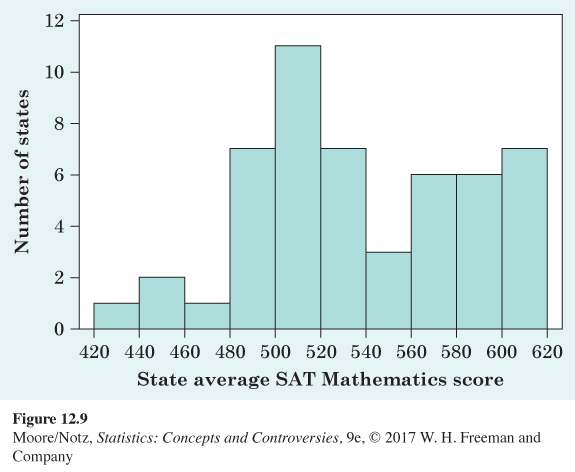
12.23 The histogram appears to be bimodal, suggesting that a single measure of center such as the mean or median is of little value in describing the distribution.
Question 12.24
 12.24 Highly paid athletes. A news article reported that of the 411 players on National Basketball Association rosters in February 1998, only 139 “made more than the league average salary’’ of $2.36 million. Was $2.36 million the mean or median salary for NBA players? How do you know?
12.24 Highly paid athletes. A news article reported that of the 411 players on National Basketball Association rosters in February 1998, only 139 “made more than the league average salary’’ of $2.36 million. Was $2.36 million the mean or median salary for NBA players? How do you know?
Question 12.25
12.25 Mean or median? Which measure of center, the mean or the median, should you use in each of the following situations? Why?
(a) Middletown is considering imposing an income tax on citizens. The city government wants to know the average income of citizens so that it can estimate the total tax base.
(b) In a study of the standard of living of typical families in Middletown, a sociologist estimates the average family income in that city.
12.25 (a) The mean is more useful in this situation because of the need for a total. (b) The median is appropriate here. The word “typical” often refers to median.
Question 12.26
12.26 Mean or median? You are planning a party and want to know how many cans of soda to buy. A genie offers to tell you either the mean number of cans guests will drink or the median number of cans. Which measure of center should you ask for? Why? To make your answer concrete, suppose there will be 30 guests and the genie will tell you either ˉx=5 cans or M=3 cans. Which of these two measures would best help you determine how many cans you should have on hand?
Question 12.27
12.27 State SAT scores. We want to compare the distributions of average SAT Math and Writing scores for the states and the District of Columbia. We enter these data into a computer with the names SATM for Math scores and SATW for Writing scores. At the bottom of the page is output from the statistical software package Minitab. (Other software produces similar output. Some software uses rules for finding the quartiles that differ slightly from ours. So software may not give exactly the answer you would get by hand.)
Use this output to make boxplots of SAT Math and Writing scores for the states. Briefly compare the two distributions in words.
| Minitab output for Exercise 12.27 | ||||||||
| Variable | N | Mean | Median | StDev | Minimum | Maximum | Q1 | Q3 |
| SATM | 51 | 537.82 | 525.00 | 48.26 | 502.00 | 620.00 | 502.00 | 585.00 |
| SATW | 51 | 517.86 | 508.00 | 45.89 | 431.00 | 587.00 | 477.00 | 566.00 |
12.27 SAT Writing scores have a slightly larger interquartile range but much larger range than the SAT Math scores. The center (median) for the SAT Math scores is higher than the SAT Writing scores.
ex12-28
Question 12.28
12.28 Do SUVs waste gas? Table 11.2 (page 261) gives the highway fuel consumption (in miles per gallon) for 31 model year 2015 mid-sized cars. You found the five-number summary for these data in Exercise 12.14. Here are the highway gas mileages for 26 four-wheel-drive model year 2015 sport utility vehicles:
(a) Give a graphical and numerical description of highway fuel consumption for SUVs. What are the main features of the distribution?
(b) Make boxplots to compare the highway fuel consumption of the mid-size cars in Table 11.2 and SUVs. What are the most important differences between the two distributions?
| Model | mpg | Model | mpg |
|---|---|---|---|
| BMW X5 xdrive35i | 27 | Lexus GX460 | 20 |
| Chevrolet Tahoe K1500 | 22 | Lexus LX570 | 17 |
| Chevrolet Traverse | 23 | Lincoln Navigator | 20 |
| Dodge Durango | 24 | Lincoln MKT | 23 |
| Ford Expedition | 20 | Mercedes-Benz ML250 Bluetec 4matic | 29 |
| Ford Explorer | 23 | Mercedes-Benz G63 AMG | 14 |
| GMC Acadia | 23 | Nissan Armada | 18 |
| GMC Yukon | 22 | Nissan Pathfinder Hybrid | 27 |
| Infiniti QX80 | 19 | Porsche Cayenne S | 24 |
| Jeep Grand Cherokee | 20 | Porsche Cayenne Turbo | 21 |
| Land Rover LR4 | 19 | Toyota Highlander | 24 |
| Land Rover Range Rover | 23 | Toyota Land Cruiser Wagon | 18 |
| Land Rover Range Rover Sport | 19 | Toyota 4Runner | 21 |
Question 12.29
12.29 How many calories in a hot dog? Some people worry about how many calories they consume. Consumer Reports magazine, in a story on hot dogs, measured the calories in 20 brands of beef hot dogs, 17 brands of meat hot dogs, and 17 brands of poultry hot dogs. Here is computer output describing the beef hot dogs,
Mean = 156.8 Standard deviation = 22.64 Min = 111 Max = 190 N = 20 Median = 152.5 Quartiles = 140, 178.5
the meat hot dogs,
Mean = 158.7 Standard deviation = 25.24 Min = 107 Max = 195 N = 17 Median = 153 Quartiles = 139, 179
and the poultry hot dogs,
Mean = 122.5 Standard deviation = 25.48 Min = 87 Max = 170 N = 17 Median = 129 Quartiles = 102, 143
(Some software uses rules for finding the quartiles that differ slightly from ours. So software may not give exactly the answer you would get by hand.) Use this information to make boxplots of the calorie counts for the three types of hot dogs. Write a brief comparison of the distributions. Will eating poultry hot dogs usually lower your calorie consumption compared with eating beef or meat hot dogs? Explain.
12.29 The boxplots show that poultry hot dogs as a group are lower in calories than meat and beef hot dogs, which have similar distributions.
Question 12.30
12.30 Finding the standard deviation. The level of various substances in the blood influences our health. Here are measurements of the level of phosphate in the blood of a patient, in milligrams of phosphate per deciliter of blood, made on six consecutive visits to a clinic:
| 5.6 | 5.2 | 4.6 | 4.9 | 5.7 | 6.4 |
A graph of only six observations gives little information, so we proceed to compute the mean and standard deviation.
(a) Find the mean from its definition. That is, find the sum of the six observations and divide by 6.
(b) Find the standard deviation from its definition. That is, find the distance of each observation from the mean, square the distances, then calculate the standard deviation. Example 4 shows the method.
(c) Now enter the data into your calculator and use the mean and standard deviation keys to obtain ˉx and s. Do the results agree with your hand calculations?
Question 12.31
12.31 What s measures. Use a calculator to find the mean and standard deviation of these two sets of numbers:
(a) 4 0 1 4 3 6
(b) 5 3 1 3 4 2
Which data set is more variable?
12.31 Both have mean 3; set (a) has s = 2.19 while set (b) has s =1.41. Set (a) is more variable.
Question 12.32
12.32 What s measures. Add 2 to each of the numbers in data set (a) in the previous exercise. The data are now 6 2 3 6 5 8.
(a) Use a calculator to find the mean and standard deviation and compare your answers with those for data set part (a) in the previous exercise. How does adding 2 to each number change the mean? How does it change the standard deviation?
(b) Without doing the calculation, what would happen to ˉx and s if we added 10 to each value in data set part (a) of the previous exercise? (This exercise demonstrates that the standard deviation measures only variability about the mean and ignores changes in where the data are centered.)
Question 12.33
12.33 Cars and SUVs. Use the mean and standard deviation to compare the gas mileages of mid-size cars (Table 11.2, page 261) and SUVs (Exercise 12.28). Do these numbers catch the main points of your more detailed comparison in Exercise 12.28?
12.33 SUV: ˉx = 21.5 mpg, s = 3.3 mpg; mid-size: ˉx = 37.4 mpg, s = 26.9 mpg; SUVs have a lower average miles per gallon than mid-size vehicles, but mid-size vehicles have more variation in miles per gallon as evidenced by the standard deviation. This agrees will the detailed comparison in Exercise 12.28.
Question 12.34
12.34 A contest. This is a standard deviation contest. You must choose four numbers from the whole numbers 0 to 9, with repeats allowed.
(a) Choose four numbers that have the smallest possible standard deviation.
(b) Choose four numbers that have the largest possible standard deviation.
(c) Is more than one choice correct in either (a) or (b)? Explain.
ex12-35
Question 12.35
12.35 ˉx and s are not enough. The mean ˉx and standard deviation s measure center and variability but are not a complete description of a distribution. Data sets with different shapes can have the same mean and standard deviation. To demonstrate this fact, use your calculator to find ˉx and s for these two small data sets. Then make a stemplot of each and comment on the shape of each distribution.
| Data A: | 9.14 | 8.14 | 8.74 | 8.77 |
| Data B: | 6.58 | 5.76 | 7.71 | 8.84 |
| Data A: | 9.26 | 8.10 | 6.13 | 3.10 |
| Data B: | 8.47 | 7.04 | 5.25 | 5.56 |
| Data A: | 9.13 | 7.26 | 4.74 | 0.00 |
| Data B: | 7.91 | 6.89 | 12.50 | 0.00 |
12.35 Both sets of data have the same mean and standard deviation (ˉx = 7.50 and s = 2.03). However, the two distributions are quite different: set A is left skewed, while set B is roughly uniform with a high outlier.
Question 12.36
12.36 Raising pay. A school system employs teachers at salaries between $40,000 and $70,000. The teachers’ union and the school board are negotiating the form of next year’s increase in the salary schedule. Suppose that every teacher is given a flat $3000 raise.
(a) How much will the mean salary increase? The median salary?
(b) Will a flat $3000 raise increase the variability as measured by the distance between the quartiles? Explain.
(c) Will a flat $3000 raise increase the variability as measured by the standard deviation of the salaries? Explain.
Question 12.37
12.37 Raising pay. Suppose that the teachers in the previous exercise each receive a 5% raise. The amount of the raise will vary from $2000 to $3500, depending on present salary. Will a 5% across-the-board raise increase the variability of the distribution as measured by the distance between the quartiles? Do you think it will increase the standard deviation? Explain your reasoning.
12.37 A 5% across-the-board raise will increase the variability of the distribution. Both the distance between the quartiles and the standard deviation will increase by a factor of 5%. In other words, the new distance between the quartiles and standard deviation after the raise will be 1.05 times the original distance between the quartiles and standard deviation before the raise.
Question 12.38
 12.38 Making colleges look good. Colleges announce an “average’’ SAT score for their entering freshmen. Usually the college would like this “average’’ to be as high as possible. A New York Times article noted, “Private colleges that buy lots of top students with merit scholarships prefer the mean, while open-enrollment public institutions like medians.’’ Use what you know about the behavior of means and medians to explain these preferences.
12.38 Making colleges look good. Colleges announce an “average’’ SAT score for their entering freshmen. Usually the college would like this “average’’ to be as high as possible. A New York Times article noted, “Private colleges that buy lots of top students with merit scholarships prefer the mean, while open-enrollment public institutions like medians.’’ Use what you know about the behavior of means and medians to explain these preferences.
Question 12.39
12.39 What graph to draw? We now understand three kinds of graphs to display distributions of quantitative variables: histograms, stemplots, and boxplots. Give an example (just words, no data) of a situation in which you would prefer that graphing method.
12.39 Answers will vary.

EXPLORING THE WEB
Follow the QR code to access exercises.