10-2 A star’s brightness can be described in terms of luminosity or magnitude

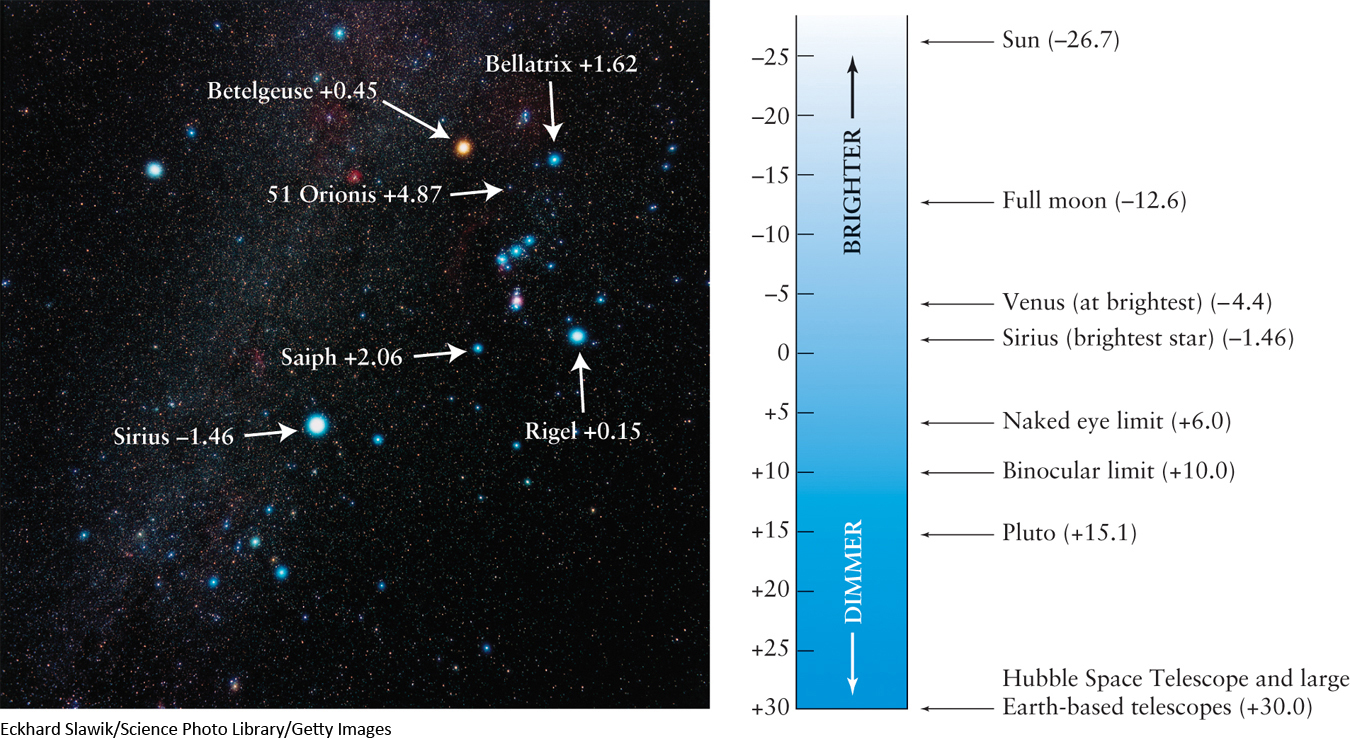
A quick survey of some of the photos in this book suggests that not all stars are the same. Some stars are brilliantly bright, whereas the vast majority is considerably dimmer. Because astronomy is among the most ancient of sciences, some of the words and tools used by modern astronomers to describe the night sky are actually many centuries old. One such tool is the magnitude scale, which astronomers frequently use to denote the brightness of stars. This scale was introduced in the second century b.c.e. by the Greek astronomer Hipparchus, who called the brightest stars first-magnitude stars.

Hipparchus based this scale in part on which stars are the first to be visible in the darkening sky after sunset. In other words, the first group of stars visible as the Sun sets below the horizon are known as magnitude +1 stars. Stars about half as bright as first-magnitude stars, and the second group of stars to become visible as the sky becomes darker, are known as magnitude +2 stars, and so forth, down to sixth-magnitude stars, the dimmest ones that can be seen when the sky is pitch black. After telescopes came into use, astronomers extended Hipparchus’s magnitude scale to include even dimmer stars.

This numbering scale that ranks stars, where the brightest objects are “number one,” is quite common in our culture. The winner of baseball’s World Series is the number one team, the restaurant voted best in your community is the number one restaurant, and the best Olympic performer in gymnastics is the number one gymnast. So, it seems reasonable that when ranking stars by brightness, the brightest stars are magnitude one, the not-as-bright-as-number-one stars are magnitude two, and so on.

In the nineteenth century, astronomers developed better techniques for measuring the light energy arriving on Earth from a star. These measurements showed that a first-magnitude star is about 100 times brighter than a sixth-magnitude star. In other words, it would take 100 stars of magnitude +6 to provide as much light energy as we receive from a single star of magnitude +1. To make computations easier, the magnitude scale was redefined so that a magnitude difference of 5 corresponds exactly to a factor of 100 in brightness. A magnitude difference of 1 corresponds to a factor of 2.512 in brightness, because

Thus, it takes 2.512 third-magnitude stars to provide as much light as we receive from a single second-magnitude star.

One might imagine that the objects might have a different magnitude rating if the observer were significantly closer to it or, alternatively, farther away from it. Because Hipparchus’s magnitude numbers describe how bright an object appears to an Earth-based observer, values in Hipparchus’s scale are properly called apparent magnitudes.

Figure 10-5 illustrates the modern apparent magnitude scale. The dimmest stars visible through a pair of binoculars have an apparent magnitude of +10, and the dimmest stars that can be detected with the Hubble Space Telescope have an apparent magnitude greater than +30.

In contrast to looking for the dimmest objects, we also need to be able to describe objects brighter than typical stars, such as the full moon. Modern astronomers use negative numbers to extend Hipparchus’s scale for the brightest of objects. For example, Sirius, the brightest star in the night sky, has a carefully measured apparent magnitude of −1.46. The Sun, the brightest object in the sky, has an apparent magnitude of −26.7.

Using this apparent magnitude scale can be misleading when judging the nature of stars. For example, our star the Sun has about the same energy output as the star Alpha Centauri. However, our Sun is much closer to Earth than Alpha Centauri so it appears many, many times brighter in the sky. In other words, because in fact some stars are relatively close to Earth and some stars are relatively far from Earth, judging all stars only by their apparent magnitudes provides an incomplete picture of the cosmos.

Go to Video 10-2

To solve this problem, astronomers imagine what the brightness or magnitude of a star would be IF the star were located exactly 10 pc (32.6 ly) from Earth. The magnitude of a star if it were at a distance of 10 pc is known as the absolute magnitude, and it is a quantity that reflects a star’s true energy output so it can be compared to other stars.

If the Sun were moved to a distance of 10 pc from Earth, it would have an apparent magnitude of +4.8. The absolute magnitude of the Sun is thus +4.8. The absolute magnitudes of the stars range from approximately +15 for the least luminous to −10 for the most luminous. (Note: Like apparent magnitudes, absolute magnitudes work “backward”: The greater the absolute magnitude, the less luminous the star.) The Sun’s absolute magnitude is about in the middle of this range.