14-3 Exploding stars release similar amounts of light and their distance can be inferred by measuring their apparent brightness
A key question that astronomers ask about galaxies is, “How far away are they?” Unfortunately, many of the techniques that are used to measure distances within our Milky Way Galaxy cannot be used for the far greater distances to other galaxies. The extremely accurate parallax method that we described earlier can be used only for stars within about 1500 ly. Beyond that distance, parallax angles become too small to measure.
335
Standard Candles: Variable Stars and Type Ia Supernovae
To determine the distance to a remote galaxy, astronomers look instead for a standard candle—an object, such as a star, that lies within that galaxy and for which we know its luminosity. By measuring how bright the standard candle appears, astronomers can calculate its distance—and hence the distance to the galaxy of which it is part—using the inverse-square law.
The challenge is to find standard candles that are luminous enough to be seen across the tremendous distances to galaxies. To be useful, standard candles should have four properties:
- 1. They should be luminous, so we can see them out to great distances.
- 2. We should be fairly certain about their luminosities, so we can be equally certain of any distance calculated from a standard candle’s apparent brightness and luminosity.
- 3. They should be easily identifiable—for example, by the shape of the light curve of a variable star.
- 4. They should be relatively common so that astronomers can use them to determine the distances to many different galaxies.
For nearby galaxies, Cepheid variable stars make reliable standard candles. These variables can be seen out to about 100 million ly using the Hubble Space Telescope, and their luminosity can be determined from their period through the period-luminosity relation shown in Figure 14-4. Beyond about 100 million ly, even the brightest Cepheid variables, which have luminosities of about 2 × 104 L⊙, fade from view.
One class of standard candles that astronomers have used beyond 100 million ly are Type Ia supernovae. Type Ia supernovae are stars that explode when they capture enough material from a very nearby star to ignite in a tremendous explosion and blow themselves apart. A Type Ia supernova can reach a maximum luminosity of about 3 × 109 L⊙ (Figure 14-12). If a Type Ia supernova is seen in a distant galaxy and its maximum apparent brightness measured, the inverse-square law can be used to find the galaxy’s distance.

One complication is that not all Type Ia supernovae are equally luminous. Fortunately, there is a simple relationship between the peak luminosity of a Type Ia supernova and the rate at which the luminosity decreases after the peak: The more slowly the brightness decreases, the more luminous the supernova. Using this relationship, astronomers have measured distances to supernovae more than 3 billion ly from Earth.
Unfortunately, this technique can be used only for galaxies in which we happen to observe a Type Ia supernova. But telescopic surveys now identify many dozens of these supernovae every year, so the number of galaxies whose distances can be measured in this way is continually increasing.
Question
ConceptCheck 14-6: If a thin cloud of intergalactic dust reduced the observed brightness of a Type Ia supernova found in a distant galaxy, would astronomers who did not know the dust was there mistakenly assume the galaxy is farther or closer than it actually is?
336
Distance Determination Without Standard Candles
 Go to Video 14-2
Go to Video 14-2
Other methods for determining the distances to galaxies do not make use of standard candles. One was discovered in the 1970s by the astronomers Brent Tully and Richard Fisher. They found that the variability in the light emitted by glowing hydrogen in a certain galaxy is related to the galaxy’s overall luminosity. This correlation is the Tully-Fisher relation—the more variability in this particular light emitted by glowing hydrogen, the more luminous the galaxy.
Distances to most galaxies are measured by identifying their brightest stars, such as supernovae.
Such a relationship exists because radiation from the approaching side of a rotating galaxy is changed to shorter wavelengths of light, while that from the galaxy’s receding side is changed to longer wavelengths of light. Thus, the variability in the light is increased by an amount directly related to how fast a galaxy is rotating. Furthermore, astronomers know that the more stars a galaxy contains, the more luminous it is. Consequently, the variability in this particular amount of light emitted by hydrogen is directly related to a galaxy’s luminosity. By combining this information with measurements of apparent brightness, they can calculate the distance to the galaxy. This technique can be used to measure distances of 300 million ly or more.
Question
ConceptCheck 14-7: Is a galaxy that appears to be quite bright but has almost no variability in the hydrogen light emitted relatively close to or very distant from our own Galaxy?
The Distance Ladder
Figure 14-13 shows the ranges of applicability of several important means of determining astronomical distances. Because these ranges overlap, one technique can be used to calibrate another. As an example, astronomers have studied Cepheids in nearby galaxies that have been host to Type Ia supernovae. The Cepheids provide the distances to these nearby galaxies, making it possible to determine the peak luminosity of each supernova using its maximum apparent brightness and the inverse-square law. Once the peak luminosity is known, it can be used to determine the distance to Type Ia supernovae in more distant galaxies. Because one measuring technique leads us to the next one like rungs on a ladder, the techniques shown in Figure 14-13 (along with others) are referred to collectively as the distance ladder.
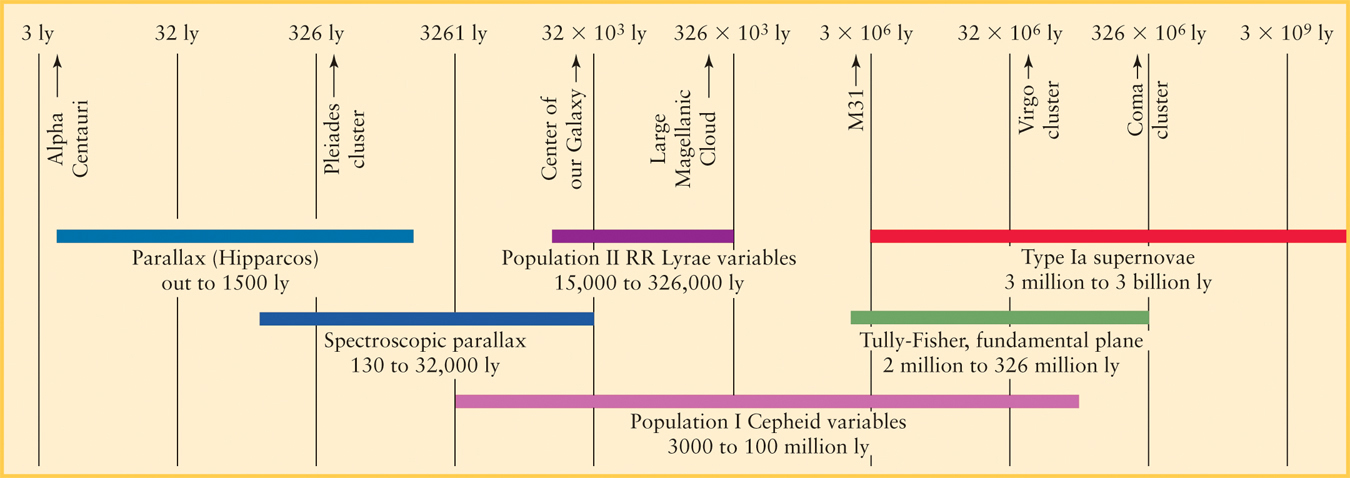
ANALOGY
If you give a slight shake to the bottom of a tall ladder, the top can wobble back and forth alarmingly. A change in distance-measuring techniques used for nearby objects can also have substantial effects on the distances to remote galaxies. For example, if astronomers discovered that the distances to nearby Cepheids were in error, distance measurements using any technique that is calibrated by Cepheids would be affected as well. (As an example, the distance to the galaxy M100 shown in Figure 14-3 is determined using Cepheids. A Type Ia supernova has been seen in M100, as Figure 14-12 shows, and its luminosity is determined using the Cepheid-derived distance to M100. Any change in the calculated distance to M100 would change the calculated luminosity of the Type Ia supernova, and so would have an effect on all distances derived from observations of how bright these supernovae appear in other galaxies.) For this reason, astronomers go to great lengths to check the accuracy and reliability of their standard candles.
Question
ConceptCheck 14-8: Which of the rungs on the distance ladder depend on an accurate measurement of parallax?
337