The 68-95-99.7 Rule

true

Stat Tutor

true

You have completed 0 question sequences out of 4.

Video Player is loading.

Current Time 0:00

Duration 0:00

Loaded: 0%

Stream Type LIVE

Remaining Time -0:00

Chapters

descriptions off, selected

captions settings, opens captions settings dialog
captions off, selected

This is a modal window.

Beginning of dialog window. Escape will cancel and close the window.

TextColorTransparency

BackgroundColorTransparency

WindowColorTransparency

Font Size

Text Edge Style

Font Family

End of dialog window.

This is a modal window. This modal can be closed by pressing the Escape key or activating the close button.

This is a modal window.

Questions 1-3

Question 1.

What percentage of the area of a Normal curve lies within two standard deviations of the mean?

99.7%

68%

95%

Correct. 95% of the area of a Normal curve lies within two standard deviations of the mean. This is an important fact.

Incorrect. 95% of the area of a Normal curve lies within two standard deviations of the mean. This is an important fact.

Try again.

Question 2.

What percent of the area under the above Normal curve is between 55 and 65?

95%

99.7%

68%

Correct. For this Normal distribution, standard deviation is 5. For all Normal curves, 68% of the area is within one standard deviation of the mean, so 68% of the area under the curve is between 55 and 65.

Incorrect. For this Normal distribution, standard deviation is 5. For all Normal curves, 68% of the area is within one standard deviation of the mean, so 68% of the area under the curve is between 55 and 65.

Try again.

Question 3.

What percentage of the area under the above Normal curve is between 45 and 75?

95%

99.7%

68%

Correct. For all Normal density curves, 99.7% of the area under the curve is within three standard deviations of the mean. Since 45 is three standard deviation lengths below the mean and 75 is three standard deviation lengths above the mean, 99.7% of the area under the curve is between 45 and 75.

Incorrect. For all Normal density curves, 99.7% of the area under the curve is within three standard deviations of the mean. Since 45 is three standard deviation lengths below the mean and 75 is three standard deviation lengths above the mean, 99.7% of the area under the curve is between 45 and 75.

Try again.

Questions 4-6

161

Question 4.

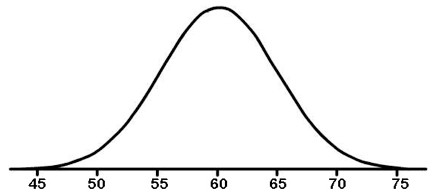
What percentage of the area under the above Normal curve is less than 60?

13.5%

50%

34%

16%

2.35%

Correct. Half of the area of the curve is less than 60 and half is greater than 60.

Incorrect. Half of the area of the curve is less than 60 and half is greater than 60.

Try again.

Question 5.

What percentage of the area curve above Normal curve is between 55 and 60?

13.5%

50%

34%

2.35%

16%

Correct. 68% of the area is between 55 and 65. Half of this area of 68% is 34%.

Incorrect. 68% of the area is between 55 and 65. Half of this area of 68% is 34%.

Try again.

Question 6.

What percentage of the area under above Normal curve is less than 55?

34%

2.35%

50%

13.5%

16%

Correct. Half of the curve is 50%. 50% minus 34% is 16%. Or you could add 0.15% + 2.35% + 13.5% = 16%

Incorrect. Half of the curve is 50%. 50% minus 34% is 16%. Or you could add 0.15% + 2.35% + 13.5% = 16%

Try again.

Questions 7-8

201

Question 7.

What percentage of the area under above Normal curve is less than 50?

2.5%

97.5%

50%

84%

34%

16%

Correct. 95% of the area under any Normal curve is within two standard deviations of the mean. That means 100% – 95% = 5% is the area less than 50 and greater than 70. Half of this is the area less than 50. So, 5%/2 = 2.5%.

Incorrect. 95% of the area under any Normal curve is within two standard deviations of the mean. That means 100% – 95% = 5% is the area less than 50 and greater than 70. Half of this is the area less than 50. So, 5%/2 = 2.5%.

Try again.

Question 8.

What percentage of the area under above Normal curve is less than 65?

50%

97.5%

16%

34%

84%

2.5%

Correct. 50% of the curve is less than 60. 68% is between 55 and 65. Half of 68% or 34% is between 60 and 65. 50% + 34% = 84%

Incorrect. 50% of the curve is less than 60. 68% is between 55 and 65. Half of 68% or 34% is between 60 and 65. 50% + 34% = 84%

Try again.

Questions 9-11

287

Question 9.

What percentage of the area under above Normal curve is between 50 and 55?

15.85%

13.5%

81.5%

47.5%

Correct. First, find the area under the curve less than 50. 100% – 95% = 5%. And half of 5% is 2.5%. Second, find the area under the curve less than 55. 100% – 68% = 34%. Half of 34% is 16%. The area under the curve between 50 and 55 equals 16% minus 2.5% = 13.5%.

Incorrect. First, find the area under the curve less than 50. 100% – 95% = 5%. And half of 5% is 2.5%. Second, find the area under the curve less than 55. 100% – 68% = 34%. Half of 34% is 16%. The area under the curve between 50 and 55 equals 16% minus 2.5% = 13.5%.

Try again.

Question 10.

What percentage of the area under above Normal curve is between 55 and 70?

81.5%

13.5%

47.5%

15.85%

Correct. The area between 55 and 65 is 68%; the area between 65 and 70 is 13.5%: 68% + 13.5% = 81.5%. If you want to know how to get the area between 65 and 70, first, find the area under the curve greater than 70. 100% – 95% = 5%. And half of 5% is 2.5%. Second, find the area under the curve greater than 65. 100% – 68% = 34%. Half of 34% is 16%. Thus, the area under the curve between 65 and 70 equals 16% minus 2.5% = 13.5%.

Incorrect. The area between 55 and 65 is 68%; the area between 65 and 70 is 13.5%: 68% + 13.5% = 81.5%. If you want to know how to get the area between 65 and 70, first, find the area under the curve greater than 70. 100% – 95% = 5%. And half of 5% is 2.5%. Second, find the area under the curve greater than 65. 100% – 68% = 34%. Half of 34% is 16%. Thus, the area under the curve between 65 and 70 equals 16% minus 2.5% = 13.5%.

Try again.

Question 11.

Math SAT scores are Normally distributed with mean µ = 500 and standard deviation σ = 100. The curve is displayed above. What percentage of the SAT Math scores are less than one standard deviation above the mean?

97.5%

2.5%

16%

34%

50%

84%

Correct. You actually don’t need to know the values of μ and σ to answer this question. (Note that 400 is one standard deviation below the mean.) The area under the curve within one standard deviation of the mean is 68%. Half of this is 34%. Then note that 50% of the area under the curve is less than the mean. The area less than one standard deviation below the mean is 50% minus 34% = 16%.

Incorrect. You actually don’t need to know the values of μ and σ to answer this question. (Note that 400 is one standard deviation below the mean.) The area under the curve within one standard deviation of the mean is 68%. Half of this is 34%. Then note that 50% of the area under the curve is less than the mean. The area less than one standard deviation below the mean is 50% minus 34% = 16%.

Try again.

StatTutor Lesson - The 68-95-99.7 Rule

Questions 1-3

Question 1.

Question 2.

Question 3.

Questions 4-6

Question 4.

Question 5.

Question 6.

Questions 7-8

Question 7.

Question 8.

Questions 9-11

Question 9.

Question 10.

Question 11.