StatTutor Lesson - Probability Rules
true
Stat Tutor
true
true
You have completed 0 question sequences out of 3.
Questions 1-2
89
Question 1.
True or False: When drawing a card from a standard deck, events A = {face card} and B = {heart} are disjoint.
| A. |
| B. |
Correct. These events are not disjoint. The Jack, Queen, and King of hearts are both face cards and hearts.
Incorrect. These events are not disjoint. The Jack, Queen, and King of hearts are both face cards and hearts.
2
Try again.
Questions 3-5
141
Question 3.
We are interested in looking at apartment complex amenities when deciding where to live next year. Let P mean a complex has a pool, C mean the complex has a clubhouse, and I mean high speed Internet. If a complex has all three (and would then be high on our list of possible places to live), the notation for this event would be
| A. |
| B. |
| C. |
Correct. Events P and C and I all occurring at the same time (in the same complex) would be P and C and I.
Incorrect. Events P and C and I all occurring at the same time (in the same complex) would be P and C and I.
2
Try again.
Questions 6-8
355
Question 6.

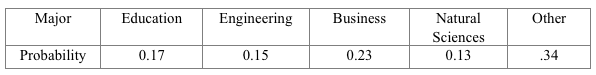
Consider the probability distribution of majors at one university as given above. What is the probability a randomly selected student has an “Other” major?
Correct. The probability of the entire sample space must be 1, and these options cover all possible majors. Subtract the total of the given probabilities from 1 to find P(Other).
Incorrect. The probability of the entire sample space must be 1, and these options cover all possible majors. Subtract the total of the given probabilities from 1 to find P(Other).
2
Try again.